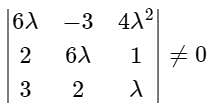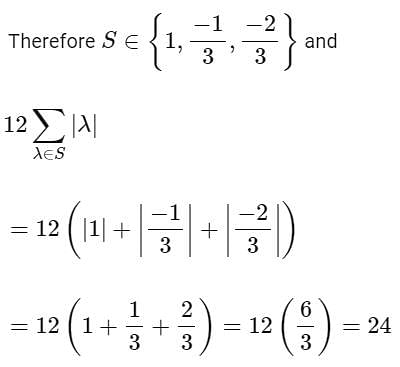JEE Main Mock Test - 3 - JEE MCQ
30 Questions MCQ Test - JEE Main Mock Test - 3
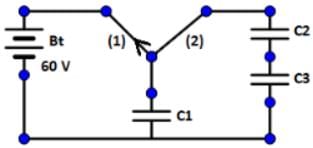
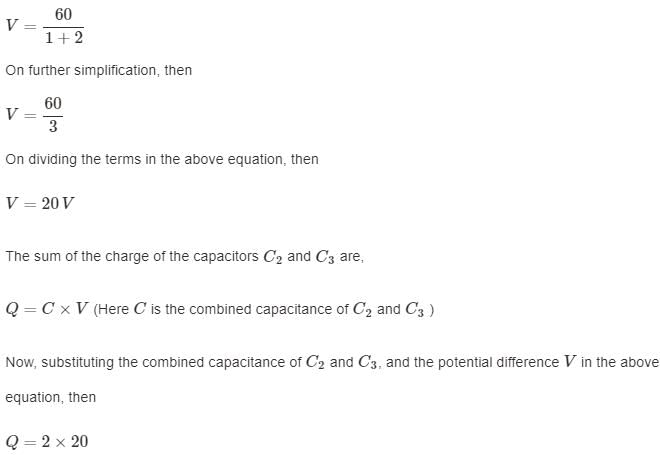
A capacitor C1 = 1.0 μF is charged up to a voltage V = 60 V by connecting it to battery B through switch (1). Now, C1 is disconnected from battery and connected to a circuit consisting of two uncharged capacitors, C2 = 3.0 μF and C3 = 6.0 μ F through switch (2), as shown in the figure. The sum of final charges on C2 and C3 is:

The length, breadth and thickness of a rectangular sheet of metal are 4.234 m, 1.005 m, and 2.01 cm respectively. Give the volume of the sheet to correct significant figures.
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Population of a town is reported as 157,900 . Which of the following statements correct?
The product of energy and time is called action. The dimensional formula for action is same as that for
The Van der Waal equation for 1 mole of a real gas is
where P is the pressure, V is the volume, T is the absolute temperature, R is the molar gas constant and a, b are Van dar Waal constants. The dimensions of a are the same as those of
Force F is given in terms of time t and distance x by
F = A sin C t + B cos D x
Then the dimensions of and
are given by
If force (F) is given by F = Pt_1 + Qt, where t is time. The unit of P is same as that of
When a wave traverses a medium, the displacement of a particle located at x at time t is given by y = a sin (bt - cx) where a, b and c are constants of the wave. The dimensions of b/c are the same as those of
Which of the following sets cannot enter into the list of fundamental quantities in any system of units ?
What is the physical quantity whose dimensions are M L2 T-2 ?
Which pair of following quantities has dimensions different from each other
A solid ball of radius R has a charge density ρ given by 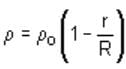 for 0 ≤ r ≤ R. The electric field outside the ball is:
for 0 ≤ r ≤ R. The electric field outside the ball is:
A heating element has a resistance of 100 Ω at room temperature. When it is connected to a supply of 220 V, a steady current of 2 A passes in it and temperature is 500°C more than room temperature. What is the temperature coefficient of resistance of the heating element?
An observer is moving with half the speed of light towards a stationary microwave source emitting waves at frequency 10 GHz. What is the frequency of the microwave measured by the observer?
(Speed of light = 3 × 108 ms–1)
An external pressure P is applied on a cube at 0°C so that it is equally compressed from all sides. K is the bulk modulus of the material of the cube and α is its coefficient of linear expansion. Suppose we want to bring the cube to its original size by heating. The temperature should be raised by:
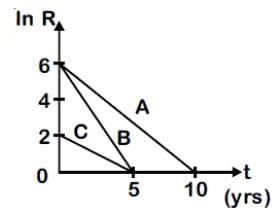
Activities of three radioactive substances A, B and C are represented by the curves A, B and C, in the figure. Then their half-lives T1/2 (A) : T1/2 (B) : T1/2 (C) are in the ratio
The ratio of mass densities of nuclei of 40Ca and 16O is close to
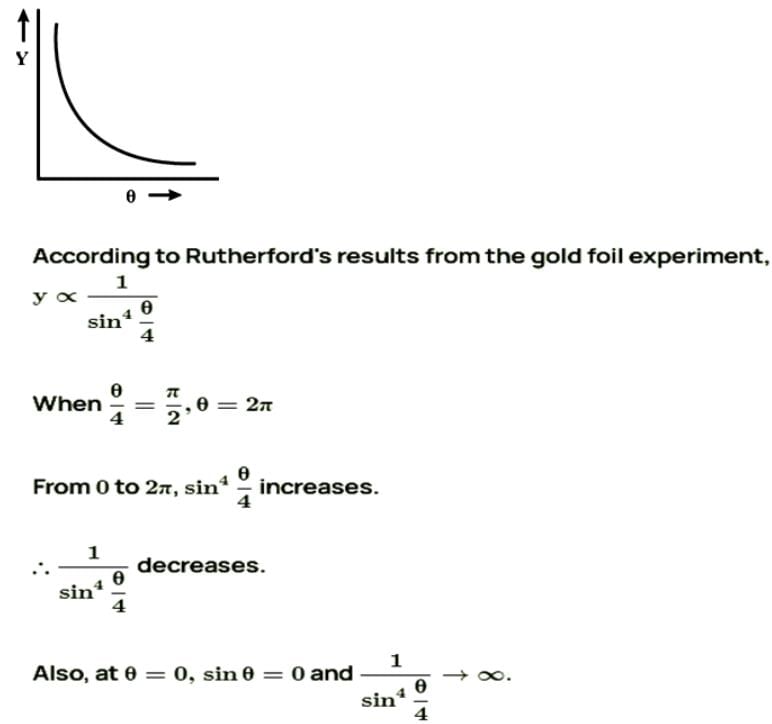
The graph which depicts the results of Rutherford gold foil experiment with α-particles is:
θ: Scattering angle
Y: Number of scattered α-particles detected
(Plots are schematic and not to scale)
A hydrogen atom, initially in the ground state is excited by absorbing a photon of wavelength 980Å. The radius of the atom in the excited state, will be _____a0.
(hc = 12500 eV - Å)
A rigid diatomic ideal gas undergoes an adiabatic process at room temperature. The relation between temperature and volume of this process is TVk/5 = constant, then value of k is ______.
The gas mixture constists of 3 moles of oxygen and 5 moles of argon at temperature T. Considering only translational and rotational modes, the total inernal energy of the system is _______RT.
A refrigerator is to maintain eatables kept inside at 9oC. If room temperature is 36oC, calculate the coefficient of performance.
Let 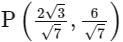 ,Q,R and S be four points on the ellipse 9x2 + 4y2 = 36. Let PQ and RS be mutually perpendicular and pass through the origin. If
,Q,R and S be four points on the ellipse 9x2 + 4y2 = 36. Let PQ and RS be mutually perpendicular and pass through the origin. If 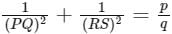 , where p and q are coprime, then p + q is equal to :
, where p and q are coprime, then p + q is equal to :
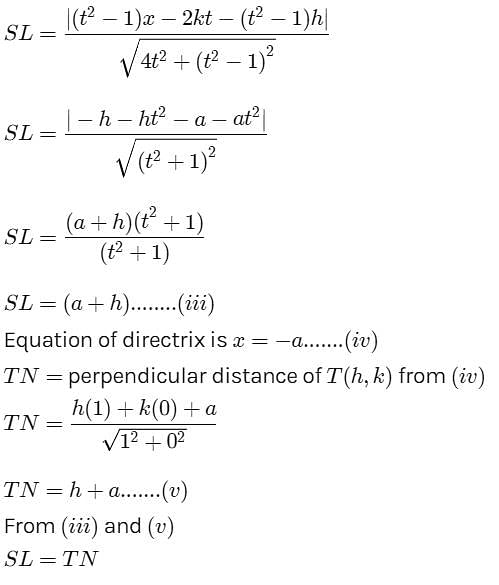
T is a point on the tangent to a parabola y2 = 4ax at its point P. TL and TN are the perpendiculars on the focal radius SP and the directrix of the parabola respectively. Then
A bag contains six balls of different colours. Two balls are drawn in succession with replacement. The probability that both the balls are of the same colour is p. Next four balls are drawn in succession with replacement and the probability that exactly three balls are of the same colour is q. If p: q = m : n, where m and n are coprime, then m + n is equal to:
If the mean and variance of four numbers 3, 7, x and y(x > y) be 5 and 10 respectively, then the mean of four numbers 3 + 2x, 7 + 2y, x + y and x - y is ______.
Let S be the set of values of λ, for which the system of equations
6λx - 3y + 3z = 4λ2,
2x - 6λy + 4z = 1,
3x + 2y + 3λz = λ has no solution. Then 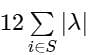 is equal to ___________.
is equal to ___________.




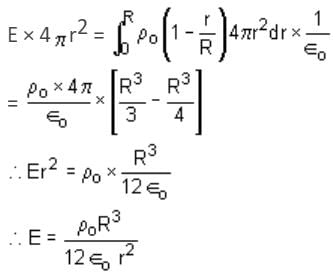
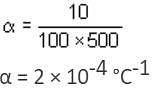
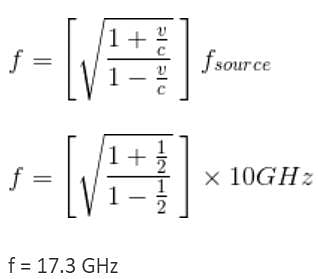
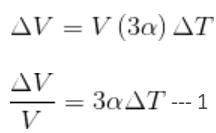
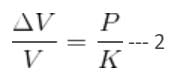

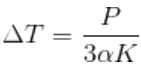

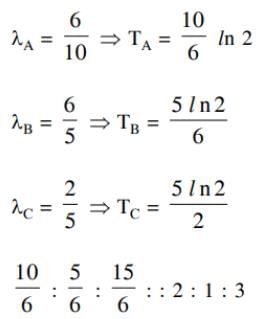
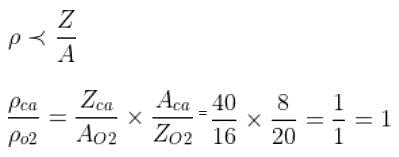
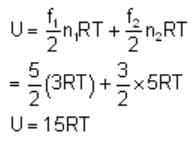
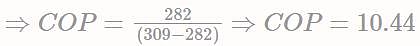
 is equal to
is equal to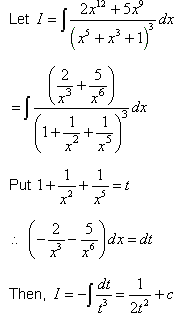
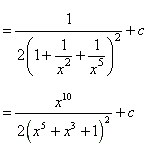
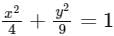 . This is the standard form of the equation of an ellipse centered at the origin, with semi-major axis a = 3 along the y-axis and semi-minor axis b = 2 along the x-axis.
. This is the standard form of the equation of an ellipse centered at the origin, with semi-major axis a = 3 along the y-axis and semi-minor axis b = 2 along the x-axis.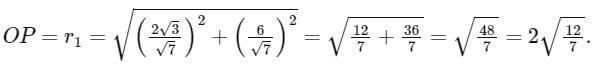
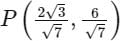 in polar coordinates. We can write
in polar coordinates. We can write 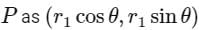 .
. 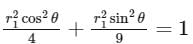
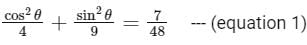
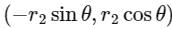 , (the negative sign is due to the fact that line RS is perpendicular to line PQ. Since they are perpendicular, the angle between them is 90 degrees or π/2 radians. In terms of sin and
, (the negative sign is due to the fact that line RS is perpendicular to line PQ. Since they are perpendicular, the angle between them is 90 degrees or π/2 radians. In terms of sin and  .
. 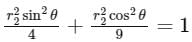
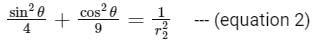
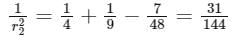
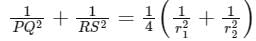
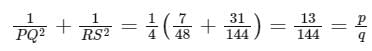
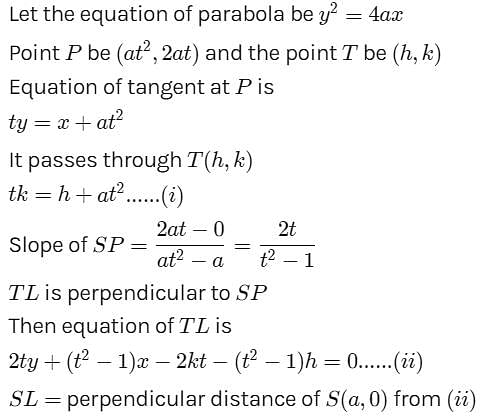
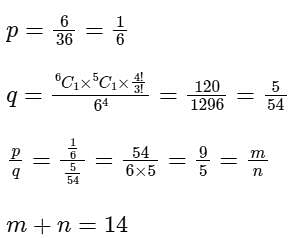
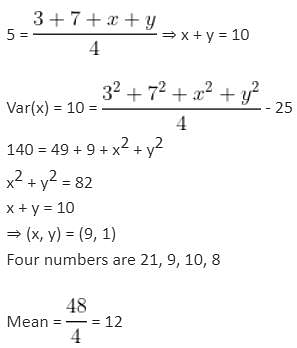
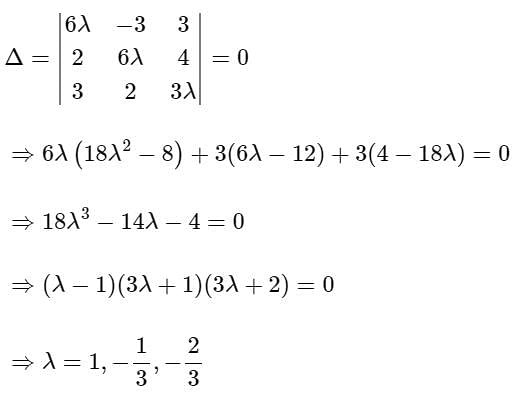
 we have
we have