CTET & State TET Exam > CTET & State TET Tests > Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - CTET & State TET MCQ
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - CTET & State TET MCQ
Test Description
10 Questions MCQ Test - Maths Pedagogy Paper 2 (Language, Place and Community Mathematics)
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) for CTET & State TET 2024 is part of CTET & State TET preparation. The Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) questions and answers have been prepared
according to the CTET & State TET exam syllabus.The Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) MCQs are made for CTET & State TET 2024 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) below.
Solutions of Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) questions in English are available as part of our course for CTET & State TET & Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) solutions in
Hindi for CTET & State TET course.
Download more important topics, notes, lectures and mock test series for CTET & State TET Exam by signing up for free. Attempt Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) | 10 questions in 10 minutes | Mock test for CTET & State TET preparation | Free important questions MCQ to study for CTET & State TET Exam | Download free PDF with solutions
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 1
What is the reason for a fear of the subject-math phobia?
A) Presence of a number of symbols and formulae.
B) Differences based on age and gender.
C) The gap between explanation by teacher transmitted and received by students.
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 1
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 2
According to many research studies, which of the following is the most appropriate reason for gender based differences in mathematical achievement in school mathematics education.
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 3
Translating verbal language to the language of mathematics, that is solving a word problem, involves three stages: (i) encoding, (ii) operations, (iii) decoding. Which one of the following examples best describes the encoding process?
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 3
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 4
Consider the following statements:
(i) Accuracy and speed cannot go together
(ii) Accuracy and speed can go together
(iii) Accuracy and speed must be developed separately
Which of the statements given above is/are true?
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 4
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 5
Language of mathematics is symbolic in nature. Here, the term 'symbolic' refers to:
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 5
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 6
Which of the following is NOT a correct strategy for a teacher to address gender stereotyping in a middle school classroom?
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 6
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 7
If the language of mathematics reveals imperfect meanings then
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 7
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 8
Which of the following personality characteristics could not be developed through 'study of Mathematics' ?
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 8
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 9
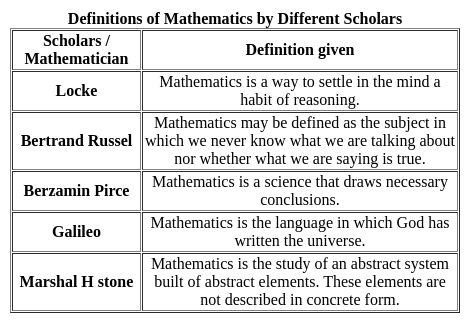
Who was the great scientist who appreciated the language of mathematics as - "Mathematics is the language in which God created the universe".
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 9
Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 10
Language of mathematics learned in school should be interlinked with everyday speech because
Detailed Solution for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) - Question 10
Information about Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) Page
In this test you can find the Exam questions for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics) solved & explained in the simplest way possible.
Besides giving Questions and answers for Maths Pedagogy Paper 2 (Language, Place and Community Mathematics), EduRev gives you an ample number of Online tests for practice
Download as PDF