CTET & State TET Exam > CTET & State TET Tests > Mathematics Paper 2 (Algebra - I) - CTET & State TET MCQ
Mathematics Paper 2 (Algebra - I) - CTET & State TET MCQ
Test Description
10 Questions MCQ Test - Mathematics Paper 2 (Algebra - I)
Mathematics Paper 2 (Algebra - I) for CTET & State TET 2024 is part of CTET & State TET preparation. The Mathematics Paper 2 (Algebra - I) questions and answers have been prepared
according to the CTET & State TET exam syllabus.The Mathematics Paper 2 (Algebra - I) MCQs are made for CTET & State TET 2024 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Mathematics Paper 2 (Algebra - I) below.
Solutions of Mathematics Paper 2 (Algebra - I) questions in English are available as part of our course for CTET & State TET & Mathematics Paper 2 (Algebra - I) solutions in
Hindi for CTET & State TET course.
Download more important topics, notes, lectures and mock test series for CTET & State TET Exam by signing up for free. Attempt Mathematics Paper 2 (Algebra - I) | 10 questions in 10 minutes | Mock test for CTET & State TET preparation | Free important questions MCQ to study for CTET & State TET Exam | Download free PDF with solutions
Mathematics Paper 2 (Algebra - I) - Question 1
What is the value of (27x3 - 58x2y + 31xy2 - 8y3), when x = -5 and y = -7?
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 1
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 2
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 3
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 4
Mathematics Paper 2 (Algebra - I) - Question 5
If (p - q) = 8, then what is the value of q3 - p3 + 24pq?
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 5
Mathematics Paper 2 (Algebra - I) - Question 6
The square of the difference between two given natural numbers is 324, while the product of these two given numbers is 144. Find the positive difference between the squares of these two given numbers.
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 6
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 7
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 8
Mathematics Paper 2 (Algebra - I) - Question 9
Simplify the expression  provided (s + t + u) ≠ 0.
provided (s + t + u) ≠ 0.
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 9
Mathematics Paper 2 (Algebra - I) - Question 10
If (d + e + f) = 14, (d2 + e2 + f2) = 96, then find the value of (de + ef + fd).
Detailed Solution for Mathematics Paper 2 (Algebra - I) - Question 10
Information about Mathematics Paper 2 (Algebra - I) Page
In this test you can find the Exam questions for Mathematics Paper 2 (Algebra - I) solved & explained in the simplest way possible.
Besides giving Questions and answers for Mathematics Paper 2 (Algebra - I), EduRev gives you an ample number of Online tests for practice
Download as PDF


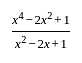
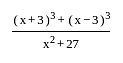
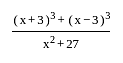

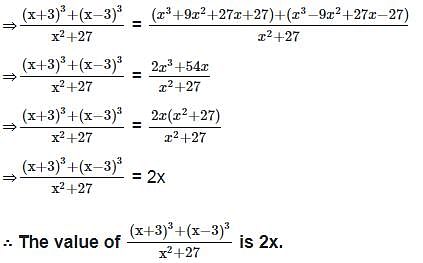
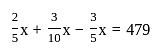
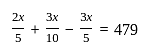
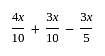 = 479
= 479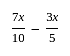 = 479
= 479 = 479
= 479