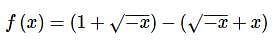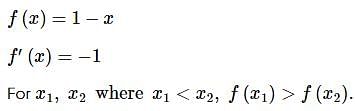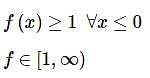AP EAMCET Mock Test - 7 - JEE MCQ
30 Questions MCQ Test - AP EAMCET Mock Test - 7
The inductance of a closed-packed coil of 400 turns is 8 mH. A current of 5 mA is passed through it. The magnetic flux through each turn of the coil is
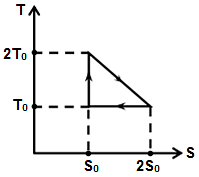
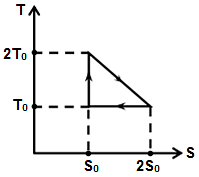
The temperature-entropy diagram of a reversible engine cycle is given in the figure. Its efficiency is

| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
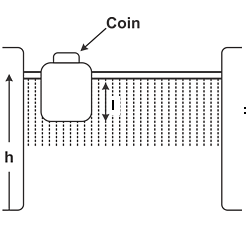
A wooden block, with a coin placed on its top, floats in water as shown in the figure. The distance h and l are shown there. After sometime, the coin falls into the water. Then,
 :
:
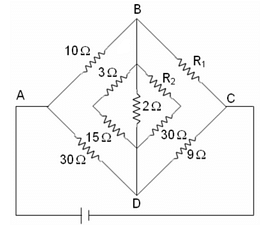
In the Wheatstone's bridge shown below, in order to balance the bridge, we must have
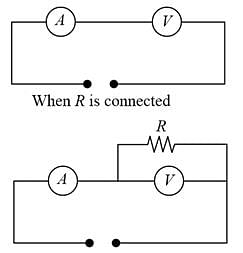
An ammeter and a voltmeter of resistance R are connected in series to an electric cell of negligible internal resistance. Their readings are A and V, respectively. If another resistance R is connected in parallel with the voltmeter, then
A variable force, given by the 2-dimensional vector 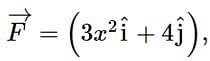 acts on a particle. The force is in newton and x is in metre. What is the change in the kinetic energy of the particle as it moves from the point with coordinates (2, 3) to (3, 0)? (The coordinates are in metres)
acts on a particle. The force is in newton and x is in metre. What is the change in the kinetic energy of the particle as it moves from the point with coordinates (2, 3) to (3, 0)? (The coordinates are in metres)
In the following reaction, which choice has value twice that of the equivalent mass of the oxidising agent SO₂ + H₂O → 3S + 2H₂O
Given the bond energies of N ≡ N , H − H and N − H bonds as 941.5, 433 and 391 kJ mole-1 respectively, the enthalpy of reaction N2 g + 3 H2 g → 2 NH3 g is
The interionic distance for cesium chloride cystal will be
For an ideal gas, the relation between the enthalpy change and internal energy change at constant temperature is given by
Which of the following shows maximum no. of oxidation states?
The sum of n terms of the series 1 + (1 + 3) + (1 + 3 + 9) + (1 + 3 + 9 + 27) + ...... is
If M denotes the mid-point of the line joining 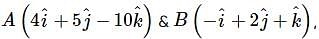 then the equation of the plane through M & perpendicular to AB is
then the equation of the plane through M & perpendicular to AB is
Let lx+my+n=0 represents a chord of the ellipse b2x2+a2y2=a2b2, If the eccentric angles of end points of the chord differ by 90°, then
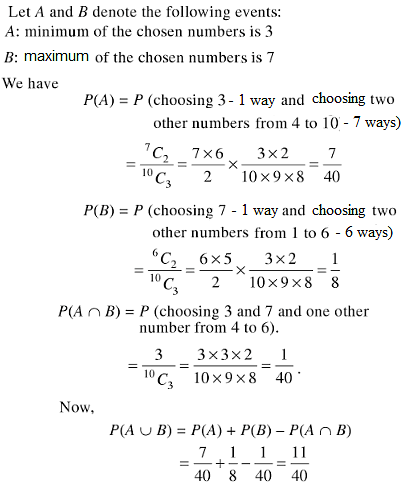
Three numbers from {1, 2, 3, … 10} are chosen at random without replacement. The probability that the minimum of the chosen numbers is 3 or the maximum of the chosen numbers is 7 is
The function f:R→R satisfies 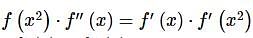 for all real x. Given that f(1)=1 and f′′(1)=8, compute the value of f′(1)+f′′(1).
for all real x. Given that f(1)=1 and f′′(1)=8, compute the value of f′(1)+f′′(1).
All the real values of m such that both roots of the equation x2 − 2mx + m2 − 1 = 0 are greater than −2 and less than 4 lies in
Nitika chose 12 numbers randomly. The sum of 12 numbers is 18 and the sum of their square is 30. What is the variance of the series?
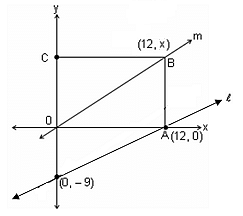
In the given figure, OABC is a rectangle. If || m, what is the area of the rectangle OABC?
If the roots of the equation x2 - bx + c = 0 are two consecutive integers, then b2 - 4c is equal to
If P represents z=x+iy in the argand plane 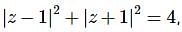 then locus of P is
then locus of P is 


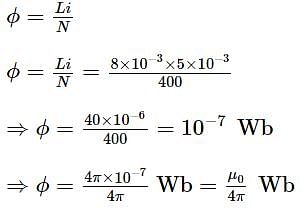

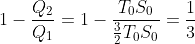
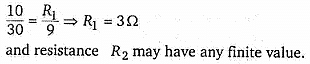
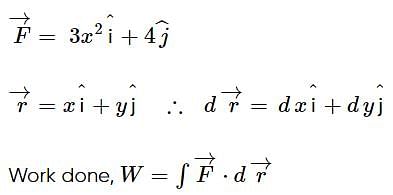
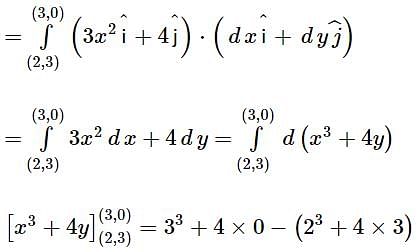



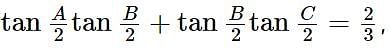 then a + c is equal to
then a + c is equal to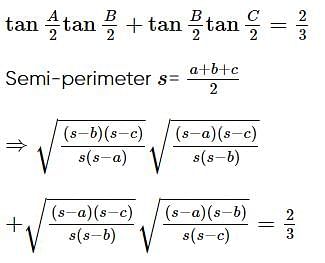
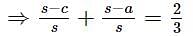
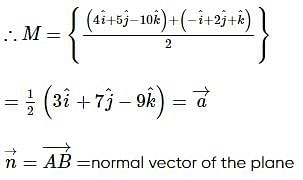
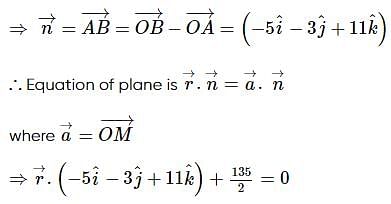
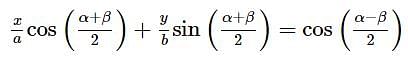
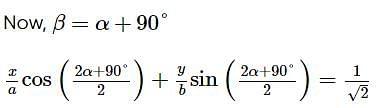
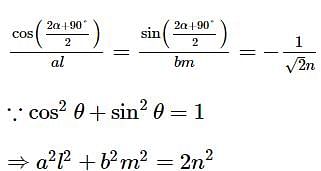
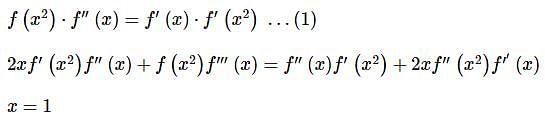
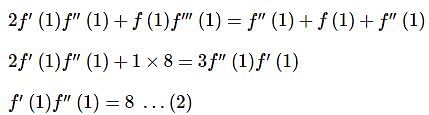
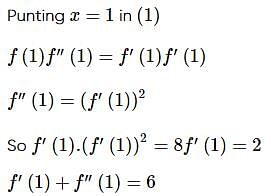
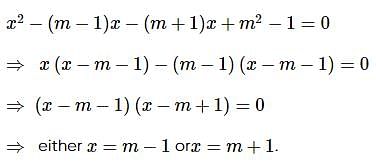


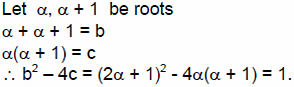
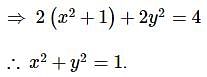
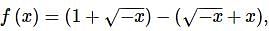 then f(x) is
then f(x) is