Test: Progression (AP And GP)- 4 - CAT MCQ
15 Questions MCQ Test - Test: Progression (AP And GP)- 4
How many terms are there in the AP 20, 25, 30,… 130.
Find the 1st term of an AP whose 8th and 12th terms are respectively 39 and 59.
There is an AP 1, 3, 5…. Which term of this AP is 55?
Find the lowest number in an AP such that the sum of all the terms is 105 and greatest term is 6 times the least.
A sum of money kept in a bank amounts to ` 1240 in 4 years and ` 1600 in 10 years at simpleInterest. Find the sum.
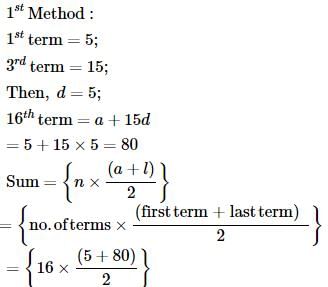
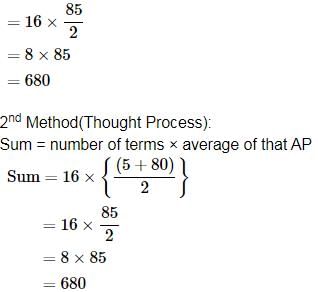
The sum of the first 16 terms of an AP whose first term and third term are 5 and 15 respectively is
The least value of n for which the sum of the series 5 + 8 + 11… n terms is not less than 670 is
How many terms are there in the GP 5, 20, 80, 320,… 20480?
If the fifth term of a GP is 81 and first term is 16, what will be the 4th term of the GP?
The sum of three numbers in a GP is 14 and the sum of their squares is 84. Find the largestnumber.
How many natural numbers between 300 to 500 are multiples of 7?
If a man saves ` 4 more each year than he did the year before and if he saves ` 20 in the first year,after how many years will his savings be more than ` 1000 altogether?
The 4th and 10th term of an GP are 1/3 and 243 respectively. Find the 2nd term.
The sum of 5 numbers in AP is 30 and the sum of their squares is 220. Which of the following is the third term?
The sum of the first four terms of an AP is 28 and sum of the first eight terms of the same AP is 88.Find the sum of the first 16 terms of the AP?