Test: Graphical Solutions - JEE MCQ
20 Questions MCQ Test - Test: Graphical Solutions
Find the pairs of consecutive even positive integers both of which are smaller than 10 and their sum of more than 11
For a student to qualify for a certain course, the average of his marks in the permitted 3 attempts must be more than 60. His first two attempts yielded only 45 and 62 marks respectively. What is the minimum score required in the third attempt to qualify?
Which one of them is the solution for x, when x is integer and 12 x > 30?
Find the value of x which satisfies 5x – 3 < 7, where x is a natural number.
The inequations -4x+1≥0 and 3-4x<0 have the common solutions given by
A point P lies in the solution region of 3x – 7 > x + 3. So the possible coordinates of P are
A connected planar graph having 6 vertices, 7 edges contains _____________ regions.
The solution of inequality 4x + 3 < 5x + 7 when x is a real number is
Two less than 5 times a number is greater than the third multiple of the number. So the number must be
Find the value of x when x is a natural number and 24x< 100.



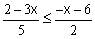
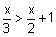 , where x is a real
, where x is a real










