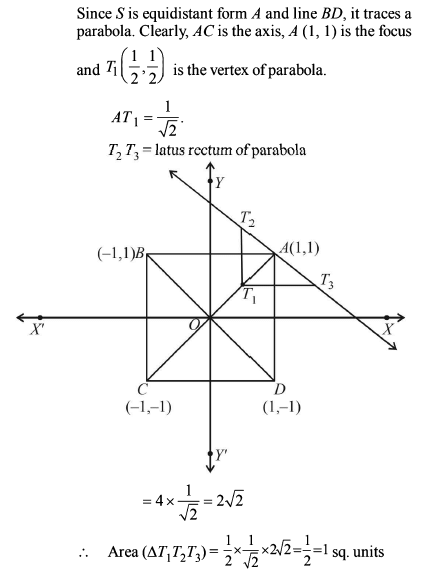
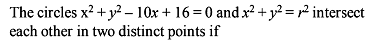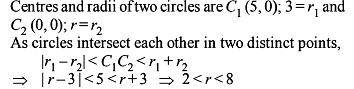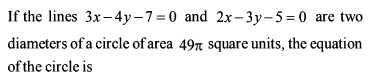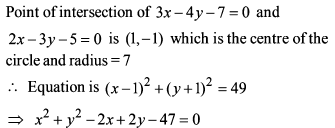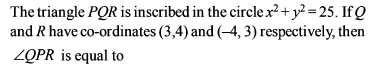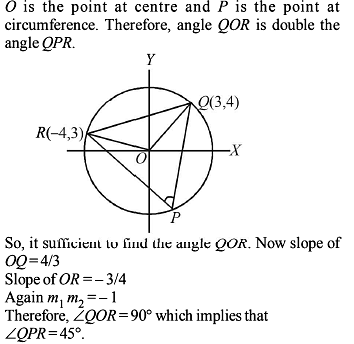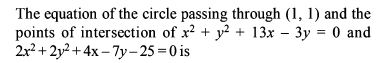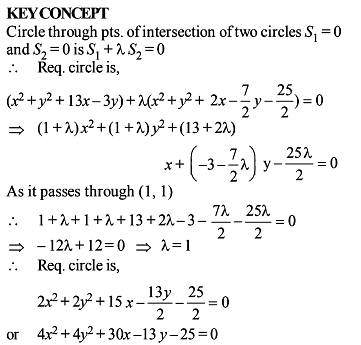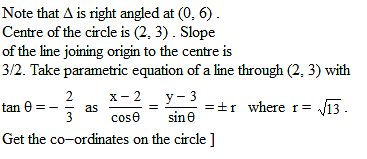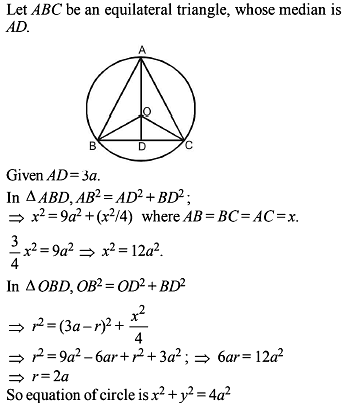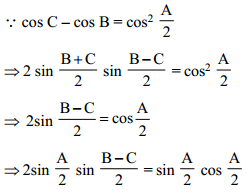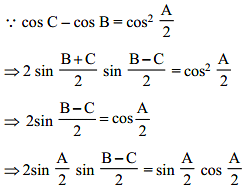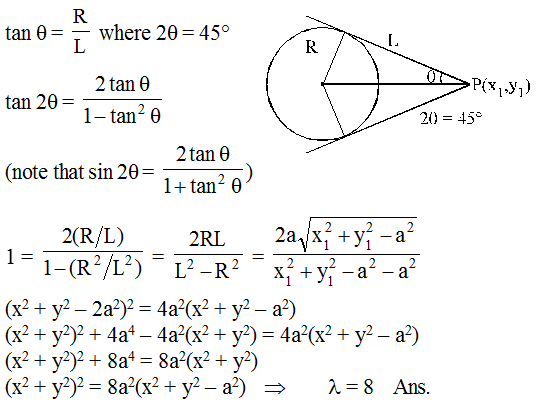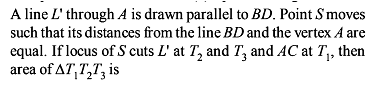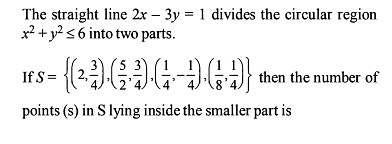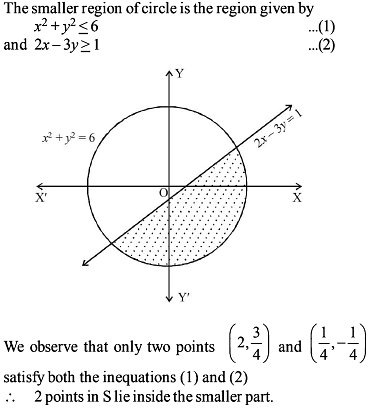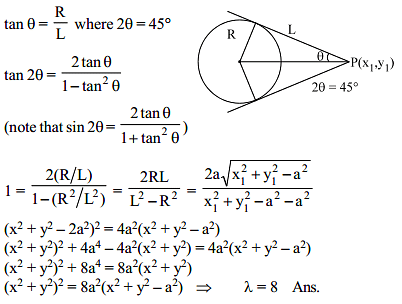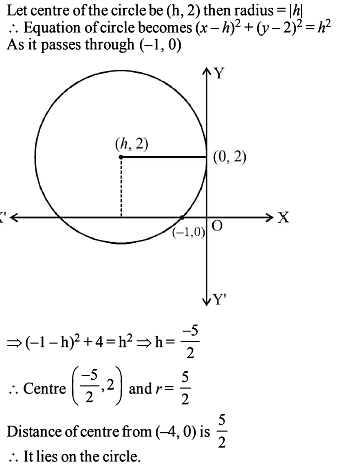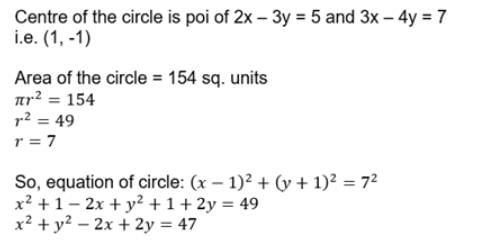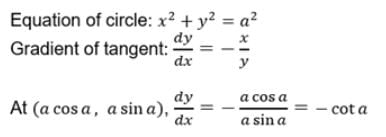Circle - 1 - JEE MCQ
30 Questions MCQ Test - Circle - 1
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
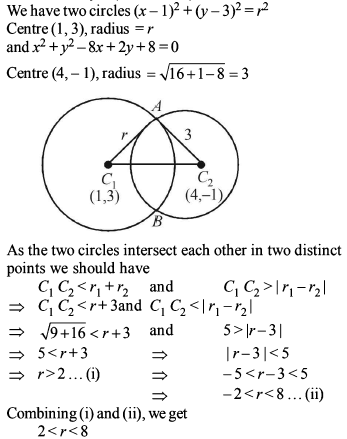
The possible radius of a circle whose centre is at the origin and which touches the circle x2 + y2 – 6x – 8y + 21 = 0, is
A circle passes through the points (- 1, 1) , (0, 6) and (5, 5) . The point(s) on this circle, the tangent(s) at which is/are parallel to the straight line joining the origin to its centre is/are :
In a triangle ABC with fixed base BC, the vertex A moves such that cos C – cos B = cos2 A/2 . If a, b and c denote the lengths of sides of the triangle opposite to angles A, B and C, respectively, then which one of the following is correct?
The locus of the point of intersection of the tangent to the circle x2 + y2 = a2, which include an angle of 45° is the curve (x2 + y2)2 = λa2 (x2 + y2 – a2). The value of λ is
The locus of the point of intersection of the tangent to the circle x2 + y2 = a2, which include an angle of 45° is the curve
(x2 + y2)2 = λa2 (x2 + y2 – a2). The value of λ is
The possible radius of a circle whose centre is at the origin and which touches the circle x2 + y2 – 6x – 8y + 21 = 0, is
The lines 2x – 3y = 5 and 3x – 4y = 7 are diameters of a circle of area 154 sq. units. The equation of the circle is
The equation of the circle passing through (3, 6) and whose centre is (2, –1) is
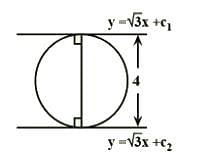
y = √3x + c1 & y = √3x + c2 are two parallel tangents of a circle of radius 2 units, then |c1 – c2| is equal to
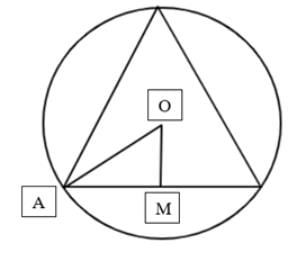
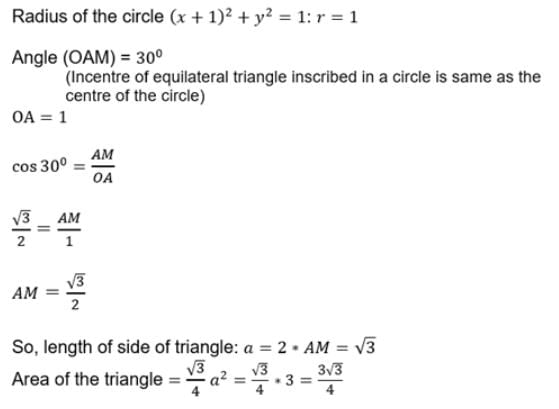
The area of an equilateral triangle inscribed in the circle x2 + y2 – 2x = 0 is
The gradient of the tangent line at the point (a cos a, a sin a) to the circle x2 + y2 = a2, is
The greatest distance of the point P(10, 7) from the circle x2 + y2 – 4x – 2y – 20 = 0 is