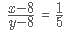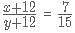Test: CAT Quantitative Aptitude- 1 (October 7) - CAT MCQ
10 Questions MCQ Test - Test: CAT Quantitative Aptitude- 1 (October 7)
Arun, Barun, Chandan and Diksha have books in the ratio 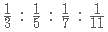 respectively. What is the the minimum number of books they must be having altogether?
respectively. What is the the minimum number of books they must be having altogether?
(Enter ‘0’ as the answer if the answer cannot be determined.)
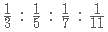 respectively. What is the the minimum number of books they must be having altogether?
respectively. What is the the minimum number of books they must be having altogether?(Enter ‘0’ as the answer if the answer cannot be determined.)
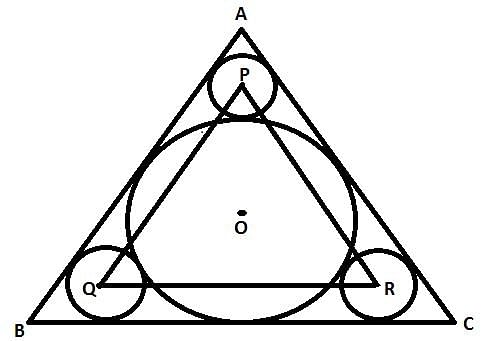
A circle with radius 6 cm is inscribed inside an equilateral triangle ABC. Three smaller circle are drawn touching the incircle and the sides of ABC as shown in the figure. Another triangle is formed by joining centres P,Q and R of these smaller circles. What is the perimeter of triangle PQR?

| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
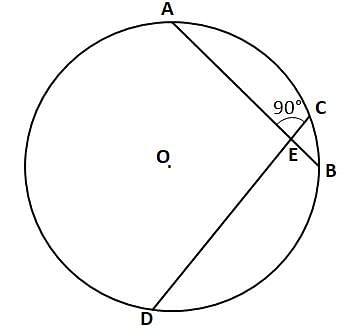
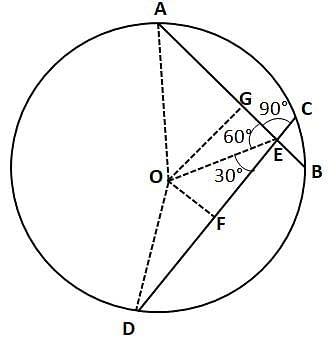
In a circle, two chords AB and CD intersect at a point E as shown in the figure. If AB = 6 cm and CD = 10 cm and ∠ OED = 30°, then find out the radius of the given circle.

The minimum value of the expression |x+3|+|x-3|+|x-6|+|x-5|+|x+5|
Which condition will a,b,c satisfy if the set of equations 3x + 4y + z = a, 2x + 6y + 4z = b and x - y - 2z = c has atleast 1 solution ? Also a + b + c ≠ 0.
DIRECTIONS for the question: Solve the following question and mark the best possible option.
A person can complete a job in 120 days. He works alone on Day 1. On Day 2, he is joined by another person who also can complete the job in exactly 120 days. On Day 3, they are joined by another person of equal efficiency. Like this, everyday a new person with the same efficiency joins the work. How many days are required to complete the job?
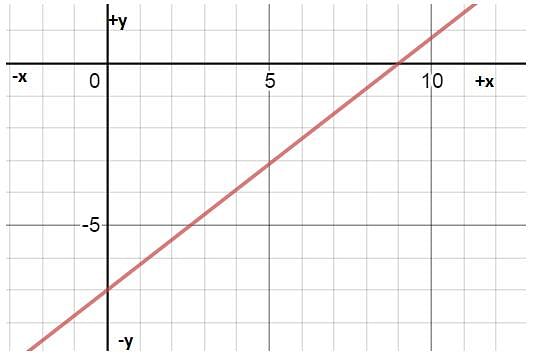
How many points in the region enclosed by x > 0, y < 0 and 7x - 9y < 63 have integral coordinates?
How many scalene triangles with integral sides can be formed with a perimeter of 45 cm?
If a, b, c, and d are integers such that a + b + c + d = 30, then the minimum possible value of
(a - b)2 + (a - c)2 + (a - d)2 is
Eight years ago, the ratio of ages of Akhil and Akash was 1:5. 12 years from now, the ratio changes to 7 : 15. Find the sum of the antecedent and the consequent of the ratio of their present ages, when the ratio is in its lowest form.


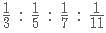
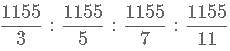 which is equivalent to
which is equivalent to 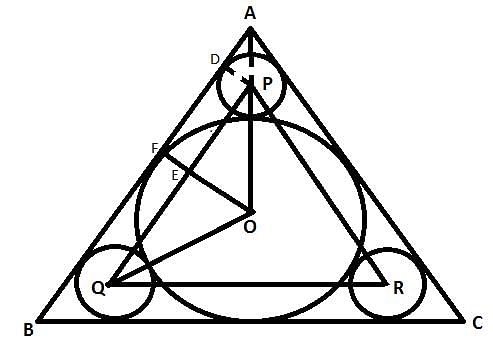
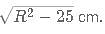
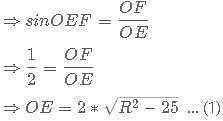
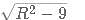
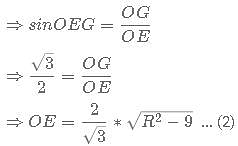
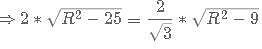
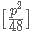 when p is even and
when p is even and 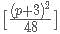 when p is odd, where [] is the nearest integer function.
when p is odd, where [] is the nearest integer function.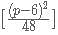 when p is even and
when p is even and 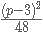 if p is odd.
if p is odd.