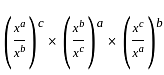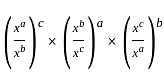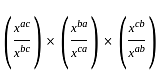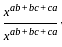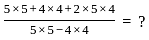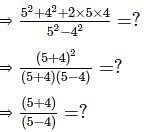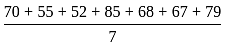RRB NTPC Mathematics Test - 1 - RRB NTPC/ASM/CA/TA MCQ
30 Questions MCQ Test - RRB NTPC Mathematics Test - 1
The income of A and B are in the ratio 5 ∶ 7 and their expenditure is in the ratio 1 ∶ 1. If A saves Rs. 2000 and B saves Rs. 4000, then what will be the income of A?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A sum of ₹ 159250 is divided among A, B, C, and D such that the ratio of the shares of A and B is 1 : 3, that of B and C is 2 : 5, and that of C and D is 2 : 3. The share (in ₹) of A is:
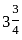 years at 8.8% p.a. at simple interest. What will be the simple interest (in Rs.) on the same sum at the same rate in
years at 8.8% p.a. at simple interest. What will be the simple interest (in Rs.) on the same sum at the same rate in 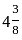 years?
years?
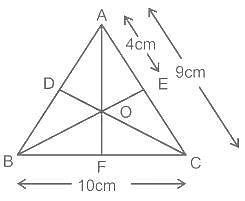
In the given triangle, O is the incentre, AE = 4 cm, AC = 9 cm and BC = 10 cm. What is the length of side AB?
The average age of Ram and Shyam is 28 years. If Rahim replaces Ram, then the average age will become 26 years. If Rahim replaces Shyam, then the average age will become 30 years. What are the respective ages of Ram, Shyam and Rahim?
The following bar graph shows the number of coolers sold by shopkeepers A and B in different years. Study the graph and answer the question that follows.
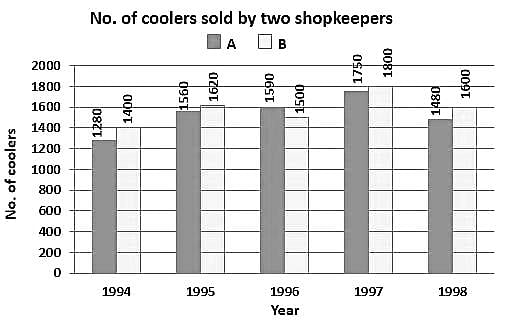
The total number of coolers sold by shopkeeper B in 1995 and 1998 together is approximately what percentage more/less than the total number of coolers sold by the shopkeeper A in 1996 and 1997 together ?
Find the least number which is completely divisible by 14, 15, and 18.
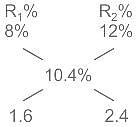
S deposits a total of ₹50,000 in two accounts which give 8% and 12% simple interest annually, respectively. After one year, he gets a total ₹5,200. How much money does he deposit in the account with a 12% interest rate?
What value will come in place of question mark (?) in the following question?
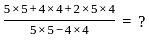
Monthly expenditure of a family has been shown in the following pie chart:
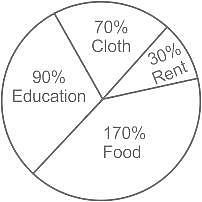
What is the percentage of expenditure on education out of total monthly expenditure?
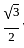 and sec(A - B) =
and sec(A - B) = 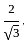 , 0° < A + B ≤ 90° and A > B, find A and B.
, 0° < A + B ≤ 90° and A > B, find A and B.
Marks (out of 100) of seven students in an examination are given below. Find the difference between their mean and median.
70, 55, 52, 85, 68, 67, 79
The areas of two similar triangles are 144 cm2 and 196 cm2 respectively. If the longest side of the smaller triangle is 24 cm, then find the longest side of the larger triangle.
The sum of the squares of 3 natural numbers is 261, and they are in the proportion 2 : 3 : 4. The difference between greatest number and smallest number is:
The production of a particular article in a company is 350 at present. It increases on an annual basis by 8% during the first year, 5% during the second year and 4% during the third year. What will be the approximate production after the third year?
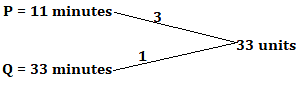
A pipe P can fill an empty tank in 11 minutes and pipe Q can empty the same full tank in 33 minutes. How much time it will take for both the pipe together to fill it 2/3 part of the tank?


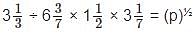 , then the value of 'p' is:
, then the value of 'p' is: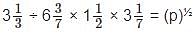
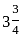
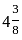
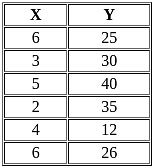
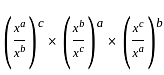 is:
is: