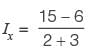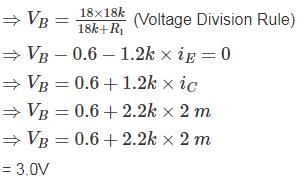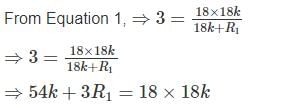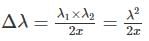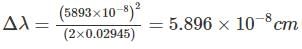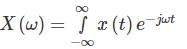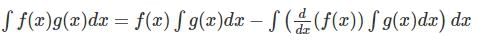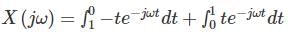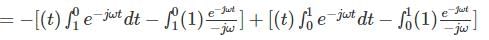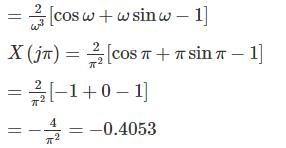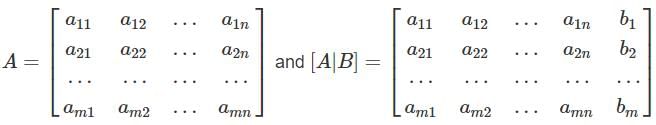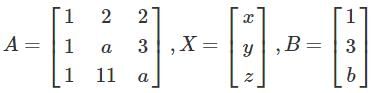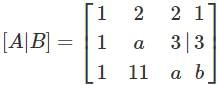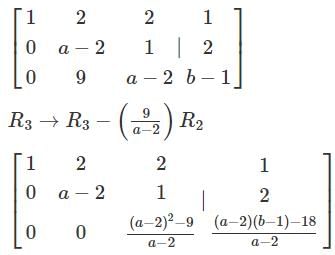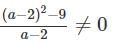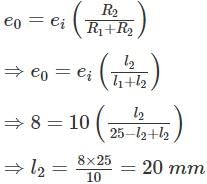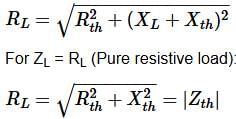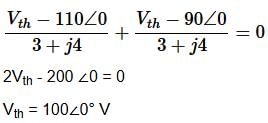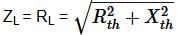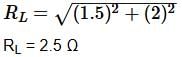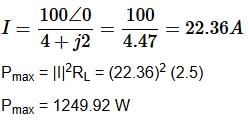GATE Instrumentation Mock Test - 4 - GATE Instrumentation MCQ
30 Questions MCQ Test - GATE Instrumentation Mock Test - 4
If 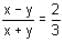 and x ≠ 0, what is the percentage (rounded to the nearest whole number) of x 3y that corresponds to x - 3y?
and x ≠ 0, what is the percentage (rounded to the nearest whole number) of x 3y that corresponds to x - 3y?
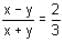 and x ≠ 0, what is the percentage (rounded to the nearest whole number) of x 3y that corresponds to x - 3y?
and x ≠ 0, what is the percentage (rounded to the nearest whole number) of x 3y that corresponds to x - 3y?
Which of the following statements must be considered TRUE regarding the mean and median of the scores obtained by all candidates who participated in GATE 2023?
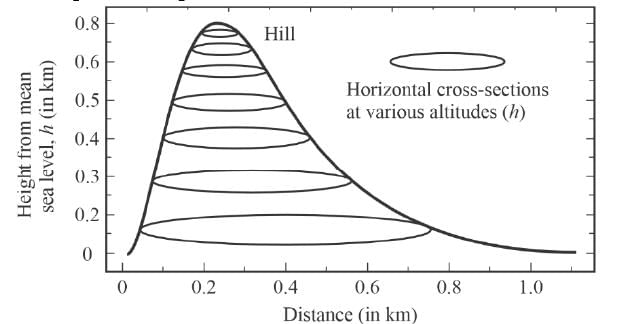
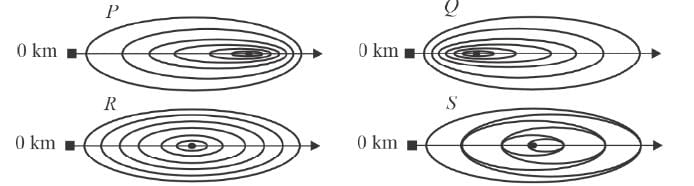
In the diagram provided, ovals are indicated at various elevations (h) on a hill. Which of the following options P, Q, R, and S represents the hill's top view?


Which of the following words is the opposite of "PROFESSIONAL"?
In a river, station B is positioned midway between stations A and C. A boat travels from A to B and returns in a total of 6 hours. If the journey from A to C takes 4 hours, what is the time required to travel from C back to A?
Identify the logic family that operates at the highest speed from the options given below:
In the MOSFET circuit displayed above,
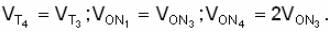
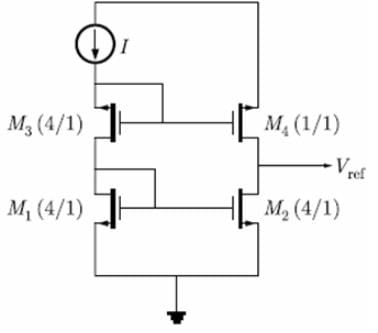
What is the value of the reference voltage VREF?
The low-frequency loop transfer function for a system with unity feedback is represented as follows:
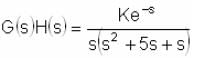
At which value of K will the system achieve stability?
The circuit illustrated below functions as a two-input NAND gate by utilizing two 2:1 multiplexers.
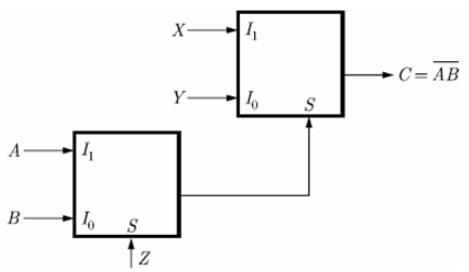
The respective values of X, Y, and Z are:
Determine the value of 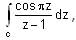 given that c represents the circle |z| = 3.
given that c represents the circle |z| = 3.
Evaluate the following assertions concerning a metal conducting sphere:
- The intensity of the electric field at a location outside the conducting sphere rises as the distance between the center of the sphere and the location increases.
- The intensity of the electric field at the surface of the conducting sphere is inversely related to the square of the sphere's radius.
- The intensity of the electric field within the conducting sphere is zero.
Which of the above assertion(s) is/are accurate?
Two large homogeneous isotropic dielectric materials intersect at the plane z = 0. For the region where z ≥ 0, the relative permittivity is εr1 = 4, and for the area where z ≤ 0, it is εr2 = 2. In the region z ≥ 0, there is a uniform electric field given by E1 = 5ax – 2ay 3az kV/m. What is the value of E2z in the region where z ≤ 0?
Consider z = x iy, where z is a complex variable and 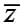 denotes its complex conjugate. The equation
denotes its complex conjugate. The equation 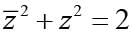 characterizes a
characterizes a
A discrete-time sequence defined as x [n] = [1, 2, 3, 4] for the range 0 ≤ n ≤ 3. What is the value of the zero lag auto-correlation for x [n]?
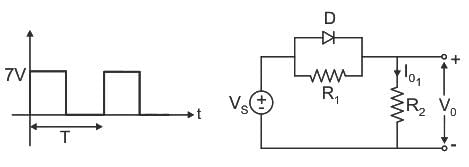
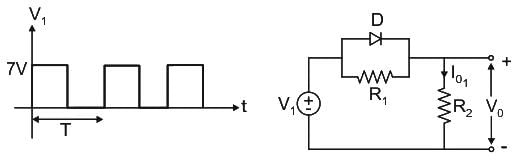
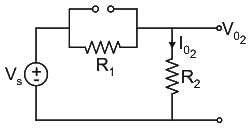
In the given circuit, Vs represents an asymmetric unipolar square wave with a period of T. The waveform has a duty cycle of 35%. Diode D is considered ideal, and both resistors R1 and R2 have a resistance of 14Ω. Which of the following statements is accurate?
How many complex multiplications are needed to compute a 16-point DFT using the decimation-in-time radix-2 FFT? Provide your answer as an integer.
Consider a periodic signal in discrete time represented as 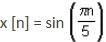 . Let ak denote the complex Fourier series coefficients of x[n]. The coefficients {ak} are non-zero for k = Bm ± 1, where m is any integer. What is the value of B?
. Let ak denote the complex Fourier series coefficients of x[n]. The coefficients {ak} are non-zero for k = Bm ± 1, where m is any integer. What is the value of B?
A differentiator possesses a transfer function that is defined by its
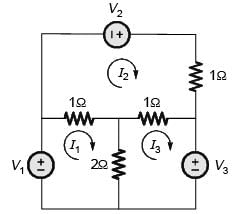
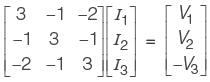
In the diagram provided below, I1, I2, and I3 represent the mesh currents. The appropriate set of mesh equations corresponding to these currents, expressed in matrix form, is _____.
The initial current of a 3φ induction motor is 10 times greater than its rated current, and the rated slip is 6%. What is the ratio of starting torque to the full load torque?
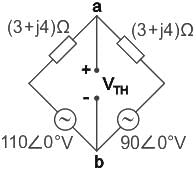
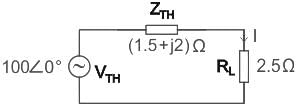
Instructions: Examine the circuit depicted in the figure below, which includes a load impedance ZL.
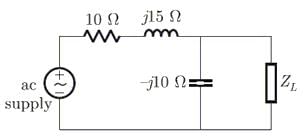
If the load impedance ZL is considered to be entirely resistive, what is the value of the load resistance (in Ω) that will maximize power absorption? Please provide your answer rounded to three decimal places.
A feedback system with unity configuration has an open-loop transfer function 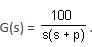 . The time at which the system's response to a unit step input reaches its maximum is π/8 seconds.
. The time at which the system's response to a unit step input reaches its maximum is π/8 seconds.
The damping ratio for the closed-loop system is ______ . (Provide the answer to one decimal place)
An acoustic pulse with a frequency of 5 MHz travels through a layer of fat tissue that is 2 cm thick before reaching a liver tissue interface at a right angle. The amplitude attenuation coefficients for fat and liver are 0.075 Npcm–1/MHz and 0.1 Npcm–1/MHz, respectively. The amplitude reflectivity coefficient at the fat-liver interface is 0.1. Considering both reflection and attenuation losses, what is the amplitude loss (in dB) of the echo pulse by the time it returns to the transducer?
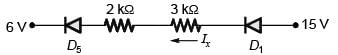
Assume the diodes in the circuit shown are ideal. The current Ix flowing through the 3 kΩ resistor (in mA, rounded off to one decimal place) is _____.
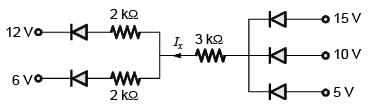
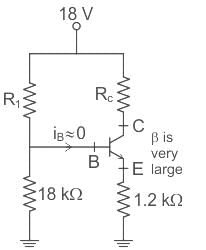
In the specified transistor arrangement, it is stated that the Quiescent current (ICQ) is 2 mA and the Quiescent Voltage from Collector to Emitter (VCEQ) equals 10 V. What is the value of R1 in __________ kΩ?
(Assume β to be significantly large and VBE = 0.6 V)
In Michelson's Interferometer, the measurements for a pair of indistinct maxima were recorded as 0.6939 mm and 0.9884 mm. Given that the average wavelength of the two light components is 5893 A°, what is the difference between the two wavelengths in A°?
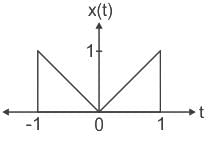
What is the value of the Fourier transform of the signal x(t) depicted in the image below at a frequency of π rad/sec?
Examine the following set of equations involving x, y, z:
- x 2y 2z = 1
- x ay 3z = 3
- x 11y az = b
For which positive value of a does the system fail to have a unique solution?
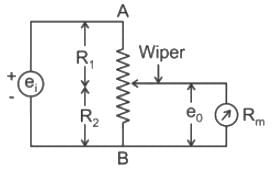
A resistive displacement transducer featuring a shaft stroke of 25 mm is utilized in the circuit depicted in the figure. The input voltage is 10 V. What is the displacement (in mm) indicated for a voltage measurement of 8 V? Assume that the resistance of the output device Rm is infinite.
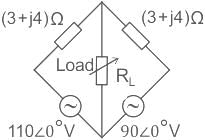
The highest amount of power that can be absorbed by the purely resistive load in the circuit depicted below is ________W


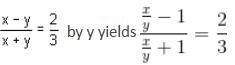
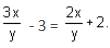
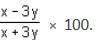
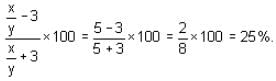

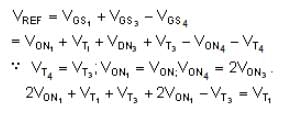
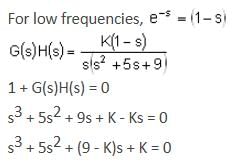
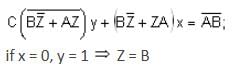
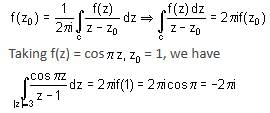
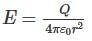
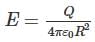
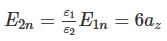
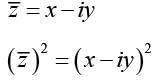
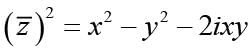
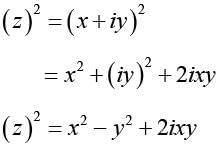
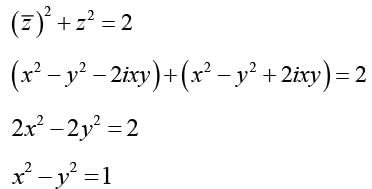
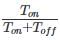
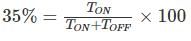
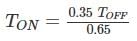
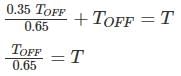
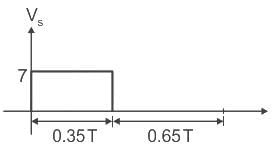
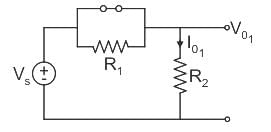
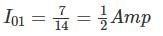

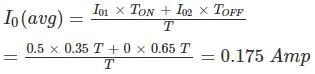
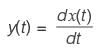
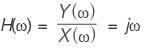
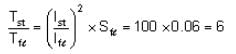
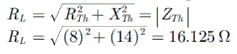
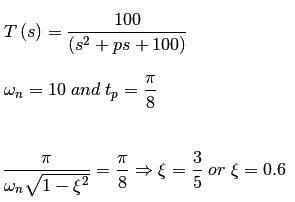

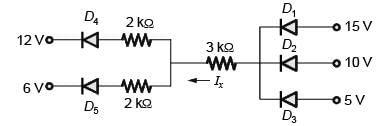
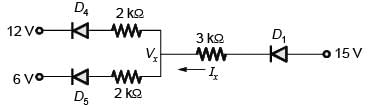
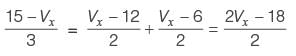
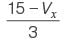 = Vx – 9 ⇒ Vx = 10.5 V
= Vx – 9 ⇒ Vx = 10.5 V