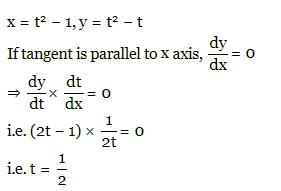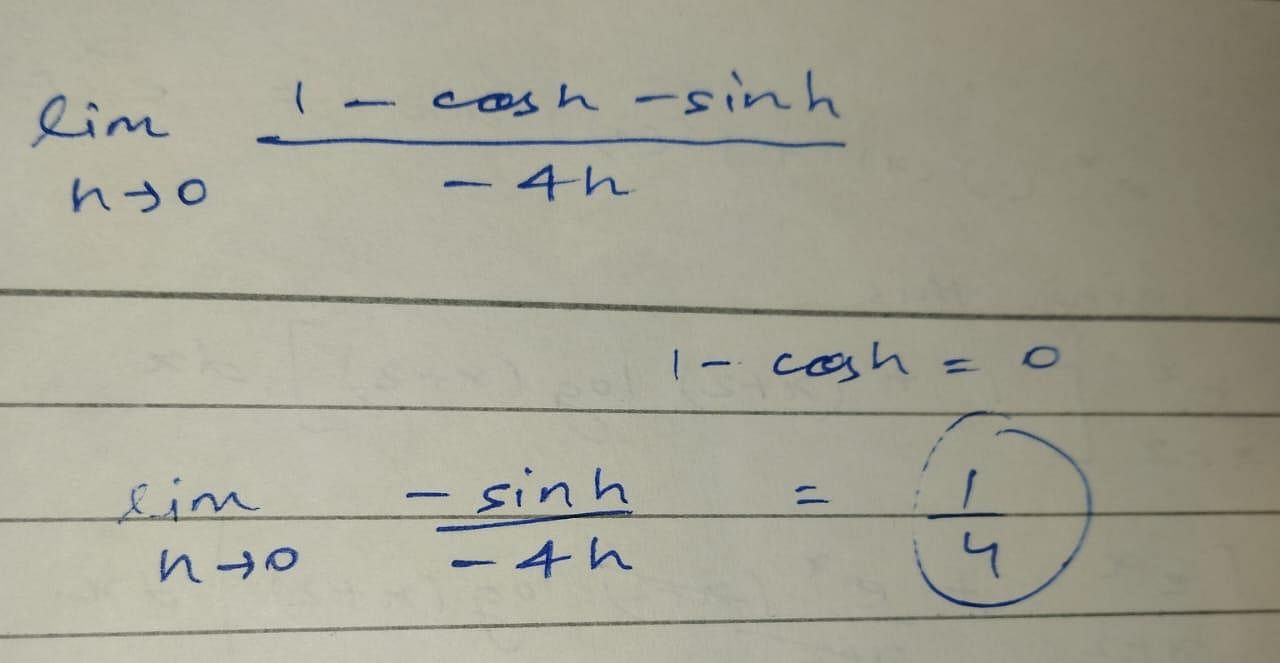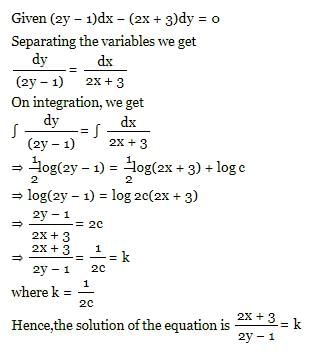All Courses
Get the App Signup / Login
Sign in Open App
JEE Exam  >  JEE Tests  >  VITEEE PCME Mock Test - 1 - JEE MCQ

VITEEE PCME Mock Test - 1 - JEE MCQ


Test Description

30 Questions MCQ Test - VITEEE PCME Mock Test - 1

VITEEE PCME Mock Test - 1 for JEE 2024 is part of JEE preparation. The VITEEE PCME Mock Test - 1 questions and answers have been prepared according to the JEE exam syllabus.The VITEEE PCME Mock Test - 1 MCQs are made for JEE 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for VITEEE PCME Mock Test - 1 below.
Solutions of VITEEE PCME Mock Test - 1 questions in English are available as part of our course for JEE & VITEEE PCME Mock Test - 1 solutions in Hindi for JEE course. Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt VITEEE PCME Mock Test - 1 | 125 questions in 150 minutes | Mock test for JEE preparation | Free important questions MCQ to study for JEE Exam | Download free PDF with solutions
VITEEE PCME Mock Test - 1 - Question 1
Save

For the curve x = t2 - 1, y = t2 - t tangent is parallel to x-axis where

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 1

VITEEE PCME Mock Test - 1 - Question 2
Save

The area between the curve y = 1 − | x | and x-axis is

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 2
Plot the graph of 1-|x| to plot it first you know graph of |x| then taking x-axis as the mirror take image of |x| then shift the the graph 1 unit upward that will be your final graph the 3 vertices of the triangle so formed will be (-1,0) ,(0,1), (1,0) then find the area it will be 1.
1 Crore+ students have signed up on EduRev. Have you? Download the App
VITEEE PCME Mock Test - 1 - Question 3
Save

If ω is a complex cube root of unity ,then the value of ω 99 + ω 100 + ω101 is

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 3
1+w+w²=0
divide w powers by 3 and put remainders as powers then you will get 0
VITEEE PCME Mock Test - 1 - Question 4
Save

If |(z-i)/(z+i)| = 1, then the locus of z is
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 4

VITEEE PCME Mock Test - 1 - Question 5
Save

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 5

VITEEE PCME Mock Test - 1 - Question 6
Save

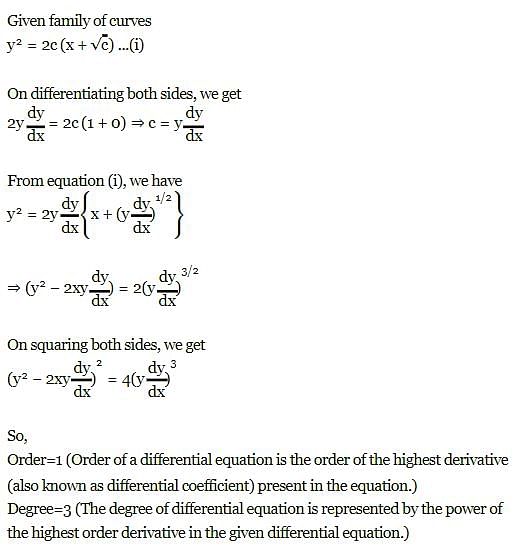
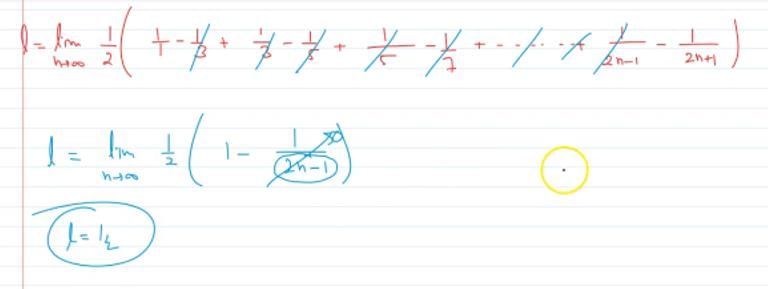
The differential equation representing the family of curves y2=2c(x+√c), where c is a positive perimeter, is of
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 6

VITEEE PCME Mock Test - 1 - Question 7
Save

If a, b, c are different 

VITEEE PCME Mock Test - 1 - Question 8
Save

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 8

VITEEE PCME Mock Test - 1 - Question 9
Save

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 9

 

VITEEE PCME Mock Test - 1 - Question 10
Save

 Δ
and A1 , B1 , C1  etc are cofactors of a 1 , b 1 , c 1 , then

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 10


 

Solution:

 


  • Explanation:

    • We know that the cofactor of a matrix is obtained by taking the determinant of the matrix formed by removing the row and column containing the element for which the cofactor is being calculated.

    • Given that A1, B1, C1, etc are cofactors of a1, b1, c1, it implies that A1 is the cofactor of a1, B1 is the cofactor of b1, and C1 is the cofactor of c1.

    • Therefore, A1 = Δ, B1 = Δ2, C1 = Δ3 (where Δ represents the determinant of the original matrix).


      •  

  • Conclusion:

    • So, the relationship between the cofactors A1, B1, C1 and the determinant Δ is A: Δ, B: Δ2, C: Δ3.

    • Hence, the correct answer is b. Δ2.


      •  


    •  
VITEEE PCME Mock Test - 1 - Question 11
Save

The solution of  is
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 11
2xydy/dx = 1 + y²

2 ydy/1 + y² = dx/x

Integrating

log(1 + y²) = logx + c

log(1 + y²/x) = c

1 + y²/x = e^c(new constant) = c′

1 + y² = cx
VITEEE PCME Mock Test - 1 - Question 12
Save

The solution of the equation (2y-1) dx-(2x+3) dy=0 is
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 12

VITEEE PCME Mock Test - 1 - Question 13
Save

Differential of x6 w.r. to x3 is equal to
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 13
Let
y = x^6
z = x³

To find : dy/dz

dy/dx = 6x^5

dz/dx = 3x²

dy/dx × dx/dz = 6x^5/3x²

= 2x³
VITEEE PCME Mock Test - 1 - Question 14
Save

If c is parameter then the differential equation whose solution is y = c2 + c/x is
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 14

 

VITEEE PCME Mock Test - 1 - Question 15
Save

The minimum value of sinx + cosx is
VITEEE PCME Mock Test - 1 - Question 16
Save

What is the value of 

VITEEE PCME Mock Test - 1 - Question 17
Save

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 17

VITEEE PCME Mock Test - 1 - Question 18
Save

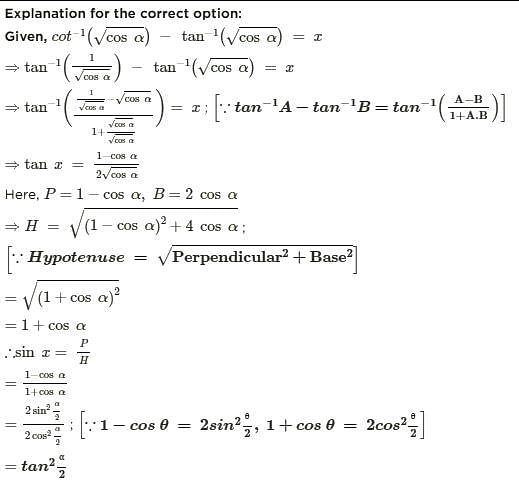
If cot-1[(cos α)1/2] - tan-1[(cos α)1/2] = x, then sin x =
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 18

VITEEE PCME Mock Test - 1 - Question 19
Save

Detailed Solution for VITEEE PCME Mock Test - 1 - Question 19

VITEEE PCME Mock Test - 1 - Question 20
Save

The function f(x) = sin-1 (cos x) is:
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 20

VITEEE PCME Mock Test - 1 - Question 21
Save

Directions: Study the following graph carefully and answer the question given below.

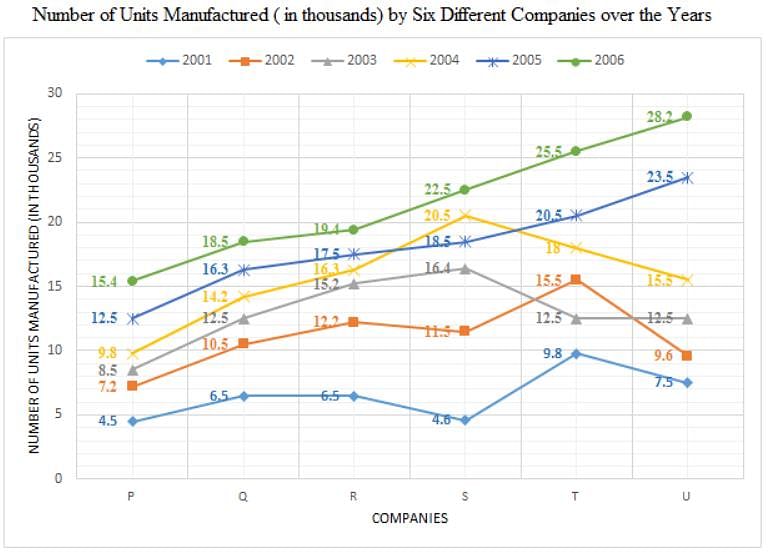
Q. What is the respective ratio of total number of units manufactured by companies Q and R together in the year 2001 to those manufactured by companies S and T together in the year 2003?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 21

The total number of units manufactured by companies Q and R together in the year 2001 = 6.5 + 6.5 = 13 thousands
The total number of units manufactured by companies S and T together in the year 2003 = 16.4 + 12.5 = 28.9 thousands
Required ratio = 13 : 28.9
= 130 : 289

VITEEE PCME Mock Test - 1 - Question 22
Save

Directions: Study the following graph carefully and answer the question given below.

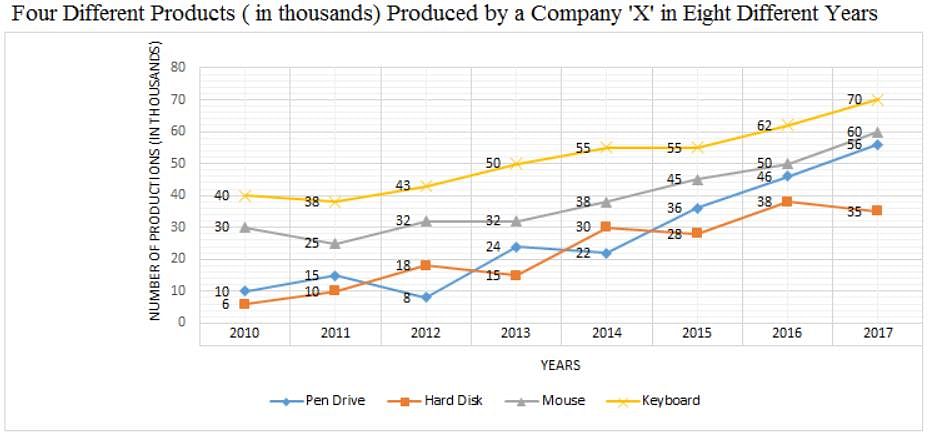
Q. What is the average number of hard disks produced by Company 'X' over all the years together?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 22

Total number of hard disks produced by Company 'X' over all the years together = 6 + 10 + 18 + 15 + 30 + 28 + 38 + 35 = 180 (in thousand) = 1,80,000

Required average = 1, 80, 000/8 = 22,500

VITEEE PCME Mock Test - 1 - Question 23
Save

Directions: Study the following graph carefully and answer the question given below.

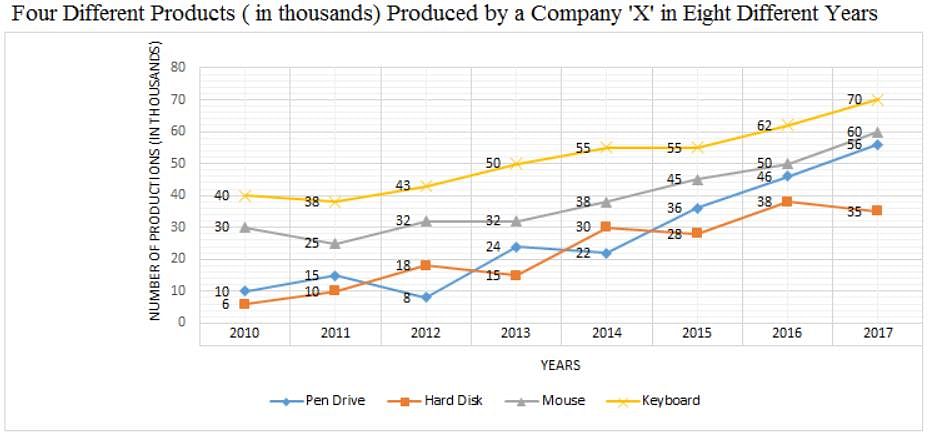
Q. What is the difference between the total number of hard disks and mouses produced by Company 'X' in the year 2010 and the total number of keyboards and pen drives produced by Company 'X' in the year 2015?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 23

Total number of hard disks and mouses produced by Company 'X' in the year 2010 = 6 + 30 = 36 thousand = 36,000
Number of keyboards and pen drives produced by Company 'X' in the year 2015 = 55 + 36 = 91 thousand = 91,000
Required difference = 91,000 - 36,000 = 55,000

VITEEE PCME Mock Test - 1 - Question 24
Save

Directions: Study the following graph carefully and answer the question given below.

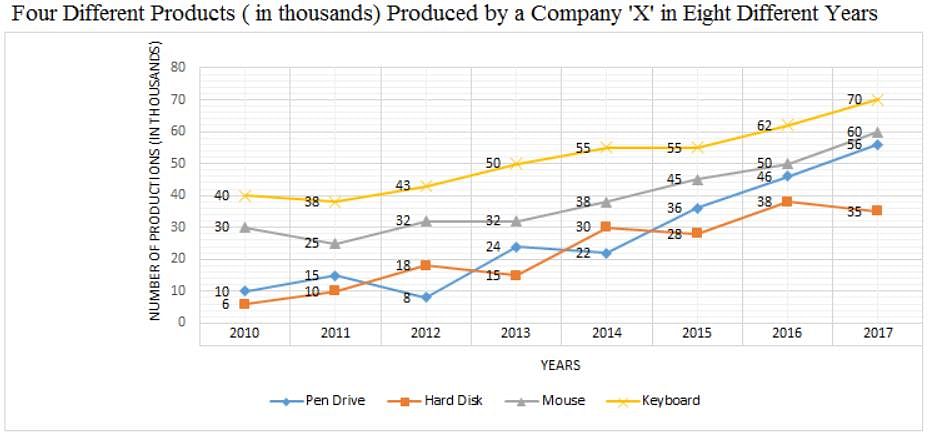
Q. What was the respective ratio between the number of pen drives produced by Company 'X' in the years 2010, 2011, 2014 and 2015 and the number of hard disks produced by Company 'X' in the years 2011, 2012, 2015 and 2017?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 24

The number of pen drives produced by Company 'X' in years 2010, 2011, 2014 and 2015 = 10 + 15 + 22 + 36 = 83 (in thousands)
The number of hard disks produced by Company 'X' in years 2011, 2012, 2015 and 2017 = 10 + 18 + 28 + 35 = 91 (in thousands)
Required ratio = 83 : 91

VITEEE PCME Mock Test - 1 - Question 25
Save

Anita had to do a multiplication. Instead of taking 35 as one of the multipliers, she took 53. As a result, the product went up by 540. What is the new product?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 25

Let us assume that the number with which Anita has to perform the multiplication is 'x'.

Instead of finding 35x, she calculated 53x.

The difference = 53x - 35x = 18x = 540

Therefore, x = 540/18 = 30

So, the new product = 30 x 53 = 1590.

VITEEE PCME Mock Test - 1 - Question 26
Save

A red light flashes three times per minute and a green light flashes five times in 2 min at regular intervals. If both lights start flashing at the same time, how many times do they flash together in each hour?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 26

A red light flashes three times per minute and a green light flashes five times in 2 min at regular intervals. So red light fashes after every 1/3 min and green light flashes every 2/5 min. LCM of both the fractions is 2 min.
Hence, they flash together after every 2 min. So in an hour they flash together 30 times.

VITEEE PCME Mock Test - 1 - Question 27
Save

Let S be the set of prime numbers greater than or equal to 2 and less than 100. Multiply all the elements of S. With how many consecutive zeroes will the product end?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 27

For number of zeroes we must count number of 2 and 5 in prime numbers below 100.
We have just 1 such pair of 2 and 5.
Hence we have only 1 zero.

VITEEE PCME Mock Test - 1 - Question 28
Save

In a stream, Q lies in between P and R such that it is equidistant from both P and R. A boat can go from P to Q and back in 6 hours 30 minutes while it goes from P to R in 9 hours. How long would it take to go from R to P?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 28

Since P to R is double the distance of P to Q,
Therefore, it is evident that the time taken from P to R and back would be double the time taken from P to Q and back (i.e. double of 6.5 hours = 13 hours).

Since going from P to R takes 9 hours, coming back from R to P would take 4 hours i.e. 13- 9 = 4

So Option A is correct

VITEEE PCME Mock Test - 1 - Question 29
Save

If A and B together can complete a piece of work in 15 days and B alone in 20 days, in how many days can A alone complete the work?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 29

A and B complete a work in = 15 days
⇒ One day's work of (A + B) = 1/ 15

B complete the work in = 20 days
⇒ One day's work of B = 1/20

⇒ A's one day's work = 1/15 − 1/20 = (4−3)/6 = 1/60

Thus, A can complete the work in = 60 days.

So Option A is correct

VITEEE PCME Mock Test - 1 - Question 30
Save

A and B together can do a piece of work in 50 days. If A is 40% less efficient than B, in how many days can A working alone complete 60% of the work?
Detailed Solution for VITEEE PCME Mock Test - 1 - Question 30

Given:

A and B together can do a piece of work in 50 days.

A is 40% less efficient than B

Concept used:

Total work = Efficiency of the workers × time taken by them

Calculation:

Let the efficiency of B be 5a

So, efficiency of A = 5a × 60%

⇒ 3a

So, total efficiency of them = 8a

Total work = 8a × 50

⇒ 400a

Now,

60% of the work = 400a × 60%

⇒ 240a

Now,

Required time = 240a/3a

⇒ 80 days

∴ A can complete 60% of the work working alone in 80 days.

View more questions
Information about VITEEE PCME Mock Test - 1 Page
In this test you can find the Exam questions for VITEEE PCME Mock Test - 1 solved & explained in the simplest way possible. Besides giving Questions and answers for VITEEE PCME Mock Test - 1, EduRev gives you an ample number of Online tests for practice

Top Courses for JEE

Download as PDF

Top Courses for JEE

Important Questions for VITEEE PCME Mock Test - 1

Find all the important questions for VITEEE PCME Mock Test - 1 at EduRev.Get fully prepared for VITEEE PCME Mock Test - 1 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the VITEEE PCME Mock Test - 1 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of VITEEE PCME Mock Test - 1 resources.

VITEEE PCME Mock Test - 1 MCQs with Answers

Prepare for the VITEEE PCME Mock Test - 1 within the JEE exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for VITEEE PCME Mock Test - 1

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace VITEEE PCME Mock Test - 1 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup