All Courses
Get the App Signup / Login
Sign in Open App
Mechanical Engineering Exam  >  Mechanical Engineering Tests  >  Test: Addition Of A System Of Coplanar Forces - Mechanical Engineering MCQ

Test: Addition Of A System Of Coplanar Forces - Mechanical Engineering MCQ


Test Description

14 Questions MCQ Test - Test: Addition Of A System Of Coplanar Forces

Test: Addition Of A System Of Coplanar Forces for Mechanical Engineering 2024 is part of Mechanical Engineering preparation. The Test: Addition Of A System Of Coplanar Forces questions and answers have been prepared according to the Mechanical Engineering exam syllabus.The Test: Addition Of A System Of Coplanar Forces MCQs are made for Mechanical Engineering 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Addition Of A System Of Coplanar Forces below.
Solutions of Test: Addition Of A System Of Coplanar Forces questions in English are available as part of our course for Mechanical Engineering & Test: Addition Of A System Of Coplanar Forces solutions in Hindi for Mechanical Engineering course. Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free. Attempt Test: Addition Of A System Of Coplanar Forces | 14 questions in 15 minutes | Mock test for Mechanical Engineering preparation | Free important questions MCQ to study for Mechanical Engineering Exam | Download free PDF with solutions
Test: Addition Of A System Of Coplanar Forces - Question 1
Save

Which of the following statement is true?

Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 1

A scalar is any positive or negative physical quantity that can be completely specified by its magnitude. Examples of scalar quantities include length, mass, time, etc.

Test: Addition Of A System Of Coplanar Forces - Question 2
Save

For two vectors defined by an arrow with a head and a tail. The length of each vector and the angle between them represents:

Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 2

For two vectors defined by an arrow with a head and a tail. The length of each vector and the angle between them represents their magnitude and direction of the line of action respectively. The head/tip of the arrow indicates the sense of direction of the vector.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Addition Of A System Of Coplanar Forces - Question 3
Save

If a vector is multiplied by a scalar:

Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 3

If a vector is multiplied by a scalar then its magnitude is increased by amount of that scalar’s magnitude. When multiplied by a negative scalar it going to change the directional sense of the vector.

Test: Addition Of A System Of Coplanar Forces - Question 4
Save

All the vectors quantities obey:
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 4

All the vectors quantities obey parallelogram law of addition. Two vectors A and B (can be called as component vectors) are added to form a resultant vector. R = A+B.

Test: Addition Of A System Of Coplanar Forces - Question 5
Save

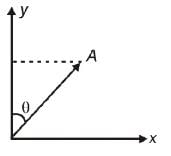
A force vector with magnitude R and making an angle α with the x-axis is having its component along x-axis and y-axis as:
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 5

The component along x-axis is the cosine component of the vector. And the y-axis component of the vector is sine component, if the angle is being made with the x-axis. And 180- α for some of the trigonometric function may change their sign.

Test: Addition Of A System Of Coplanar Forces - Question 6
Save

Dividing the X-axis component and the Y-axis component of the of the vector making an angle with Y-axis θ will give us.
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 6


tan θ = P/B
         = x component / y component 

Test: Addition Of A System Of Coplanar Forces - Question 7
Save

Vector shown in the figure below have a length of 3m and the angles shown A and B are 60 and 30 degrees each. Calculate the X-axis and Y-axis components:
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 7

The sine and the cosine components of the given vectors considering the angle B as the only angle of consideration comes 1.5m and 2.59m.

Test: Addition Of A System Of Coplanar Forces - Question 8
Save

Shown as in the figure below, A=60 degree and B=30 degree. Calculate the total length obtained by adding the x-axis component of both the vectors.
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 8

After getting the cosine components of the given vectors we obtain the total length of the x-axis components to be 3cos60 + 2cos30 = 3.23.

Test: Addition Of A System Of Coplanar Forces - Question 9
Save

The magnitude of the resultant of the two vectors is always_____________
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 9

Yes, the magnitude of the resultant of the two vectors always depends on the angle between them. It might be greater or smaller than one of the vector’s length. For perfectly saying, it does depends upon the angle between them.

Test: Addition Of A System Of Coplanar Forces - Question 10
Save

If two equal vector forces are mutually perpendicular then the resultant force is acting at which angle as compared to one of the vector?
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 10

The vectors are mutually perpendicular, this means that the angle between the forces is 90 degree. Thus the resultant will form at 45 degrees to any of the vector.

Test: Addition Of A System Of Coplanar Forces - Question 11
Save

What is the direction of the resultant vector if two vectors having equal length is placed in the Cartesian plane at origin as, one being parallel to and heading towards positive x-axis and the other making 165 degree with it and heading in the opposite direction of that of the first one?
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 11

 If one is heading towards positive X-axis and the other is in the other direction opposite to the first one, with both having the same length and having an angle between them being obtuse, means that the direction is to be in the direction of either 1st quadrant or in the 4th quadrant.

Test: Addition Of A System Of Coplanar Forces - Question 12
Save

Force vector R is having a______________
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 12

As it is a force vector, it means it is going to have a direction and a magnitude. The magnitude is not the square root of R, R is just the magnitude of the vector given.

Test: Addition Of A System Of Coplanar Forces - Question 13
Save

The resultant of three equal vectors having mutual angles being 120 degree and being originated from a single point is zero.
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 13

As one can imagine three vectors coming out of a single point and are having a 120 degree angle with their adjacent ones, the resultant would be zero.

Test: Addition Of A System Of Coplanar Forces - Question 14
Save

Every point on the force vector is having the same magnitude and the same direction as the whole force vector have.
Detailed Solution for Test: Addition Of A System Of Coplanar Forces - Question 14

The vector is made by joining the final and the starting point. If we consider any arbitrary point then the length would be calculated w.r.t the starting point, which might give different magnitude but same direction.

Information about Test: Addition Of A System Of Coplanar Forces Page
In this test you can find the Exam questions for Test: Addition Of A System Of Coplanar Forces solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Addition Of A System Of Coplanar Forces, EduRev gives you an ample number of Online tests for practice

Top Courses for Mechanical Engineering

Download as PDF

Top Courses for Mechanical Engineering

Important Questions for Addition Of A System Of Coplanar Forces

Find all the important questions for Addition Of A System Of Coplanar Forces at EduRev.Get fully prepared for Addition Of A System Of Coplanar Forces with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Addition Of A System Of Coplanar Forces syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Addition Of A System Of Coplanar Forces resources.

Addition Of A System Of Coplanar Forces MCQs with Answers

Prepare for the Addition Of A System Of Coplanar Forces within the Mechanical Engineering exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Addition Of A System Of Coplanar Forces

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Addition Of A System Of Coplanar Forces with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup