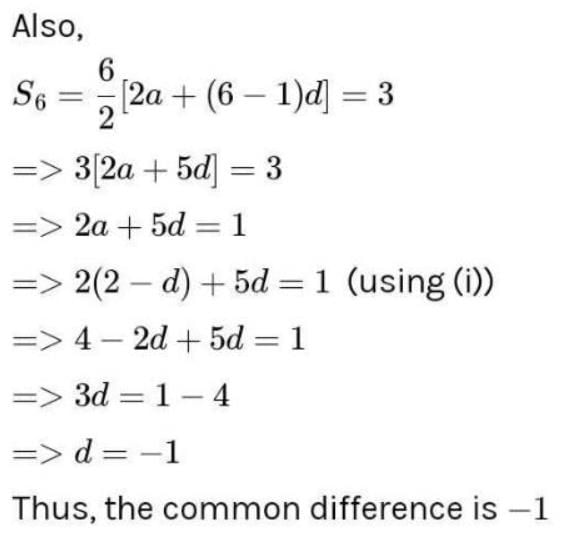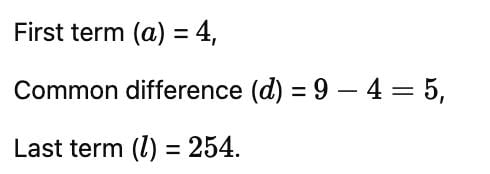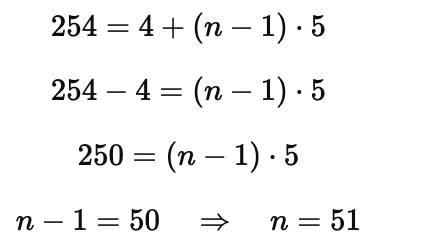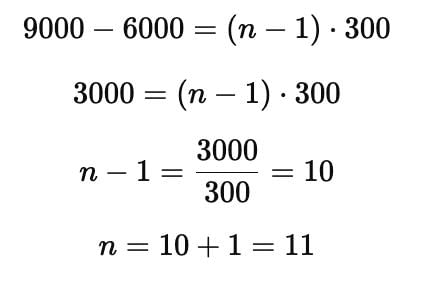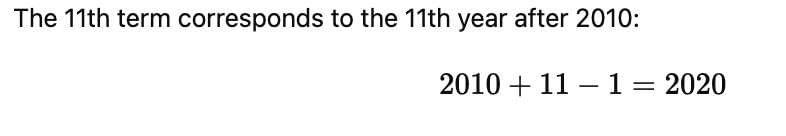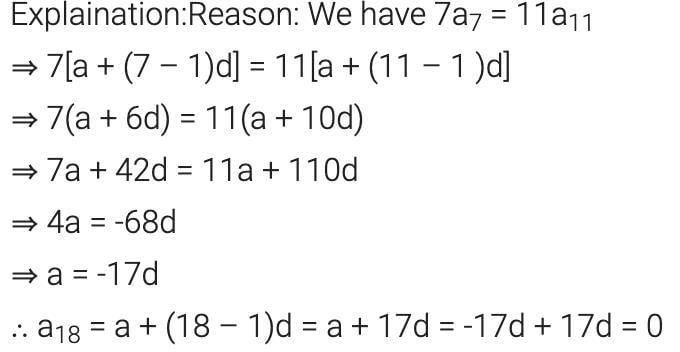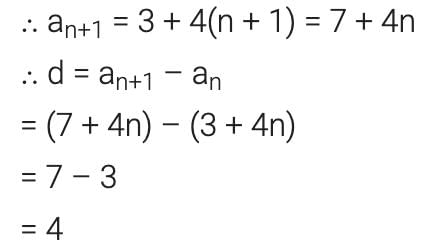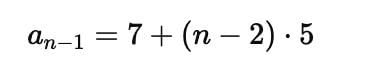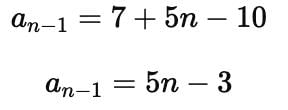Practice Test: Arithmetic Progressions - Class 10 MCQ

The fourth term of an A.P. is 4. Then the sum of the first 7 terms is :

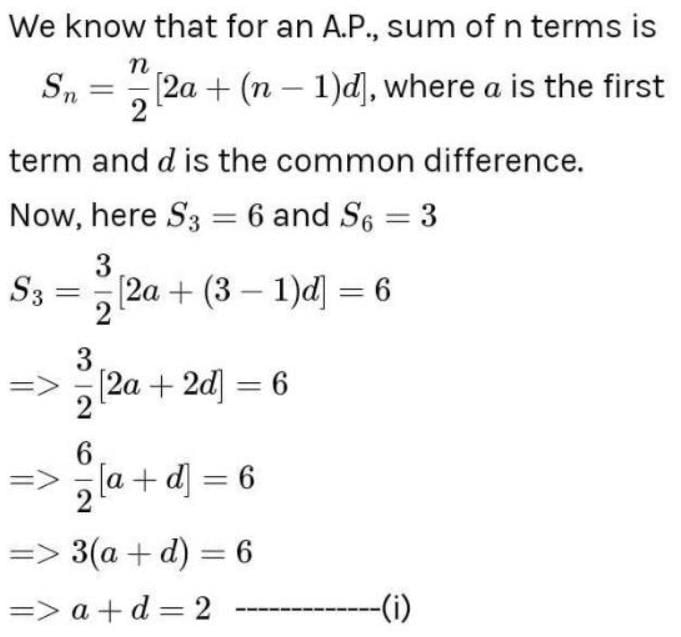
In an A.P. s₃ = 6, s₆ = 3, then it's common difference is equal to :

The 15th term from the last term of the AP: 4,9,14,......254 is :

Ramesh started work in 2010 with an annual salary of ₹6000 and received an increment of ₹300 each year. In which year did his income reach ₹9000?

The sum of first n odd natural numbers is

If 7 times the 7th term of an A.P. is equal to 11 times its 11th term, then 18th term is

Find the sum of 12 terms of an A.P. whose nth term is given by an = 3n + 4

The n^th term of an A.P. is given by a_n = 3 + 4n. The common difference is

The (n – 1)th term of an A.P. is given by 7,12,17, 22,… is

If {a_n} = {2.5, 2.51, 2.52,...} and {b_n} = {3.72, 3.73, 3.74,...} be two AP's, then a₁₀₀₀₀₅ – b₁₀₀₀₀₅ =

 In an Arithmetic Progression, if a = 28, d = -4, n = 7, then a_n is:

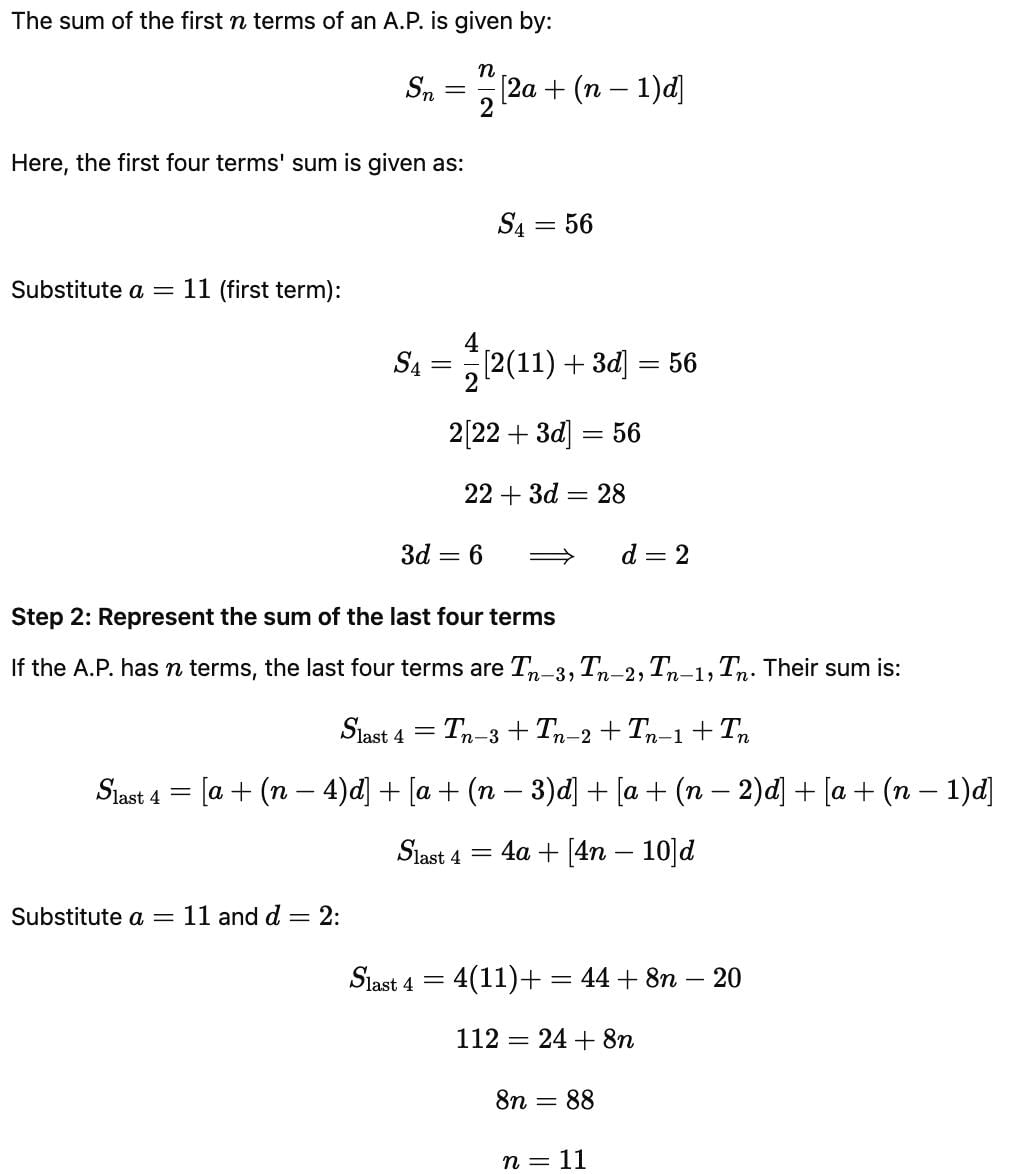
The sum of the first four terms of an A.P. is 56. The sum of the last four terms is 112. If its first term is 11, then find the number of terms.