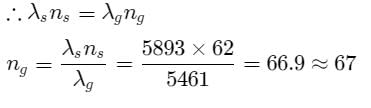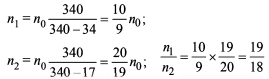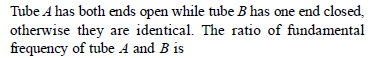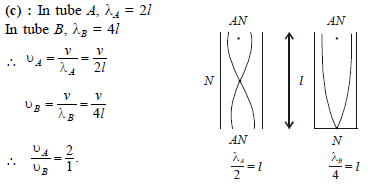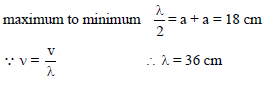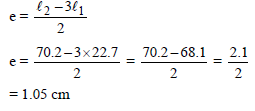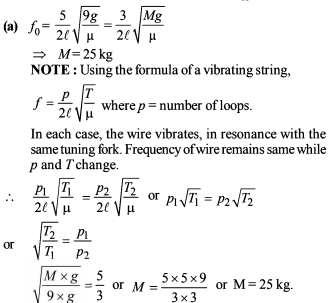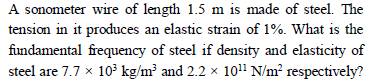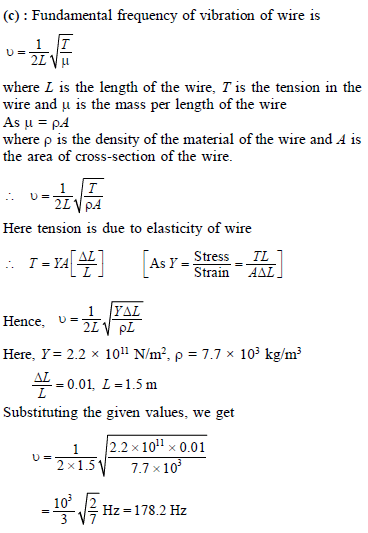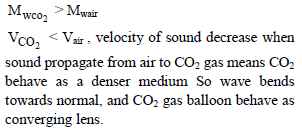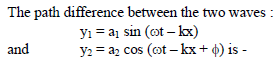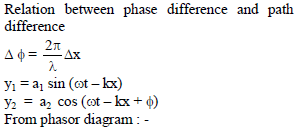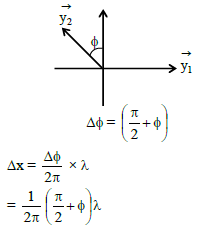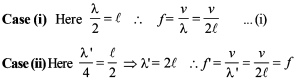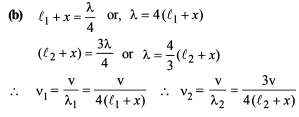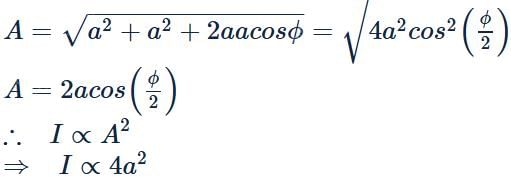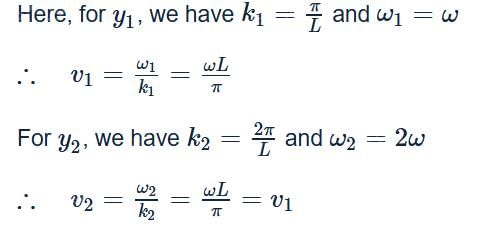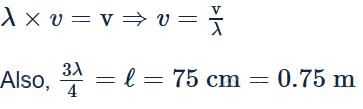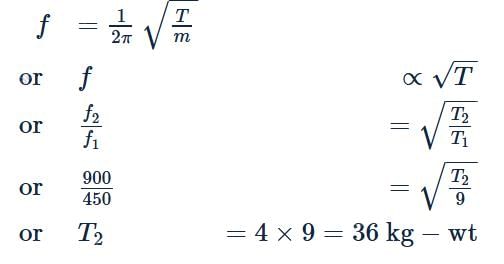Chapter Test: Waves - 1 - JEE MCQ

In young's double slit experiment, interference pattern is observed on the screen L distance apart from slits, average distance between adjacent fringes is x and slits separation is d, then the wavelength of light will be _

A tuning fork of frequency n is sounded at the open end of a long cylindrical tube having a side opening and fitted with a movable reflecting piston. On moving the piston through 9 cm, the intensity of sound heard by the listener changes from maximum to minimum. If speed of sound is 360 m/s, value of n is 

In a resonance pipe the first and second resonance are obtained at lengths 22.7 cm and 70.2 cm respectively. What will be the end correction -

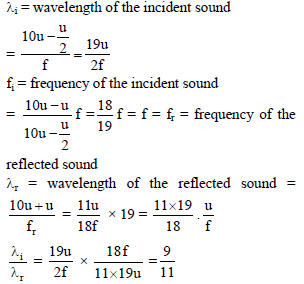
A wall is moving with velocity u and a source of sound moves with velocity u/2 in the same direction as shown in the figure. Assuming that the sound travels with velocity 10u. The ratio of incident sound wavelength on the wall to the reflected sound wavelength by the wall, is equal to -

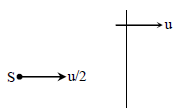
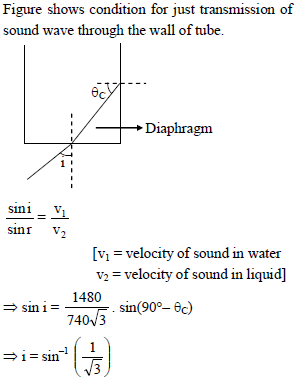
One end of a thin metal tube is closed by thin diaphragm of latex and the tube is lower in water with closed end downward. The tube is filled with a liquid 'x'. A plane progressive wave inside water hits the diaphragm making an angle 'θ' with its normal. Assuming Snell's law to hold true for sound. Maximum angle 'θ' for which sound is not transmitted through the walls of tube is (velocity of sound in liquid x = 740√3 m/s and in water = 1480 m/s)

A tuning fork and an air column in resonance tube whose temperature is 51°C produces 4 beats in 1 second when sounded together. When the temperature of the air column decreases, the number of beats per second decreases. When the temperature remains 16°C, only 1 beat per second is produced. Then the

frequency of the tuning fork is -

A boat at anchor is rocked by waves whose crests are 100 m apart and velocity is 25 m/s. The boat bounces up once in every -

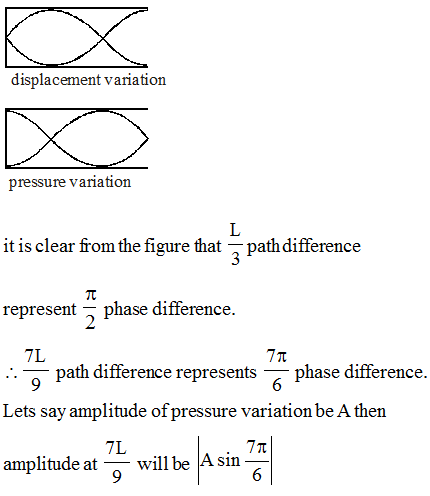
A closed organ pipe of length L is vibrating in its first overtone. There is a point Q inside the pipe at a distance 7 L/9 from the open end. The ratio of pressure amplitude at Q to the maximum pressure amplitude in the pipe is

A balloon filled with CO₂, then for sound wave this will behave as a -

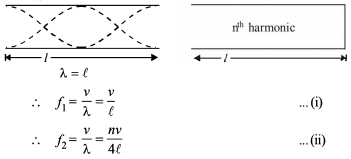
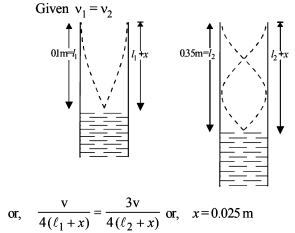
The first overtone of an open organ pipe and the fundamental tone of a closed organ pipe give 5 beats per second when sounded together. If the length of the closed pipe is 25 cm, what are the possible lengths of the open organ pipe (Speed of sound in air = 340 m/sec) -

The superposition takes place between two waves of frequency f and amplitude a. The maximum intensity I_max = constant ×____

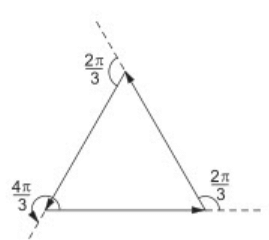
A point mass is subjected to two simultaneous sinusoidal displacements in the x-directions: x₁(t)=Asinωt and x₂(t)=Asin(ωt+2π/3). Adding a third sinusoidal displacement x(t)=Bsin(ωt+ϕ) brings the mass to a complete rest. The values of B and ϕ are respectively

The ends of a stretched wire of length L are fixed at x=0 and x=L. In one experiment, the displacement of the wire is y₁=Asin(πx/L) sin ωt and the energy is E₁, and in another experiment the displacement is y₂=Asin(2πx/L)sin2ωt and the energy is E₂. Then

A vibrating string of certain length ℓ under a tension T resonates with a mode corresponding to the first overtone (third harmonic) of an air column of length 75 cm inside a tube closed at one end. The string also generates 4 beats per second when excited along with a tuning fork of frequency n. Now when the tension of the string is slightly increased the number of beats reduces 2 per second. Assuming the velocity of sound in air to be 340 m/s, the frequency n of the tuning fork in Hz is

A segment of wire vibrates with a fundamental frequency of 450 Hz under a tension of 9 kgwt. Then tension at which the fundamental frequency of the same wire becomes 900 Hz is