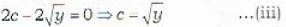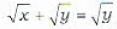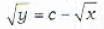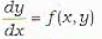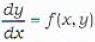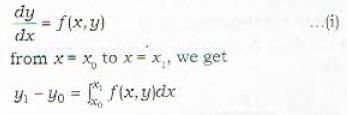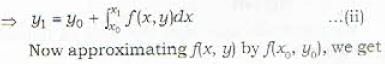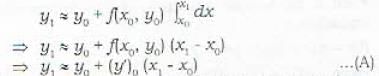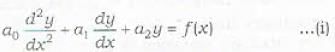Differential Equations - 19 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Differential Equations - 19
Which of the following differential equations is known as Clairaut’s equation?
The general solution of the differential equation (y - px) (p - 1) = p is given by
The general solution of i he differential equation sin(y - px) = p is given by
The differential equation e3x(p - 1) + p3 e2y = 0 can be reduced to Clairaut’s form by means of the substitution
Which of the following differential equations cannot be transformed to the Clairaut’s form by means of the substitution x2 = u and y2 = v ?
The differential equation x2p2 + yp(2x + y) + y2 = 0 can be reduced to the Clairaut’s form by means of which of the following substitutions?
Which of the following statements is incorrect regarding the singular solutions of the differential equations?
To obtain the singular solution of the differential equation in Clairaut’s form it is necessary to calculate
The singular solution (s) of the differential equation 4xp2 = (3x - a)2 is/arc given by
The differential equation p2 + y2 = 1 has how many singular solutions?
The differential equation p2(1 - x2) = 1 - y2 has how many singular solutions?
The singular solution(s) of the differential equation 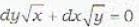 is/are given by
is/are given by
The singular solution(s) of the differential equation 8ap3 = 27y is/are given by
The general solution of the differential equation (a2 - x2)p2 + 2xyp + (b2 - y2) = 0 represents a family of straight lines whose envelope is the
The Euler’s method provides the solution of which of the following firsi order differential equations
The Euler’s method for solving a differential equation of the type dy/dx = f(x, y) provides
The solution of the differential equation dy/dx = f(x, y) through a given point (x0, y0) can be written as y = F(x). The Euler's method determines discrete points on the solution curve y = F(x). Let (x1 , y1,) be first point calculated and let (y')0 denote the value of dy/dx at (x0, y0) then
Let (x2, y2) be the second point calculated by the Euler’s method on the solution curve y = F(x). Then
Let the solution of the differential equation dy/dx = x + y pass through (0, 1) and let (0.05, y1) be the first point calculated on the solution curve by the Euler’s method. Then y1 is equal to
Consider the differential equation
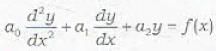
This will be differential equation with constant coefficients if
|
27 docs|150 tests
|


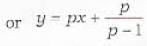 ......(ii)
......(ii)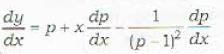
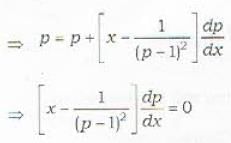
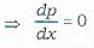
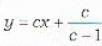


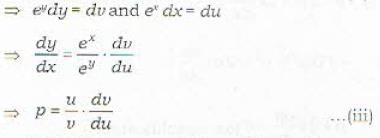
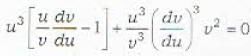
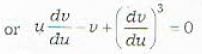
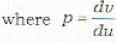
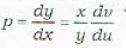 .....(ii)
.....(ii)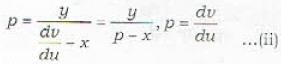
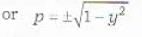 ...(iii)
...(iii)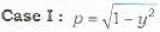
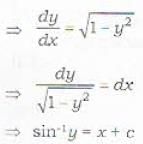
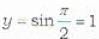
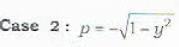
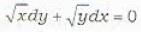

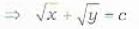 ......(i)
......(i)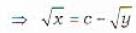
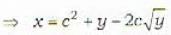 ...(ii)
...(ii)