Differential Equations - 7 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Differential Equations - 7
Let y (x) = x sin x be one of the solution of an nth order linear differential equation with constant coefficients.
Then the minimum value of n is
Then the minimum value of n is
The general solution of the differential equation y"(x) - 4y'(x) + 8y(x) = 10ex cos x is
The general solution of the differential equation ( x + y - 3) dx - (2x + 2y + 1) dy = 0 is
The solution of the differential equation yy' + y2 - x = 0, where c is a constant, is
The differential equation representing all circles centred at (1, 0) is
The differential equation y" + 6y' + 9y = 50 e2x have particular integral
The c-discriminant does not contain one of the following,
Let φ1( (x) and φ2(x) are particular integral of L(y) = eax - f (x), a, b being constants, then a PI of L(y) = 2 beax is
Solution of the differential equation (a2 - 2xy - y2) dx = (x +y)2 dy is
The slope of a curve at any point is the reciprocal of twice the ordinate at the point and it passes through the point (4, 3). The equation of the curve is
Order and degree of the differential equation 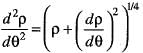 are respectively
are respectively
|
27 docs|150 tests
|


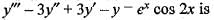
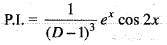
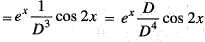
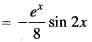
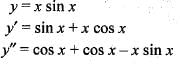
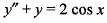 ...(i)
...(i)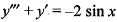
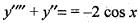 ...(ii)
...(ii)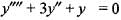

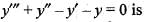
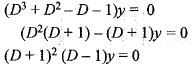
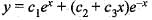
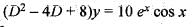
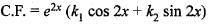
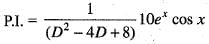
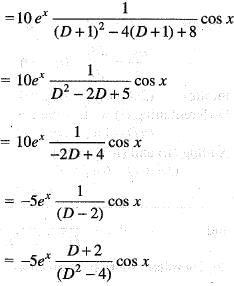
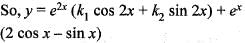
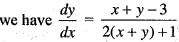
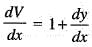
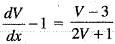
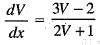
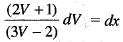
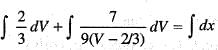

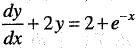 with y(0) = 0, then
with y(0) = 0, then 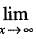 y(x) equals
y(x) equals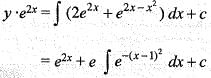
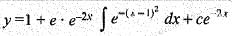
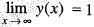
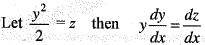
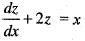
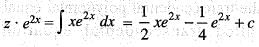

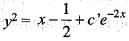
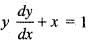
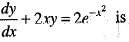

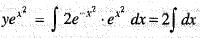
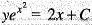
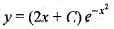
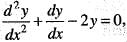 has the solution
has the solution 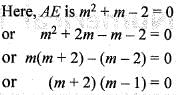
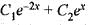
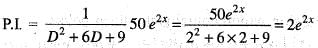
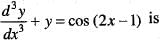

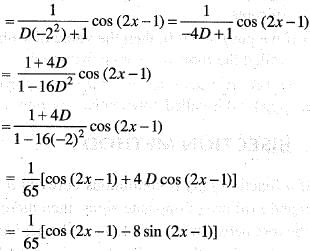
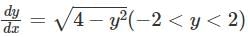
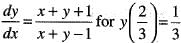 is
is











