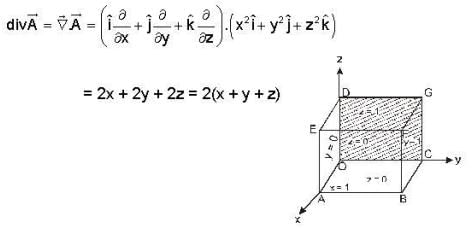
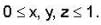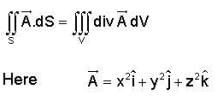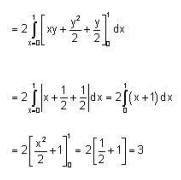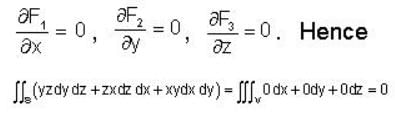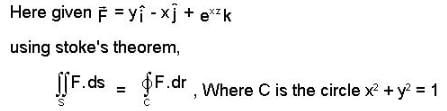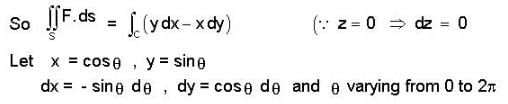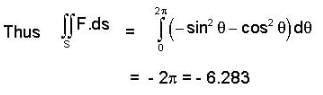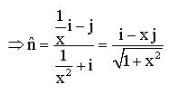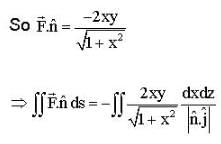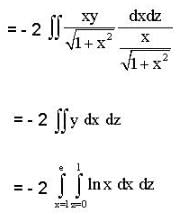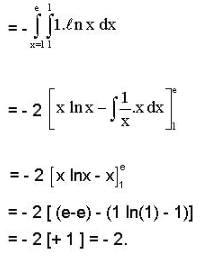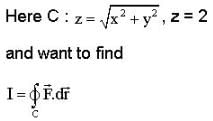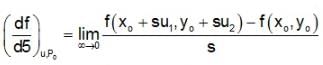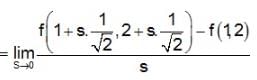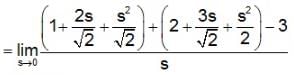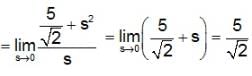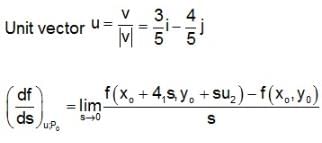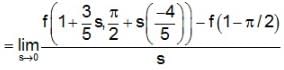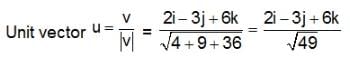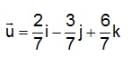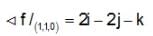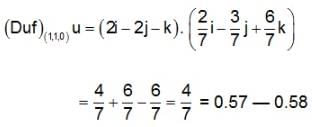IIT JAM Mathematics Practice Test- 10 - Mathematics MCQ
30 Questions MCQ Test - IIT JAM Mathematics Practice Test- 10
A non - zero vector 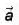 is parallel to the line of intersection of the plane determined by the vectors i, i +j and the plane determined by the vectors i - j, i + k. Then the angle between
is parallel to the line of intersection of the plane determined by the vectors i, i +j and the plane determined by the vectors i - j, i + k. Then the angle between 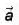 and the vector i - 2j + 2k is ,
and the vector i - 2j + 2k is ,
The unit normal to the surface xy2 + 2xz = 4 at the point (2, -2, 3) is
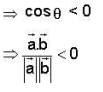
The values of x for which the angle between the vectors  is obtuse,
is obtuse,
The value of line integral  and C is the boundary curve of the upper half surface of the sphere x2 + y2 + z2 = 1.
and C is the boundary curve of the upper half surface of the sphere x2 + y2 + z2 = 1.
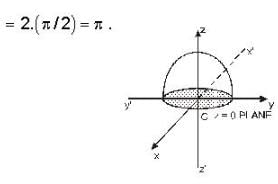
The value of 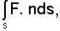 where F = 2xi + 3yj + 4zk and S is the surface of sphere x2 + y2 + z2 = 1
where F = 2xi + 3yj + 4zk and S is the surface of sphere x2 + y2 + z2 = 1
The differential equation of the family of parabolas with foci at the arigin and axis along the x-axis is)
Let y(x) = 4(x) sinx + v(x) cos x be a solution of the differential equation, y”+y sec x, Then 4(x) is
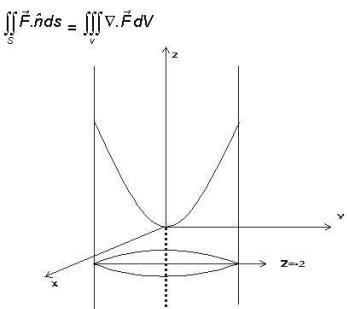
Let W be the region inside the solid cylinder x2 + y2 < 9 between the plane z = - 2 and the parabolio d z = x2 + y2. Let S is the boundary of W and 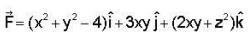 be a vector field. Then the value of
be a vector field. Then the value of 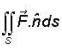 cannot be ?
cannot be ?
If 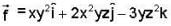 then at the point (1 -1 ,1 ), select the correct statements:
then at the point (1 -1 ,1 ), select the correct statements:
The value of line integral 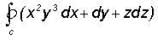 where C is the circle x2 + y2 = 16 , z = -1, is
where C is the circle x2 + y2 = 16 , z = -1, is
if  are unit vectors, then the possible values of
are unit vectors, then the possible values of 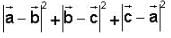 is/are
is/are
The directional derivative of f at P in the direction of  with
with 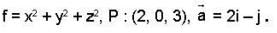
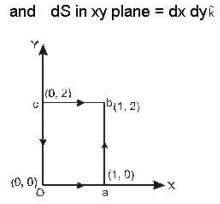
The value of 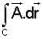 for the vector field
for the vector field 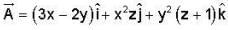 for a plane rectangular area with vertics at
for a plane rectangular area with vertics at 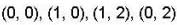 in x-y plane, is ____________.
in x-y plane, is ____________.
The value of surface integral
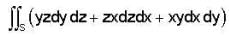
Where S is closed Sufrace 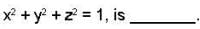
Let S be the surface with the indicated orientaton 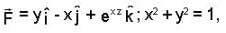 then the value of
then the value of 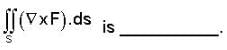
Let S be the portion of the cylinder y = Inx in the first octant whose projection parallel to the y-axis on to the xz-plane is the rectangle Rxz : 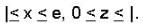 Let
Let 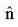 be the unit vector normal to S that points away from the xz-plane. Let
be the unit vector normal to S that points away from the xz-plane. Let 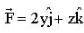 be a rector field, then the value of integral
be a rector field, then the value of integral 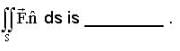
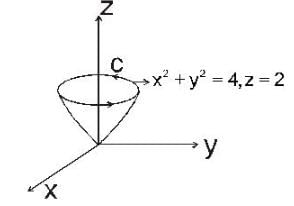
The circulation of the field 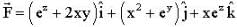 around the curve C in which the plane z = 2 meets the cone
around the curve C in which the plane z = 2 meets the cone  Counterclock wise as viewed from above, is equal to ....... (correct upto two decimal places)
Counterclock wise as viewed from above, is equal to ....... (correct upto two decimal places)
The direcitional derivative of 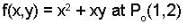 in the direction of the unit vector
in the direction of the unit vector 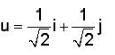
Find the directional derivative of 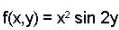 at the point
at the point 
Find the derivation of 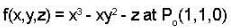 in the direction v = 2i - 3j + 6k
in the direction v = 2i - 3j + 6k


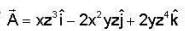 find (p.q) where
find (p.q) where  at ( 1 - 1, 1).
at ( 1 - 1, 1).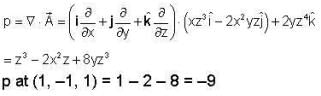
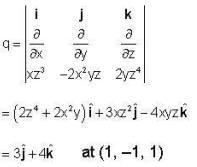
 then the point (1,-1,2), we have
then the point (1,-1,2), we have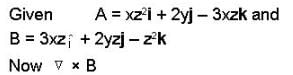
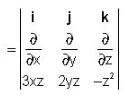
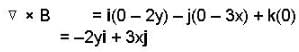
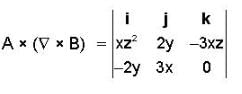
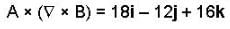 (1)
(1)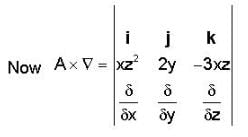
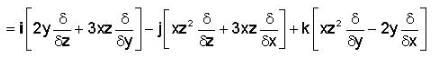
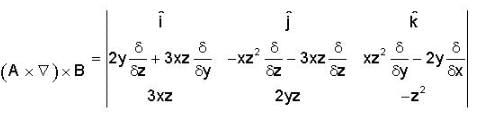
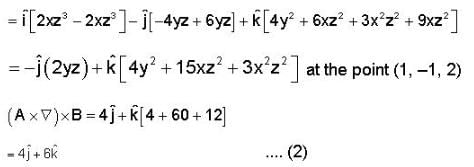


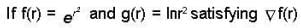
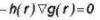 then
then 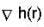 is equal to,
is equal to,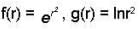
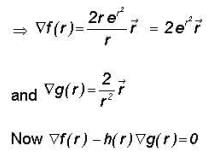
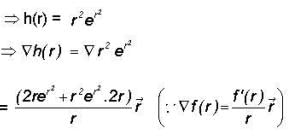
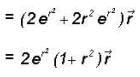
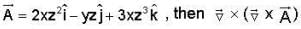 is equal to
is equal to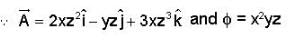
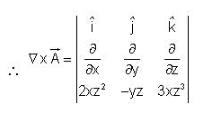
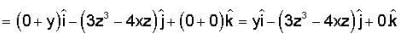
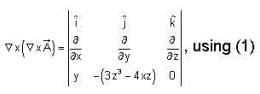
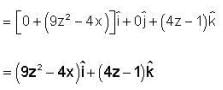
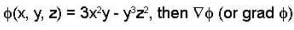 at the p oin t (1, -2, 1), is
at the p oin t (1, -2, 1), is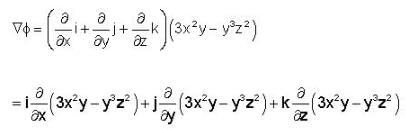
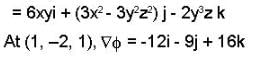
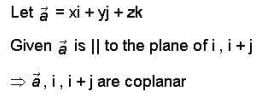
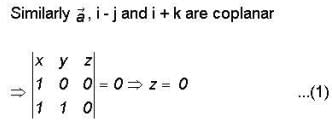
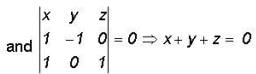
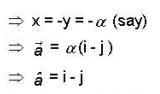
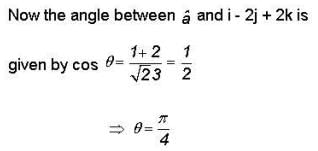
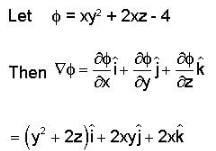
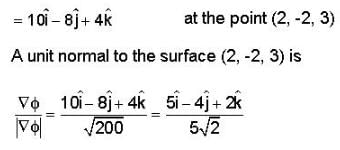
 is given by
is given by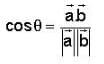
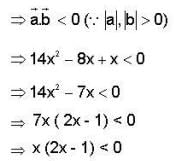
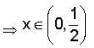
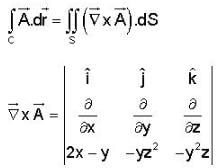
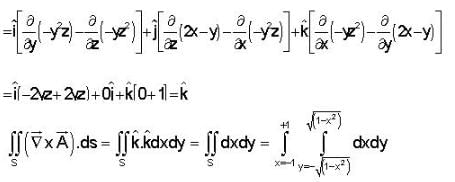
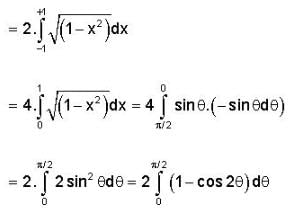
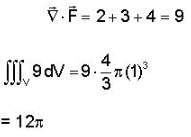
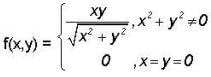 then
then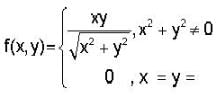
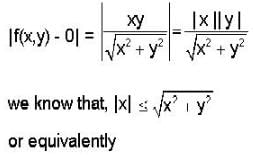
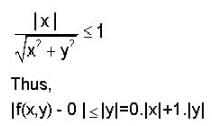
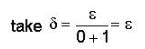
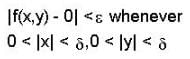
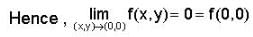
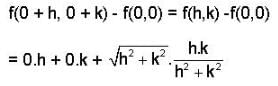
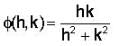
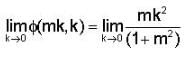
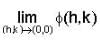 d o e s not exists.Hence,f(x,y) is not
d o e s not exists.Hence,f(x,y) is not 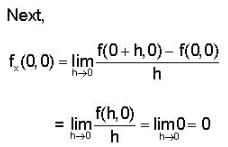
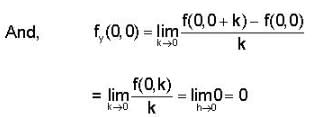
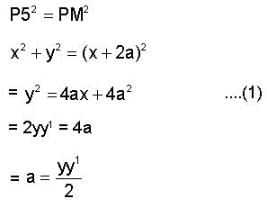
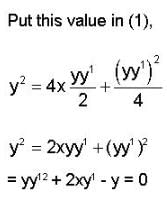
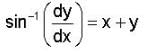 is
is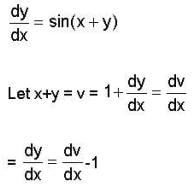
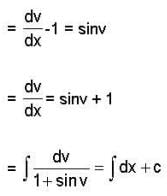
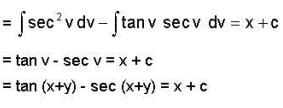
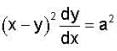 is equal to,
is equal to,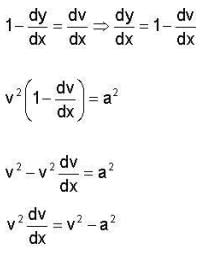
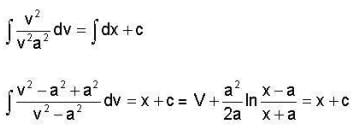
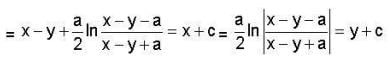
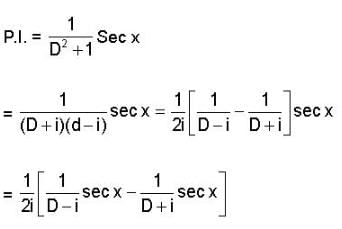
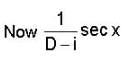
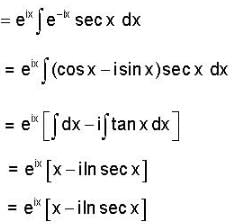
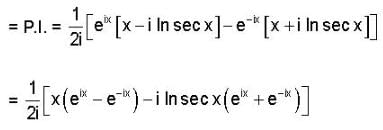
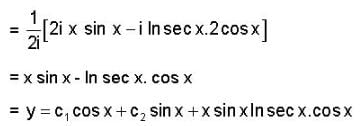
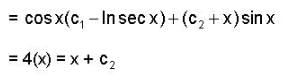
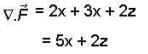
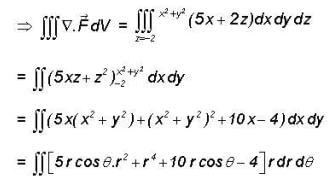
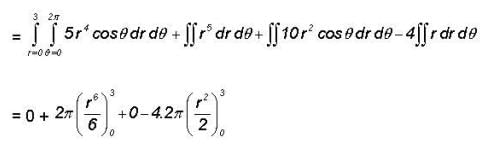
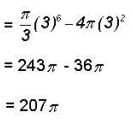
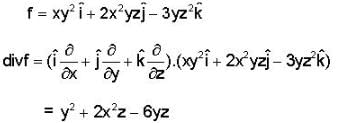
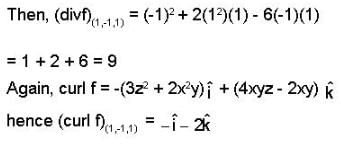
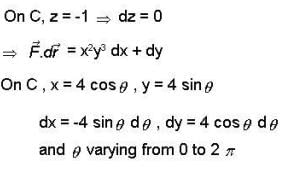
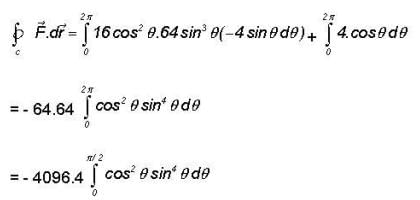
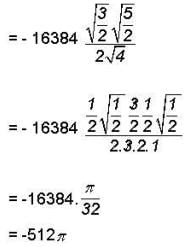
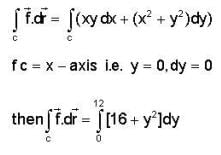
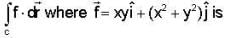
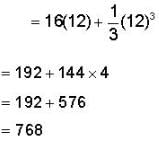
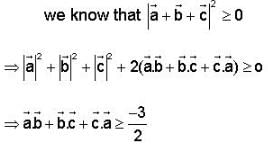
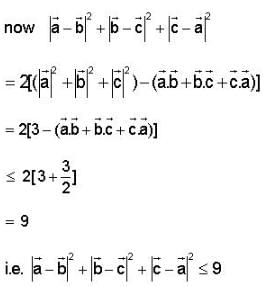
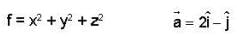
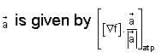
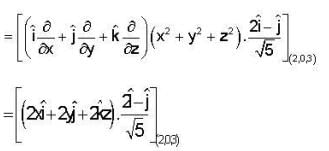

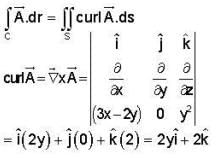
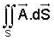 for the vector
for the vector 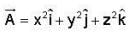 take over
take over