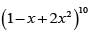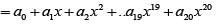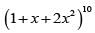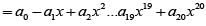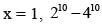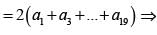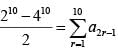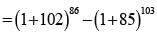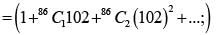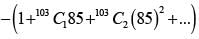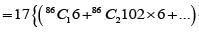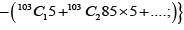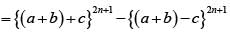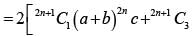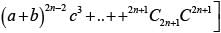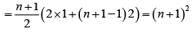JEE Advanced Level Test: Binomial Theorem- 3 - JEE MCQ
30 Questions MCQ Test - JEE Advanced Level Test: Binomial Theorem- 3
Coefficient of x5 in the expansion of 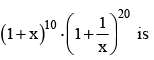
The coefficients of 9th, 10th and 11th terms in the expansion of (1 + x)n are in A.P. then n =
Coefficient of x4 in (1 + x – 2x2)6 is
It is suitable to use Binomial Distribution only for
The number of terms in the expansion of [(a + 4b)3 + (a - 4b)3]2 are
If (1+x+x2)n 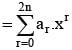 then a1 - 2a2 + 3a3 - ... - 2na2n = ....
then a1 - 2a2 + 3a3 - ... - 2na2n = ....
The sum of the binomial coefficients of the 3rd, 4th terms from the beginning and from the end of (a + x)n is 440 then n =
Let R = (5√5 + 11)2n+1, f = R - [R], then Rf =
I + F when I is odd and 0 < F < 1, then (I + F) (I - F) =
The expansion [x + (x3 - 1)1/2]5 + [x + (x3 - 1)1/2]5 is a polynomial of degree
If t0, t1, t2, ............tn are the consecutive terms in the expansion (x + a)n then (t0 - t2 + t4 - t6 + ....)2 + (t1 - t3 + t5....)2 =
Coefficient of x50 in (1 + x)1000 + 2x(1 + x)999 + 3x2 (1 +x)998 +....is
The coefficient of x9 in (x + 2) (x + 4) (x + 8).....(x + 1024) is
The coefficient of xn in the polynomial (x +n C0) (x + 3.nC1) (x + 5.nC2)...[x + (2n+ 1).nCn] is
If x = (2 +√3)n, n ∈ N and f = x - [x], then 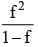
If 22006 - 2006 divided by 7, the remainder is
In a Binomial Distribution, if p, q and n are probability of success, failure and number of trials respectively then variance is given by
The coefficient of the term independent of x in the expansion of 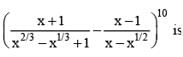
The coefficient of x12 in the expansion of (1+2x2 - x3)8
Coefficient of x11 in the expansion of (1+x2)4 (1+x3)7 (1+x4)12 is
The number of irrational terms in the expansion of (21/5 +31/10)55 is
In the expansion of 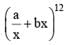 , the coefficient of x-10 will be
, the coefficient of x-10 will be
The middle term in the expansion of (1 – 2x + x2)n is
(103)86 - (86)103 - 86 is divisible by
The number of dissimilar terms in the expansion of (a + b + c)2n+1 – (a + b – c)2n+1 is


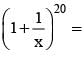

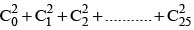 = (where nCr = 25Cr)
= (where nCr = 25Cr)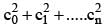
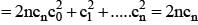
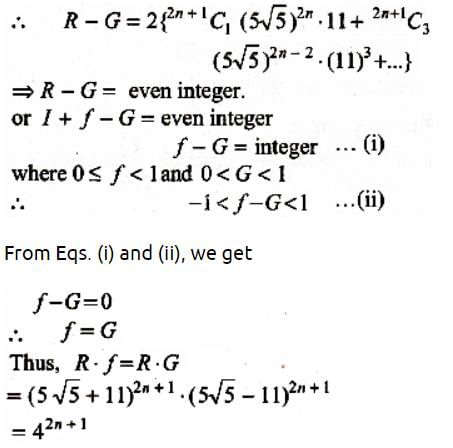
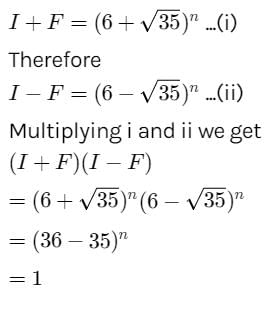
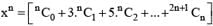
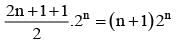
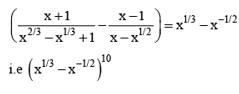
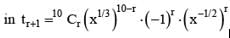
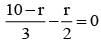
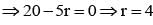
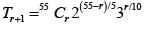
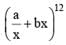
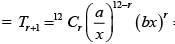
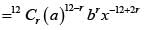
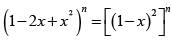
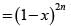
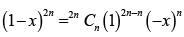
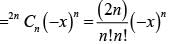
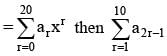 equlas
equlas