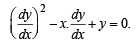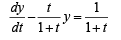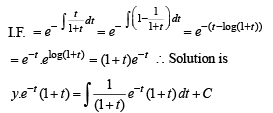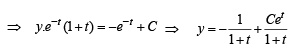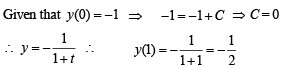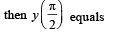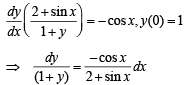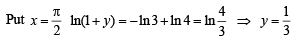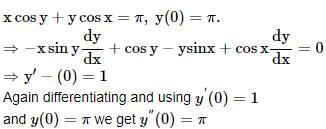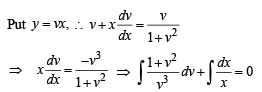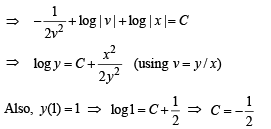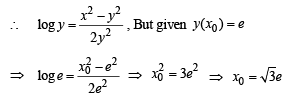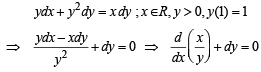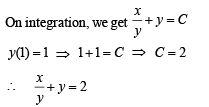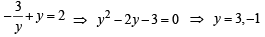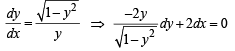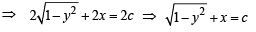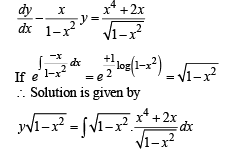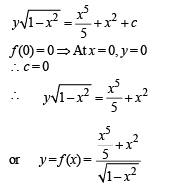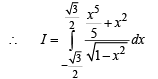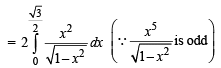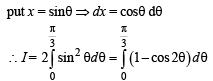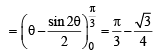JEE Advanced (Single Correct MCQs): Differential Equations - JEE MCQ
Test Description
9 Questions MCQ Test - JEE Advanced (Single Correct MCQs): Differential Equations
JEE Advanced (Single Correct MCQs): Differential Equations for JEE 2025 is part of JEE preparation. The JEE Advanced (Single Correct MCQs): Differential Equations questions and answers have been prepared
according to the JEE exam syllabus.The JEE Advanced (Single Correct MCQs): Differential Equations MCQs are made for JEE 2025 Exam.
Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for JEE Advanced (Single Correct MCQs): Differential Equations below.
Solutions of JEE Advanced (Single Correct MCQs): Differential Equations questions in English are available as part of our course for JEE & JEE Advanced (Single Correct MCQs): Differential Equations solutions in
Hindi for JEE course.
Download more important topics, notes, lectures and mock test series for JEE Exam by signing up for free. Attempt JEE Advanced (Single Correct MCQs): Differential Equations | 9 questions in 10 minutes | Mock test for JEE preparation | Free important questions MCQ to study for JEE Exam | Download free PDF with solutions
JEE Advanced (Single Correct MCQs): Differential Equations - Question 1
A solution of the differential equation
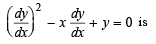
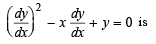
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 1
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 2
JEE Advanced (Single Correct MCQs): Differential Equations - Question 3
If y(t) is a solution of 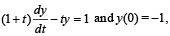 then y(1) is equal to
then y(1) is equal to
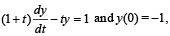 then y(1) is equal to
then y(1) is equal to
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 3
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 4
JEE Advanced (Single Correct MCQs): Differential Equations - Question 5
If y = y(x) and it follows the relation x cos y + y cos x = π then y" (0) =
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 5
JEE Advanced (Single Correct MCQs): Differential Equations - Question 6
The solution of primitive integral equation (x2 + y2) dy = xy dx is y = y(x). If y (1) = 1 and (x0) = e, then x0 is equal to
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 6
JEE Advanced (Single Correct MCQs): Differential Equations - Question 7
For th e primitive in tegral equation ydx + y2dy = x dy; x ∈ R, y > 0, y = y(x), y(1) = 1, then y(–3) is
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 7
JEE Advanced (Single Correct MCQs): Differential Equations - Question 8
The differential equation 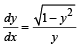 determines a family of circles with
determines a family of circles with
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 8
JEE Advanced (Single Correct MCQs): Differential Equations - Question 9
The function y = f (x) is the solution of the differential equation 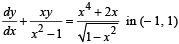 satisfying f (0) = 0. Then
satisfying f (0) = 0. Then 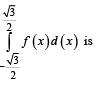
Detailed Solution for JEE Advanced (Single Correct MCQs): Differential Equations - Question 9
Information about JEE Advanced (Single Correct MCQs): Differential Equations Page
In this test you can find the Exam questions for JEE Advanced (Single Correct MCQs): Differential Equations solved & explained in the simplest way possible.
Besides giving Questions and answers for JEE Advanced (Single Correct MCQs): Differential Equations, EduRev gives you an ample number of Online tests for practice
Download as PDF