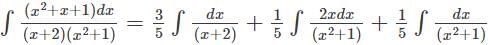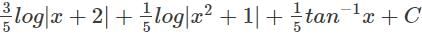Mathematics: CUET Mock Test - 10 - CUET MCQ
30 Questions MCQ Test CUET Mock Test Series - Mathematics: CUET Mock Test - 10
What will be the average rate of change of the function [y = 16 – x2] between x = 3 and x = 4?
For differential equation 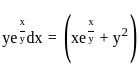 dy, y(0) = 1, the value of y is equal to:
dy, y(0) = 1, the value of y is equal to:
For two events A, B
P(A ∪ B) = 7/12, P(A) = 5/12, P(B) = 3/12 Then P(A ∩ B) =
The probability distribution of X is:
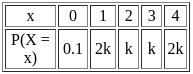
Then var(X) =
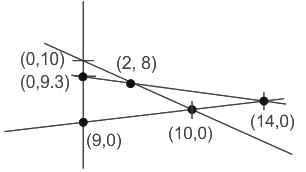
The maximum value of z = 4x + 2y subject to constraints
2x + 3y ≤ 28
x + y ≤ 10
x, y ≥ 0 is
What will be the approximate change in the surface area of a cube of side xm caused by increasing the side by 2%.
What will be the average rate of change of the function [y = 16 – x2] at x = 4?
Find the approximate value of f(4.04), where f(x)=7x3+6x2-4x+3.
What will be the value of the co-ordinate whose position of a particle moving along the parabola y2 = 4x at which the rate at of increase of the abscissa is twice the rate of increase of the ordinate?
The time rate of change of the radius of a sphere is 1/2π. When it’s radius is 5cm, what will be the rate of change of the surface of the sphere with time?
Find the approximate change in the volume of cube of side xm caused by increasing the side by 6%.
|
39 docs|147 tests
|


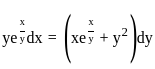
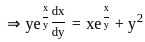
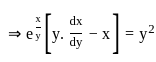
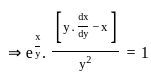 ......(1)
......(1)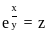
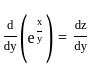
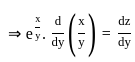
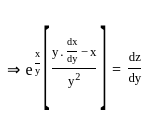 ........(2)
........(2)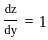
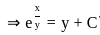

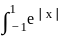 dx =
dx =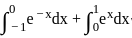
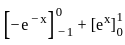
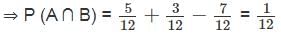

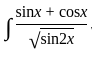
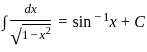
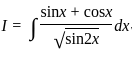 . . .(1)
. . .(1)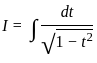
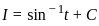
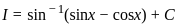
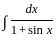 equals
equals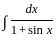
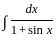 ×
× 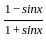 dx
dx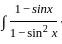 ...(1 - sin2x = cos2x)
...(1 - sin2x = cos2x)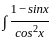 =
= 
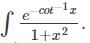
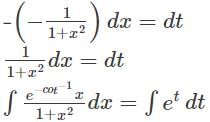
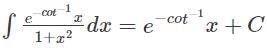
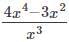 .
.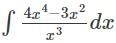
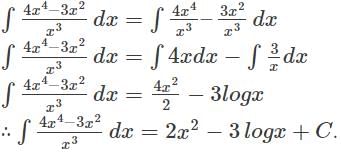
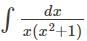 equals ______
equals ______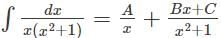
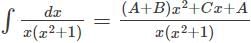
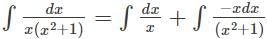
 equals ___
equals ___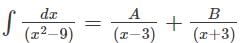
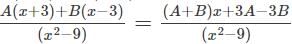
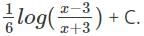
 .
.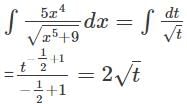
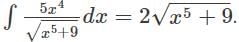
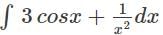
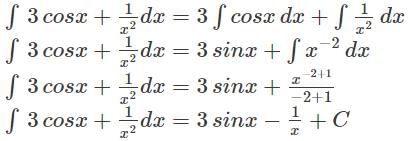
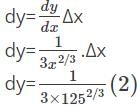
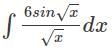
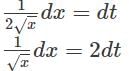
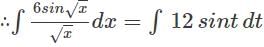
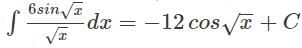
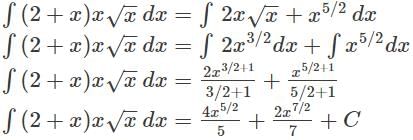
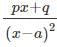 represents?
represents?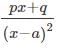 which can also be written as
which can also be written as 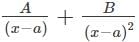 and is further used to solve integration by partial fractions numerical.
and is further used to solve integration by partial fractions numerical.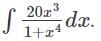
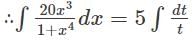
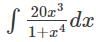 = 5log(1+x4)+C
= 5log(1+x4)+C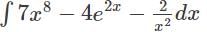
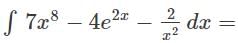
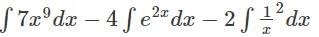
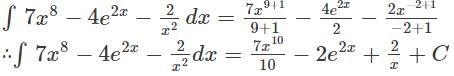
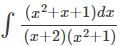 equals ______
equals ______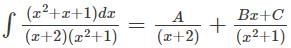
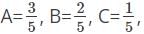 now putting these values in the equation we get,
now putting these values in the equation we get,