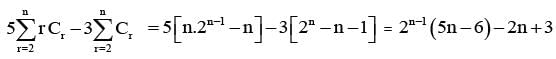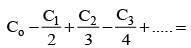Test: Binomial Theorem - 3 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Test: Binomial Theorem - 3
15 buses fly between Hyderabad and Tirupati. The number of ways can a man go to Tirupati from Hyderabad by a bus and return by a different bus is
In a class of 10 students there are 3 girls. The number of ways they can be arranged in a row, so that no two girls are consecutive is k. 8!, where k =
S1, S2, …., S10 are the speakers in a conference. If S1 addresses only after S2, then the number of ways the speakers address is
The total number of 9 digit numbers which have all different digits is
The number of 6 digit numbers in which all the odd digits and only odd digits appear, is
The letters of the word ‘ZENITH’ are permuted in all possible ways and the words thus formed are arranged as in a dictionary. The rank of the word ‘ZENITH’ is
The sum of all the numbers that can be formed by taking all the digits from 2, 3, 4, 5 is
The number of ways in which 6 gentlemen and 3 ladies be seated round a table so that every gentleman may have a lady by his side is
The number of ways in which 7 men be seated at a round table so that two particular men are not side by side is
The letters of the word ‘MADHURI’ are arranged in all possible ways. The number of arrangements in which there are 2 letters between R and H is
The number of ways to arrange the letters of the word ‘GARDEN’ with vowels in alphabetical order is
The number of ways in which 7 Indians and 6 Pakistanis sit around a round table so that no two Indians are together is
Number of ways in which 7 seats around a table can be occupied by 15 persons is
|
27 docs|150 tests
|