Test: Combinations - GMAT MCQ
10 Questions MCQ Test Practice Questions for GMAT - Test: Combinations
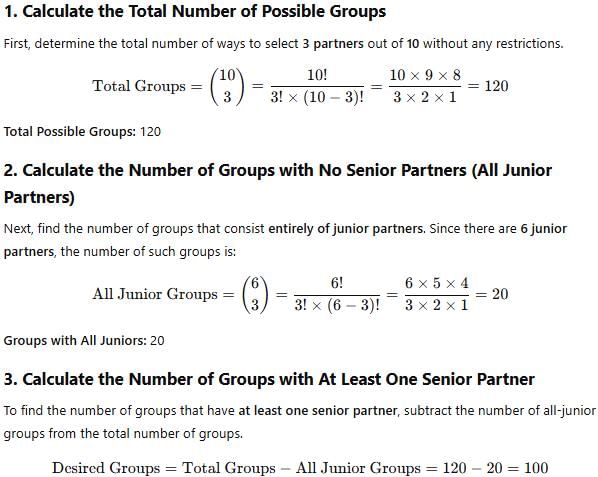
A firm has 4 senior partners and 6 junior partners. How many different groups of 3 partners can be formed in which at least one member of the group is a senior partner. (Two groups are considered different if at least one group member is different)
From a group of 7 men and 6 women, five persons are to be selected to form a committee so that at least 3 men are there on the committee. In how many ways can it be done?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
If two integers x and y such x>y are selected at random between -8 and 11, inclusive, how many cases are possible?
A palindrome is a number that reads the same forward and backward. For example - 1331. How many 4-digit palindromes can be formed using two of the five digits - 1, 3, 5, 7, and 9?
How many arrangements of the letters A, C, C, E, N, T include at least one letter between the two C’s?
A box contains 90 balls of different colours: 13 yellow, 19 green, 27 red, 10 black, 7 brown and 14 white. Find the smallest number V such that any V balls drawn from the box will contain at least 14 balls of the same colour.
Warren has five vehicles: a blue convertible, a black sedan, a red coupe, a silver station wagon, and a blue SUV. If he drives a different car to work each day from Monday through Friday, in how many orders could he drive his five cars during a week if he always drives the convertible on Friday and won’t drive the same color vehicle on consecutive days?
If the Board of Selectmen contains 4 positions, and if in the current election two candidates are running for each position, then in how many different ways can these candidates be elected to the Board?
|
18 docs|139 tests
|
Important Questions for Combinations
Combinations MCQs with Answers
Online Tests for Combinations Practice Questions for GMAT
|
© EduRev
|
Education Revolution
|
|
