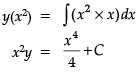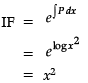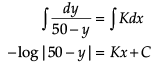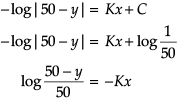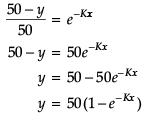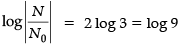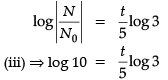Differential Equations- Case Based Type Questions - Free with solutions
MCQ Practice Test & Solutions: Test: Differential Equations- Case Based Type Questions (20 Questions)
You can prepare effectively for JEE Mathematics (Maths) Class 12 with this dedicated MCQ Practice Test (available with solutions) on the important topic of "Test: Differential Equations- Case Based Type Questions". These 20 questions have been designed by the experts with the latest curriculum of JEE 2026, to help you master the concept.
Test Highlights:
- - Format: Multiple Choice Questions (MCQ)
- - Duration: 40 minutes
- - Number of Questions: 20
Sign up on EduRev for free to attempt this test and track your preparation progress.
Direction: Read the following text and answer the following questions on the basis of the same:
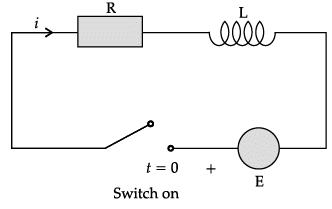
A differential equation of the form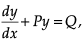 
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.
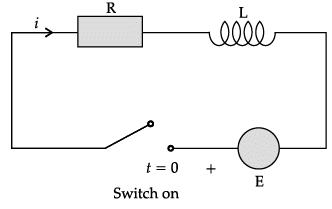
Consider the linear differential equation
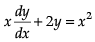
Q. The general solution is _______.
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.Detailed Solution: Question 1
Direction: Read the following text and answer the following questions on the basis of the same:
A differential equation of the form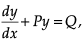 
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.
Consider the linear differential equation
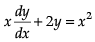
Q. The value of ∫ P dx = ______.
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.Detailed Solution: Question 2
Direction: Read the following text and answer the following questions on the basis of the same:
A differential equation of the form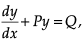 
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.
Consider the linear differential equation
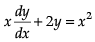
Q. ∫ Qdx = _______.
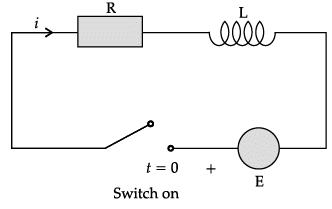
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.Detailed Solution: Question 3
Direction: Read the following text and answer the following questions on the basis of the same:
A differential equation of the form 
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.
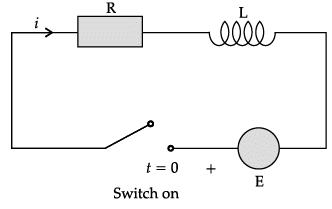
Consider the linear differential equation
Q. The integrating factor is _______.
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.Detailed Solution: Question 4
Direction: Read the following text and answer the following questions on the basis of the same:
A differential equation of the form 
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.
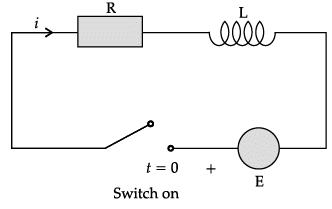
Consider the linear differential equation
Q. If y(1) = 0, then y(2) = _______.
where P and Q are functions of x alone, is called a first order linear differential equation. It has many applications in physics including RL circuits.Detailed Solution: Question 5
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. The value of C in the particular solution given that y(0) = 0 and k = 0.049 is
Detailed Solution: Question 6
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. Which method of solving a differential equation can be used to solve dy/dx = k(50 - y)?
Detailed Solution: Question 7
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. State the order of the above given differential equation.
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. The solution of the differential equation dy/dx = k(50 - y) is given by,
Detailed Solution: Question 9
Direction: Read the following text and answer the following questions on the basis of the same:
Polio drops are delivered to 50K children in a district. The rate at which polio drops are given is directly proportional to the number of children who have not been administered the drops. By the end of 2nd week half the children have been given the polio drops. How many will have been given the drops by the end of 3rd week can be estimated using the solution to the differential equation dy/dx = k(50 - y) where x denotes the number of weeks and y the number of children who have been given the drops.
Q. Which of the following solutions may be used to find the number of children who have been given the polio drops?
Detailed Solution: Question 10
Direction: Read the following text and answer the following questions on the basis of the same:
A Veterinary doctor was examining a sick cat brought by a pet lover. When it was brought to the hospital, it was already dead. The pet lover wanted to find its time of death. He took the temperature of the cat at 11.30 pm which was 94.6°F. He took the temperature again after one hour; the temperature was lower than the first observation. It was 93.4°F. The room in which the cat was put is always at 70°F. The normal temperature of the cat is taken as 98.6°F when it was alive. The doctor estimated the time of death using Newton law of cooling which is governed by the differential equation: dT/dt ∞ (T−70), where 70°F is the room temperature and T is the temperature of the object at time t.
Substituting the two different observations of T and t made, in the solution of the differential equation dT/dt = k(T - 70) where k is a constant of proportion, time of death is calculated.
Q. The solution of the differential equation dT/dt = k(T - 70) is given by,
Direction: Read the following text and answer the following questions on the basis of the same:
A Veterinary doctor was examining a sick cat brought by a pet lover. When it was brought to the hospital, it was already dead. The pet lover wanted to find its time of death. He took the temperature of the cat at 11.30 pm which was 94.6°F. He took the temperature again after one hour; the temperature was lower than the first observation. It was 93.4°F. The room in which the cat was put is always at 70°F. The normal temperature of the cat is taken as 98.6°F when it was alive. The doctor estimated the time of death using Newton law of cooling which is governed by the differential equation: dT/dt ∞ (T−70), where 70°F is the room temperature and T is the temperature of the object at time t.
Substituting the two different observations of T and t made, in the solution of the differential equation dT/dt = k(T - 70) where k is a constant of proportion, time of death is calculated.
Q. Which method of solving a differential equation helped in calculation of the time of death?
Direction: Read the following text and answer the following questions on the basis of the same:
A Veterinary doctor was examining a sick cat brought by a pet lover. When it was brought to the hospital, it was already dead. The pet lover wanted to find its time of death. He took the temperature of the cat at 11.30 pm which was 94.6°F. He took the temperature again after one hour; the temperature was lower than the first observation. It was 93.4°F. The room in which the cat was put is always at 70°F. The normal temperature of the cat is taken as 98.6°F when it was alive. The doctor estimated the time of death using Newton law of cooling which is governed by the differential equation: dT/dt ∞ (T−70), where 70°F is the room temperature and T is the temperature of the object at time t.
Substituting the two different observations of T and t made, in the solution of the differential equation dT/dt = k(T - 70) where k is a constant of proportion, time of death is calculated.
Q. What will be the degree of the above given differential equation.
Direction: Read the following text and answer the following questions on the basis of the same:
A Veterinary doctor was examining a sick cat brought by a pet lover. When it was brought to the hospital, it was already dead. The pet lover wanted to find its time of death. He took the temperature of the cat at 11.30 pm which was 94.6°F. He took the temperature again after one hour; the temperature was lower than the first observation. It was 93.4°F. The room in which the cat was put is always at 70°F. The normal temperature of the cat is taken as 98.6°F when it was alive. The doctor estimated the time of death using Newton law of cooling which is governed by the differential equation: dT/dt ∞ (T−70), where 70°F is the room temperature and T is the temperature of the object at time t.
Substituting the two different observations of T and t made, in the solution of the differential equation dT/dt = k(T - 70) where k is a constant of proportion, time of death is calculated.
Q. If the temperature was measured 2 hours after 11.30 pm, what will be the change in time of death?
Direction: Read the following text and answer the following questions on the basis of the same:
A Veterinary doctor was examining a sick cat brought by a pet lover. When it was brought to the hospital, it was already dead. The pet lover wanted to find its time of death. He took the temperature of the cat at 11.30 pm which was 94.6°F. He took the temperature again after one hour; the temperature was lower than the first observation. It was 93.4°F. The room in which the cat was put is always at 70°F. The normal temperature of the cat is taken as 98.6°F when it was alive. The doctor estimated the time of death using Newton law of cooling which is governed by the differential equation: dT/dt ∞ (T−70), where 70°F is the room temperature and T is the temperature of the object at time t.
Substituting the two different observations of T and t made, in the solution of the differential equation dT/dt = k(T - 70) where k is a constant of proportion, time of death is calculated.
Q. If t = 0 when T is 72, then the value of C is
Direction: Read the following text and answer the following questions on the basis of the same:
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given that the number triples in 5 hours.
Q. If N0 is the initial count of bacteria, after 10 hours the count is _______.
Detailed Solution: Question 16
….(ii)
Direction: Read the following text and answer the following questions on the basis of the same:
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given that the number triples in 5 hours.
Q. If ‘N’ is the number of bacteria, the corresponding differential equation is _______.
Detailed Solution: Question 17
Direction: Read the following text and answer the following questions on the basis of the same:
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given that the number triples in 5 hours.
Q. 
Detailed Solution: Question 18
Direction: Read the following text and answer the following questions on the basis of the same:
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given that the number triples in 5 hours.
Q. The general solution is _______.
Detailed Solution: Question 19
Direction: Read the following text and answer the following questions on the basis of the same:
The rate of increase in the number of bacteria in a certain bacteria culture is proportional to the number present. Given that the number triples in 5 hours.
Q. The bacteria becomes 10 times in _______ hours.
Detailed Solution: Question 20
61 videos|239 docs|58 tests |
