Test: Fractions/Ratios/Decimals - GMAT MCQ
10 Questions MCQ Test - Test: Fractions/Ratios/Decimals
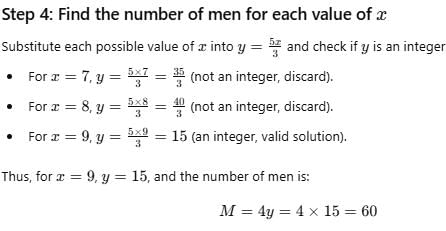
The ratio of women to children at a certain party is 2: 5 and the ratio of children to men is 3:4. If there are more than 13 and less than 20 women at the party, what is the number of men at the party?
The ratio of cars to trucks in your toy box is 5 to 2. After you lose two cars, you buy a pack of eight trucks. The ratio of cars to trucks in the toy box after these changes is 3 to 2. How many trucks did you have originally ?
Which of the following fractions is equal to the decimal 0.0625 ?
In a certain game, each player scores either 2 points or 5 points. If n players score 2 points and m players score 5 points, and the total number of points scored is 50, what is the least possible positive difference between n and m?
Two libraries are planning to combine a portion of their collections in one new space. The new space will house 1/3 of the books from Library A, along with 1/4 of the books from Library B. If there are twice as many books in Library B as in Library A, what proportion of the books in the new space will have come from Library A?
A pool which was 2/3 full to begin with, was filled at a constant rate for 5/3 hours until it was until it was 6/7 full. At this rate, how much time would it take to completely fill this pool if it was empty to begin with?
A school library consists of literature books and science books in the ratio of 5:6. If 50 more literature books are added to the library and the number of science books is increased by 1/4th of the existing science books, the ratio of the literature books and science books increases to 4:3. If the library consists of only literature and the science books, what is the initial number of books in the library?
An assembly line produces 500 vehicle parts daily. The line produces only car parts and truck parts in a ratio of 2 : 3 and the ratio of defective car parts to defective truck parts is 1 : 2. If 6 parts on a certain day are defective, how many non-defective car parts are produced on that day?
A bag contains blue, red and green marbles only. If the ratio of blue marbles to red marbles is 4 to 5, and the ratio of green marbles to blue marbles is 4 to 3, what is the minimum number of marbles in the bag?
A photographer purchases 30 rolls of black and white film and 25 rolls of color film. If the cost of each roll of color film is twice of the cost of each roll of black and white film, what percent of the total cost of the film is the price of a roll of color film?