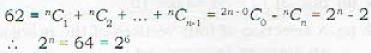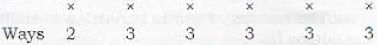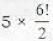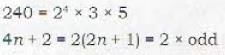Test:- Permutations And Combinations - 11 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Test:- Permutations And Combinations - 11
In a club election the number contestants is one more than the number of maximum candidates for which a voter can vote. If the total number of ways in which a voter can vote be 62 then the number of candidates is
The total number of selections of at most n things from (2n + 1) different things is 63. Then the value of n is
Let 1 ≤ m < n ≤ p. The number of subsets of the set A - {1, 2, 3, p} having m, n as the least and the greatest elemens respectively, is
The number of ways in which n different prizes can be distributed among m(< n) persons if each is entitled to receive at most n - 1 prizes, is
The number of possible outcomes in a throw of n ordinary dice in which at least one of the dice shown an odd number is
The number of different 6 digit numbers that can be formed using the three digits 0, 1 and 2 is
The number of different matrices that can be formed with elements 0, 1, 2 or 3, each matrix having 4 elements, is
Let A be a set of (n ≥ 3) distinct elements. The number of triplets (x, y, z) of the elements of .4 in which at. least two coordinates are equal is
The number of different pairs of words  that can be made with the letters of the wrord STATICS is
that can be made with the letters of the wrord STATICS is
Total number of 6-digit number in which all the odd digits and only odd digits appear, is
The number of divisors of the form 4n + 2(n ≥ 0) of the integer 240 is
In the next World Cup of cricket there will be 12 teams, divided equally in two groups. Teams of each group will play a match against each other. From each group 3 top teams will qualify for the next round. In this round, each team will play against others once. Four top teams of this round will qualify for the semifinal round, where each team will play against the others once. Two top teams of this round will go to the final round, where they will play the best of three matches. The minimum number of matches in the next World Cup will be
The number of different ways in which 8 persons can stand in a row so that between two particular persons A and B there are always two persons, is
Four couples (husband and wife) decide to form a committee of four members. The number of different committees that can be formed in which no couple finds a place is
From 4 gentlemen and 6 ladies a committee of five is to be selected. The number of ways in which the committee can be formed so that gentlemen are in majority is
There are 20 questions in a question paper. If no two students solve the same combination of questions but solve equal number of questions then the maximum number of students who appeared in the examination is
Nine hundred distinct n-digit positive numbers are to be formed using only the digits 2, 5 and 7. The smallest value of n for which this is possible is
The total number of integral solutions for (x, y, z) such that xyz = 24 is
The number of ways in which the- letters of the word ARTICLE can be rearranged so that the even places are always occupied by consonants is
A cabinet of ministers consists of 11 ministers, one minister being the chief minister. A meeting is to be held in a room having a round table and 11 chairs round it, one of them being meant for the chairman. The number of ways in which the minister can take their chairs, the chief minister occupying the chairman's place, is
|
27 docs|150 tests
|