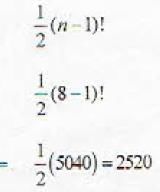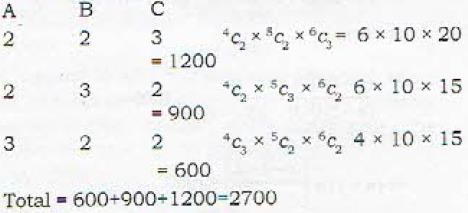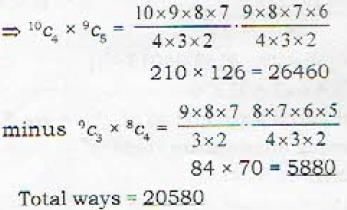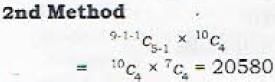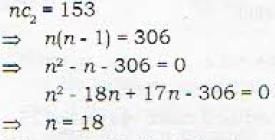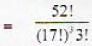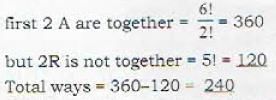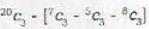Test:- Permutations And Combinations - 5 - Mathematics MCQ

To fill 12 vacancies there are 25 candidates of which 5 are from scheduled casts. If 3 of the vacancies are reserved for scheduled casts candidates while the rest are open to all. The number of ways in which the selections can be made is :

A businessman hosts a dinner to 21 guests. He is having 2 round tables which can accommondate 15 and 6 persons. How many ways are there to host dinner?

In how many ways can 8 men will be seated around a round table when in no two ways a man has the same neighbors?

A question paper consists of two sections having respectively 3 and 4 questions. The following note is given on the paper. “It is not necessary to attempt all the questions one question from each section is compulsory'. “In how' many ways the candidate can select the 5 questions?

 A candidate is required to answer 7 out of 15 question which are divided into three groups each containing 4,5,6 question respectively. He is required to select at least 2 questions from each group. In how many ways can he make up his choice?

 A letter lock consists of three rings each marked with 12 different letters. In how many ways it is possible to make an unsuccessful attempt to open the lock.

The number of ways of choosing a committee of 4 women and 5 men from 10 women and 9 men, If Mr. A refuse to serve on committee, if Mr. B is a member of the committee, can not exeed.

In a football championship, 153 matches were played. Every team played one match with each other. The number of teams participating in the championship is:

The number of ways in which 52 cards can be divided into 4 set, three of them having 17 cards each and the fourth one having just one cards is:

In how many ways can the letters of the word ‘ARRANGE’ be arranged so that the two A's are together but not two R’s is-

In an examination there are three multiple questions and each question has 4 choice. Number of ways in which a student can fail to get all answer correct is-

 There are three straight lines which are parallel and lie in the same plane A total number of 5 point on the first, 7 points on the second, mid 8 points on the third straight line as selected. The maximum number of triangles formed with the vertices at these points are :

The number of ways in which a team of eleven players can be selected from 22 players including 2 of them and excluding 4 of them is:

Seven women and seven men are to sit round a circular table such that there is a man on either side of every women; the number of seating arrangements is :

The number of ways in which four letters can be selected from the word ‘RAMANEA’ is 

The number of ways in which seven persons can be arranged at a round table if two particular persons may not seat together is :

The number of ways in which 4 boys and 4 girls can form a line, with boys and girls alternating, is :