Test: Probability and Statistics - 7 - Mathematics MCQ

In an election, candidate A has 4 chance of winning B has 3 chance, C has, 2 chance and D has, 1 chance. Just before the election C withdraws. The chances of other three candidates are

An anti-aircraft gun can take a maximum of four shots at a plane moving away from it. The probability of hitting the plane in the first, second, third and fourth shot are 0.4, 0.3, 0.2 and 0.1 respectively. What is the probabilities that the gun hits the plane?

If A and B are two independent events in a given sample space and the probability that both A and B occur is 0.16 while the probability that neither occurs is 0.36 the probabilities P[A) and P[B) are respectively

A, B and C are independent events such that P(A) = 0.2, P(B) - 0.1 and P(C) = 0.4, then P(A E BE C) equals

Missiles are fired at a target. The probability of each missile hitting the targes is p : the hits are independent of one another. Each missile which hits the target brings it down with probability r. The missiles are fired until the target is brought down or the missile reserve is exhausted. the reserve consists of n missiles (n>2). Defining the event A as at least one missile will remain in reserve", the probability P(A) is:

For the events A and B to be independent, the probability that both occur is 1/6 and probability that neither of them occur is 1/3. Then the probability of occurrence of A is

For n independent events A₁, A₂, ...., A_n, let P(A_i) = 1/(i+1), i = 1, 2 ..., n. Then, the probability that none of the events will occur is

Suppose the random variable X has the density function

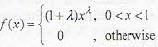
The maximum likelihood estimate of λ based on a given random sample X₁ = x₁,X₂ = x₂,...,x_n = x_n is

Suppose an interactive computer system is proposed for which it is estimated that the mean response time E(T) = 0.5 sec and standard deviation σ = 0.1 sec. Using Chebyshev's inequality, the probability P[|T-0.5| ≥ 0.25] is

X is a normal variate with μ and σ². The value of E(e^X) is

A and B throw a die in succession to win a bet with A starting first. Whoever throws '1' first wins an amount of Rs. 110. What are the respective expectations of A and B?

For a binomial distribution n = 40 and P = 0.05. The variance for the data is :

For a distribution   variance = 100. The coefficient of variation is:

For Poisson variate P(x = 1) = P(x = 2). The mean of a x is :

The mean of a class is 50 and variance is 9. Then what is the standard error of the marks?

If 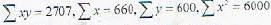 then find the coefficient of correlation.

If variance is x. If each x term is increased by w, then the new variance is: