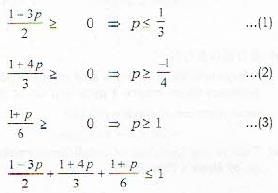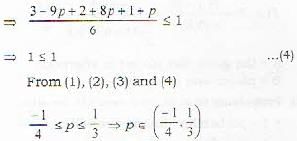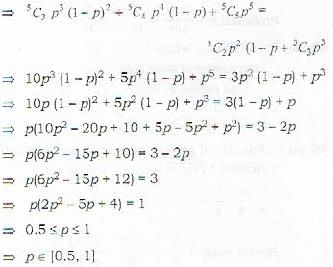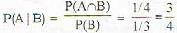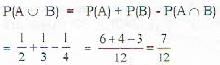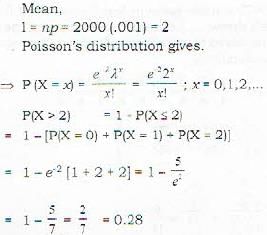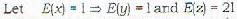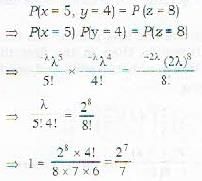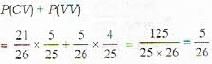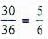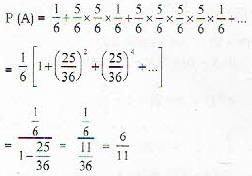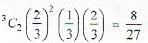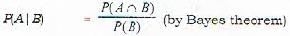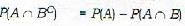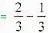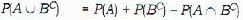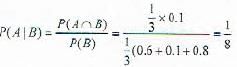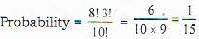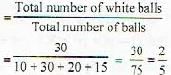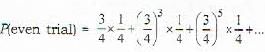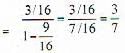Test: Probability and Statistics - 8 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Test: Probability and Statistics - 8
Let A, B and C be independent and mutually exclusive events with probability of occurrences 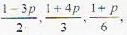 respectively, then p lies in
respectively, then p lies in
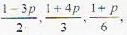 respectively, then p lies in
respectively, then p lies inA communication system consists of n components components. Each of these functions independently with probability p. The system function correctly if and only if at least half of its components functions. For what range of p, the probability that a five-components system functions correctly is higher than the probability that a three-components system functions correctly?
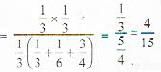
The probability of getting a defective floppy in three boxes A, 13 and C arc 1/3, 1/6 and 3/4, respectively. A box is selected randomly and a floppy is drawn from it. The probability that the floppy is defective and is drawn from box A is
Let A and B be two events with P(A) = 1 /2, P(B) = 1 /3 and P(A ∩ B) = 1/4, What is P(A|B)?
Let A and B be two events with P(A) =1/2, P(B) = 1/3 and P (A ∩ B) = 1/4, What is P (A ∪ B)?
If the probability of a bad reaction from a certain injection is 0.001 then what is the chance that out of 2000 individuals more than two will get a bad injection?
Let X, Y, Z be independent Poisson variables, such that E(X) = E(Y) and E[Z) = 2E(X). If P[X- 5, y = 4) is equal to P[Z = 8), then E(X) is
Two letters are chosen one after another without replacement from the English alphabet. What is the probability that the second letter chosen is a vowel?
A cow is tied with a pole by a 100 meter long rope. What is the probability that at some point of time the cow is at least 60 meters away from the pole?
If two dice, are tossed the probability of getting the sum at least 5 is
A and B play a game of dice. A throws che die first. The person who first gets a 6 is the winner. What is the probability that A wins?
Two teams A and B play a series of four matches. If the probability that team A wins a match is 2/3, then the probability that team A wins three matches, loses one and the third win occurs in the fourth match is
A fair coin is tossed twice. Let A be the event that at least one tail appears and B be the event that both head and tail appear. Then P[A/B], the probability of A given B, is
Let A and B be events with P(A) = 2/3, = P(B) = 1/2 and P(A∩B) = 1/3. Match Lists 1 and II and select the correct answer:

Total number of ways in which four boys and three girls can sit in a row so that boys and girls have alternate seats is
A player is going to play a match either in the morning or in the afternoon or in the evening all possibilities being equally likely. The probability that he wins the match is 0.6, 0.1 and 0.8 according as if the match is played in the morning, afternoon or in the evening respectively. Given that he has won the match, the probability that the match was played in the afternoon is
The probabilities that a husband and wife will be alive 20 years from now are given by 0.8 and 0.9 respectively. What is the probability that in 20 years at least one, will be alive?
A shelf has 6 mathematics books and 4 physics books. The probability that 3 particular mathematics books will be together is?
A marble is drawn at random from a box containing 10 red, 30 white, 20 blue and 15 orange marbles. What is the probability of the ball is white?
Three persons play a game by tossing a fair coin each independently. The game ends in a trial if all of them get the same outcome in that trial, otherwise they continue to the next trial. What is the probability that the game ends in an even number of trials?
|
27 docs|150 tests
|