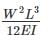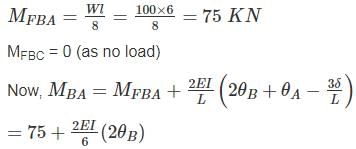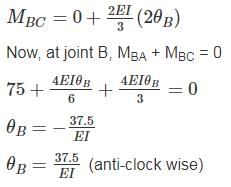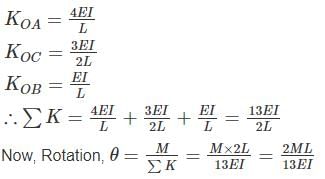Test: Structural Analysis- 1 - Civil Engineering (CE) MCQ
10 Questions MCQ Test - Test: Structural Analysis- 1
Match List - I (Method) with List-II (Factors) and select the correct answer using the codes given below the lists:
List - I
A. Moment distribution
B. Slope deflection
C. Kani’s method
D. Force method
List - II
1. Rotation factor
2. Flexibility
3. Hardy Cross
4. Displacements
5. Stiffness matrix
In the virtual work method of plastic analysis of steel structure, the virtual quantity is
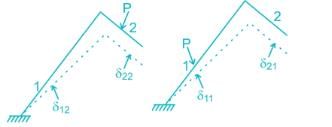
The figure below shows the displacement caused by load P at two points 1 and 2 respectively. According to Maxwell reciprocal theorem which option is CORRECT.

A fixed beam AB is subjected to a triangular load varying from zero at end A to ‘w’ per unit length at end B. The ratio of fixed end moment at B to A will be
Find the carry over moment at support B, in the beam shown with internal hinges at C & D:-
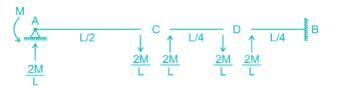
The strain energy stored (U) in the cantilever beam shown is –
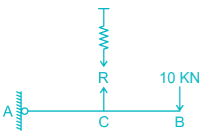
Find the vertical displacement of joint B if the spring constant k = 1 KN/mm and the rigid beam is loaded as shown below:-
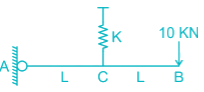
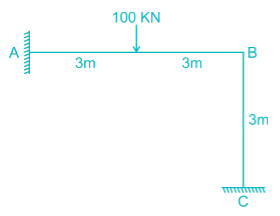
Find the rotation of joint B if the frame is loaded as shown below:
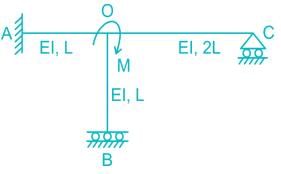
All members of the frame shown below have equal flexural rigidity EI. Calculate the rotation of joint O if moment M is applied?


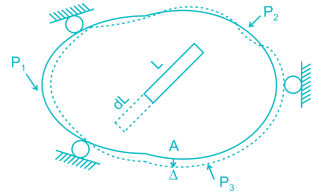
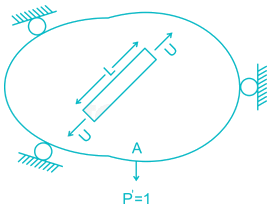



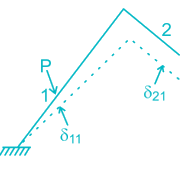
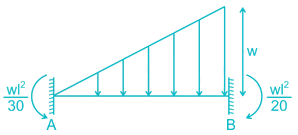
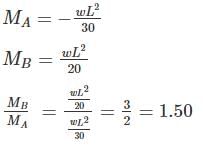
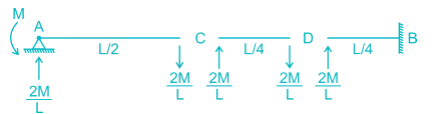

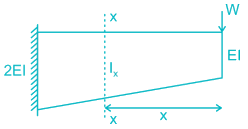
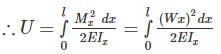

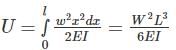

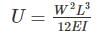

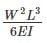 but greater than
but greater than