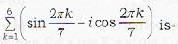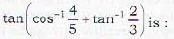Test : Trigonometric Functions And Identities - 7 - Mathematics MCQ
20 Questions MCQ Test Topic-wise Tests & Solved Examples for Mathematics - Test : Trigonometric Functions And Identities - 7
A 6-ft-tall man finds that the angle of elevatior of the top of a 24-ft-high pillar and The angle of depression of its base are complementary angles. The distance of the man from the pillar is
A rocket of height h meters is fired vertically upwards. Its velocity at time t seconds is (2t + 3) meters/second. If the angle of elevation of the top of the rocket from a point on the ground after 1 second of firing is π/6 and after 3 seconds it is π/3 then the distance of the point from the rocket is
Three vertical poles of heighis h1 , h2, and h3 at the vertices A, B and C of a ΔABC subtend angles α, β and γ respectively at the circumcenter of the triangle. If cot α, cot β and cot γ are in AP then h1 , h2, and h3 in
The angle of elevation of the top of a hill from each of the vertices A,B,C of horizontal triangle is α. The heights of the hill is
A piece of a paper in the shape of a sector of a circle of radius 10 cm and of angle 216° just covers the lateral surface of a right circular cone of vertical angle 2θ. Then sinθ is
The angle of elevation of the lop of a vertical pole when observed from each vertex of a regular hexagon is π/3. If the area of the circle circumscribing the hexagon be A metre2 then the area of the hexagon is
A vertical pole PO is standing at the centre O of a square ABCD. If AC subtends an angle 90° at the top, p, of the pole then the angle subtended by a side of the square at P is
A vertical lamp-post of height 9 meters stands at the corner of a rectangular field. The angle of elevation of its top from the farthest corner is 30°. while from another corner it is 45°. The area of the field is
A vertical lamp-post, 6 m high, stands at a distance of 2 m from a wall, 4 m high. A 105-m-tall man starts to walk away from the wall on the other side of the wall, in line with the lamp- post. The maximum distance to which the man can walk remaining in the shadow is
A circular ring of radius 3 cm is suspended horizontally from a point 4 cm vertically above the centre by 4 strings attached at equal intervals to its circumference. If the angle between two consecutive strings be θ then cos θ is
In a triangle, the length of the two larger sides are 10 & 9 respectively. If the angles are in A.P., then the length of the third side can be:
The smallest positive root of the equation tan x - x = 0 lies in
The set of all x in interval |0, π| for which 2sin2x - 3sin x + 1 ≥ 0 is, ..............
The sides of triangle inscribed in a given circle subtend angles α, β and γ at the centre. The minimum value of the arithmetic mean of 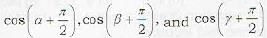 is equal to ...........
is equal to ...........
The set of all x for which ln ( l + x) ≤ x is equal to ..................
The number of all possible triplets (a1, a2, a3) such that a1 + a2 cos2x + a3 sin2x =0 for all x is:
The value of the expression √3 cosec 20° - sec 20° is equal to
If P = sin2θ, + cos4θ, then for all real values of θ:
|
27 docs|150 tests
|