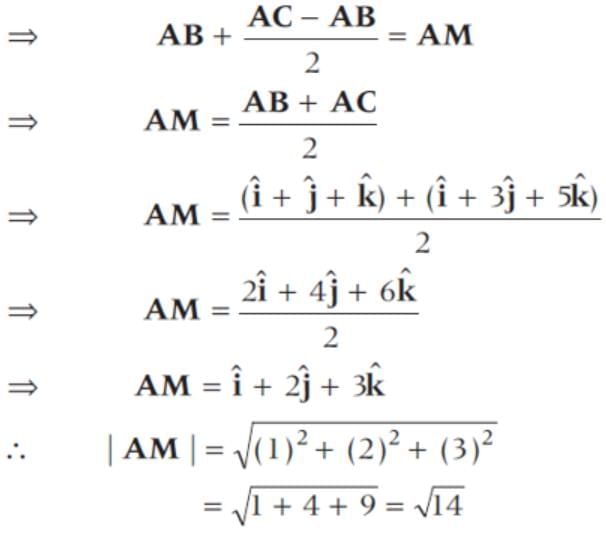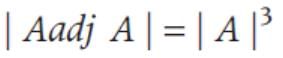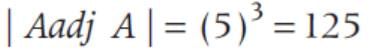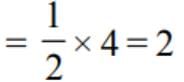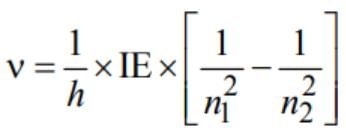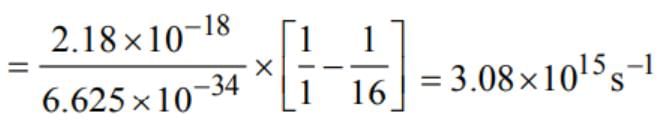UGEE SUPR Mock Test-1 - JEE MCQ
30 Questions MCQ Test UGEE Mock Test Series 2025 - UGEE SUPR Mock Test-1
If the forward voltage in a semiconductor diode is changed from 0.5 V to 0.7 V, then the forward current changes by 1.0 mA. The forward resistance of diode junction will be
The heat generated in a circuit is given by Q = I2 Rt, where I is current, R is resistance andt is time. If the percentage errors in measuring I, R and t are 2%, 1% and 1% respectively, thenthe maximum error in measuring heat will be
The r.m.s. velocity of oxygen molecule at 16°C is 474 m/sec. The r.m.s. velocity in m/s of hydrogen molecule at 127°C is
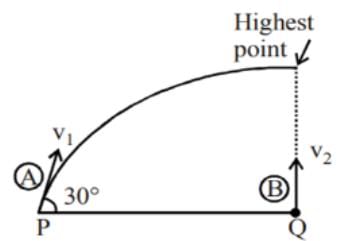
A projectile A is thrown at an angle of 30° to the horizontal from point P. At the same time, another projectile B is thrown with velocity v2 upwards from the point Q vertically below the highest point. For B to collide with A, v2/v1 should be
A boy pushes a toy box 2.0 m along the floor by means of a force of 10 N directed downward at an angle of 60° to the horizontal. The work done by the boy is
The engine ofa truck moving along a straight road delivers constant power. The distance travelled by the truck in time t is proportional to
The escape velocity from a planet is ve. A tunnel is dug along a diameter of the planet and a small body is dropped into it at the surface. When the body reaches the centre of the planet, its speed will be
In an adiabatic process, the pressure is increased by 2/3%. If γ = 3/2 then the volume decreases by nearly
Let x + y = 3 - cos 4θ and x - y = 4 sin2θ then the greatest of xy is
The length of the latus rectum of the parabola which has focus at (-1, 1) and the directrix is 4x
A student read common difference ofan A. P as - 2 instead of 2 and got the sum of first 5 terms as -5. Actual sum of first five terms is
If the side of a cube is increased by 5%, then the surface area of a cube is increased by
The area of the region bounded by the curve y2 = 8x and the line y = 2x is
The general solution of the differential equation x2dy - 2xydx = x4 cos xdx is
The area of the region bounded by the line y = 2x + 1, X-axis and the ordinates x = -1 and x = 1 is
The two vector 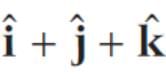 and
and 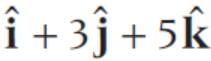 represent the two sides
represent the two sides 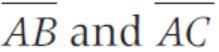 respectively of a ΔABC. The length of the median through A is
respectively of a ΔABC. The length of the median through A is
If A is a square matrix of order 3 and |A| = 5, then |A adj. A| is
A solution of NH4Cl and NH3 has 8.0. Which of the following hydroxides may be precipitated when this solution is mixed with equal volume of 0.2 M of metal ion.
Which of the following is not a disproportionation reaction?
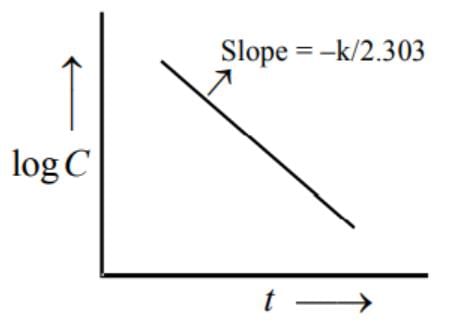
In a 1st order reaction, reactant concentration C varies with time t as:
Which of the following has the highest pπ - pπ bonding tendency ?
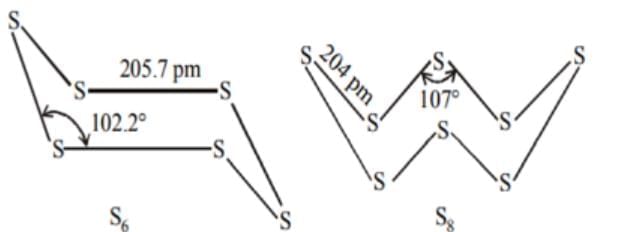
Which of the following set contains species having same angle around the central atom?
Note: Ignore Lone pair bond pair repulsion
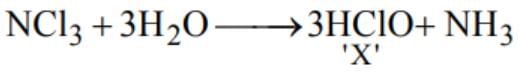
Hydrolysis of NCl3 gives NH3 and X. Which ofthe following is X ?
Element 'B' forms ccp structure and 'A' occupies half of the octahedral voids, while oxygen atoms occupy all the tetrahedral voids. The structure of bimetallic oxide is :
The frequency of radiation emitted when the electron falls from n = 4 to n = 1 in a hydrogen atom will be (Given : ionization energy of H = 2.18 × 10-18J atom-1 land h = 6.625 × 10-34 Js)
|
12 tests
|


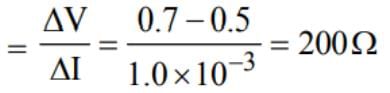
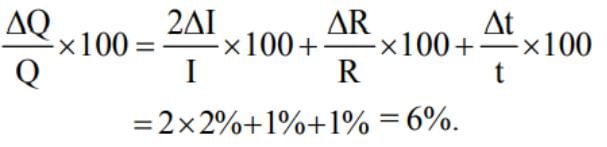

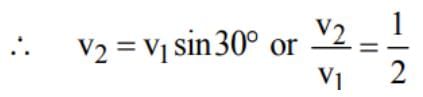
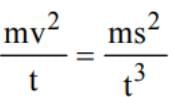
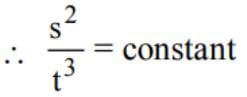
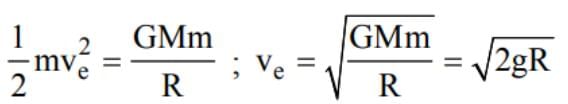
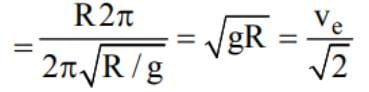
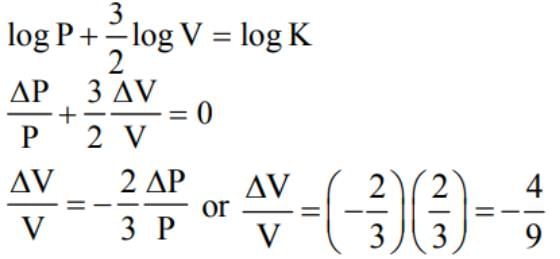
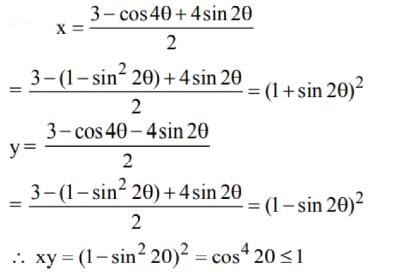
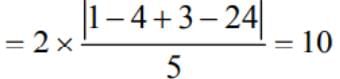
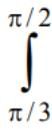 x sin (π[x] -x) dx is equal to:
x sin (π[x] -x) dx is equal to: 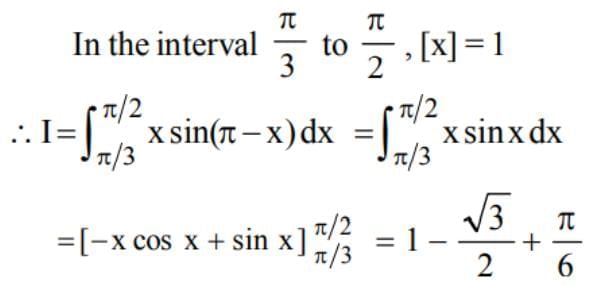
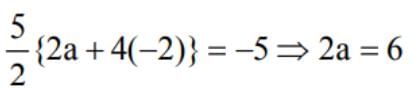
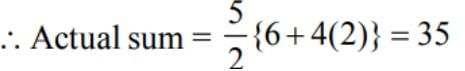
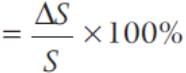
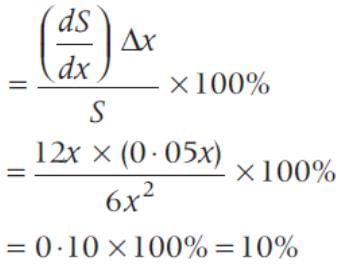
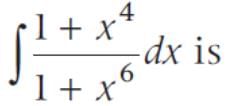
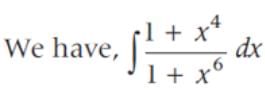
 sin2 x dx is
sin2 x dx is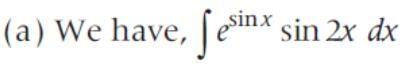
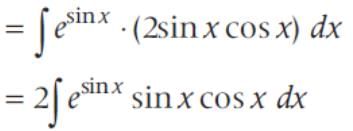
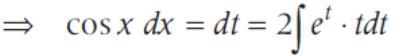
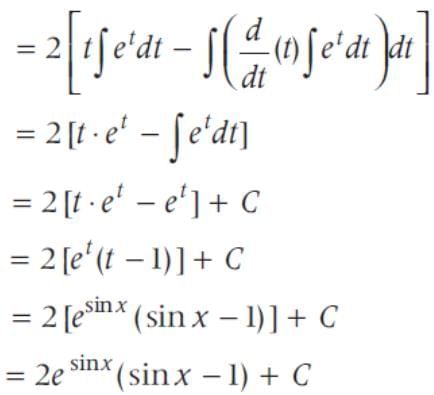
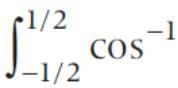 x dx is
x dx is 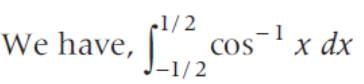
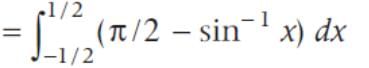
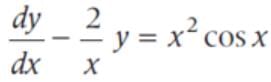
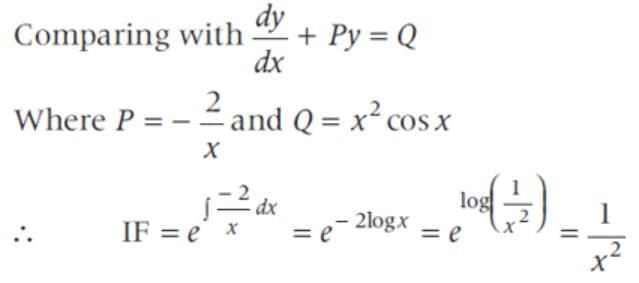
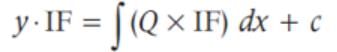
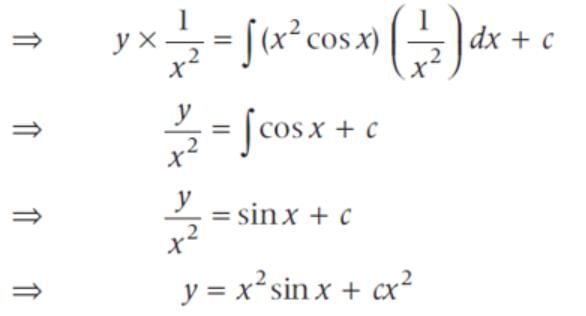
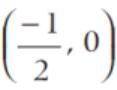 and (0, 1).
and (0, 1).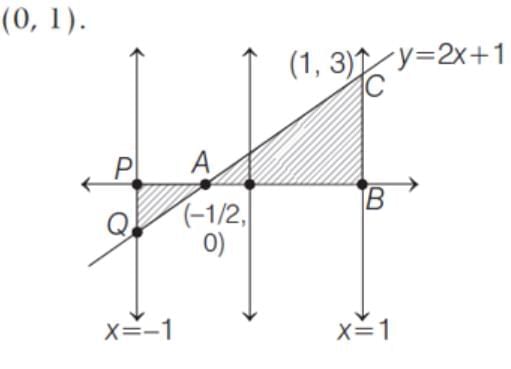
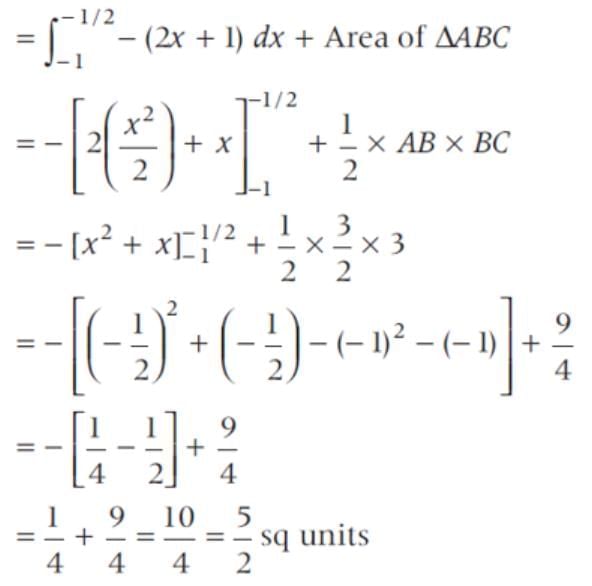
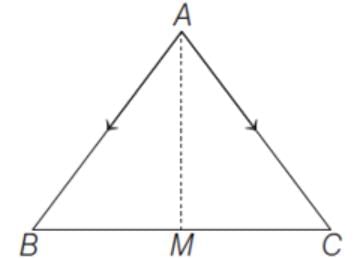
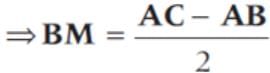 (since, M is a mid-point of BC)
(since, M is a mid-point of BC)