Test: Distance Of A Point From A Plane - JEE MCQ
10 Questions MCQ Test Mathematics (Maths) Class 12 - Test: Distance Of A Point From A Plane
Equation of a plane which is at a distance d from the origin and the direction cosines of the normal to the plane are l, m, n is.
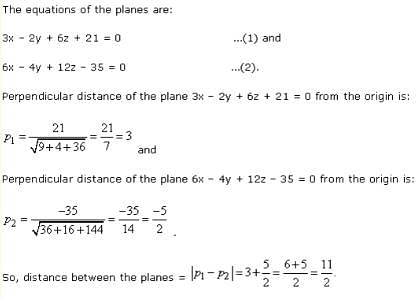
The distance between the planes 3x – 2y + 6z + 21 = 0 and – 6x + 4y – 12z + 35 = 0 is:
The distance of the point (2, 3, – 5) from the plane x + 2y – 2z = 9 is:
The foot of the perpendicular drawn from the (- 1, – 3, – 5) to a plane is (2, 4, 6). The equation of the plane is:
A point is 5 units away from the vertical plane and 4 units away from profile plane and 3 units away from horizontal plane in 1st quadrant then the projections are drawn on paper the distance between the front view and top view of point is _____________
The distance of the plane 6x – 2y + 3z = 12 from the origin is:
The distance of the point from the plane
is:
Distance of the point P (1, 1, p) from the plane whose equation is is
The angle between the line and the plane 2x – y + 2z + 7 = 0 is:
|
204 videos|290 docs|139 tests
|