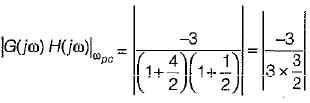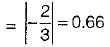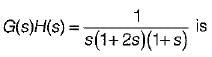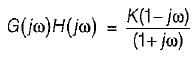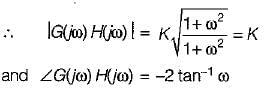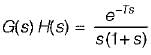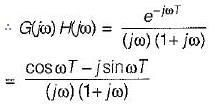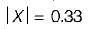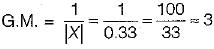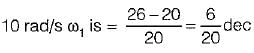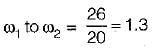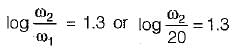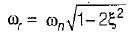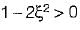Test: Frequency Domain Analysis of Control Systems- 3 - Electrical Engineering (EE) MCQ
20 Questions MCQ Test Control Systems - Test: Frequency Domain Analysis of Control Systems- 3
Phase crossover frequency is defined at
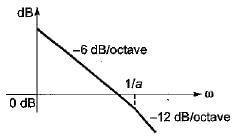
The Bode plot for a transfer function is shown below:
The steady state error corresponding to a parabolic input is

The steady state error corresponding to a parabolic input is
The frequency at which the Nyquist plot crosses the negative real axis is known as
A unity feedback system has a forward path gain of
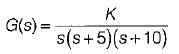
The phase angle of the Nyquist plot for ω → ∞ is
The open loop transfer function of a system is given as
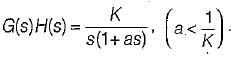
The Bode plot of this system is represented as:
A second order system has
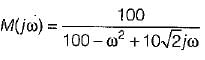
It’s Mp (peak magnitude) will be approximately
The closed loop transfer function of a control system is given by
C(s)/R(s) = 1/(1+s)
For the input r(t) = sint, the steady state value of c(t) is equal to
A system has transfer function equal to (1-s)/(1+s). Its gain at ω = 1 rad/s is
The gain margin of a unity negative feedback system having forward path transfer function
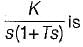
The characteristic equation of a closed loop control system is given by s2 + 4s + 16 = 0. The resonant frequency (in radian/sec) of the system is
The gain margin (in dB) of a system having the open-loop transfer function
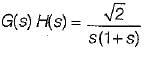 is
is
The forward path transfer function of an unity feedback system is given by
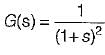
What is the phase margin for this system?
The closed loop system having the open loop transfer function,
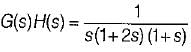 is
is
The nyquist plot for a unity feedback control system having open loop transfer function G(s) = K(1-s)/(s+1) is shown in figure below :
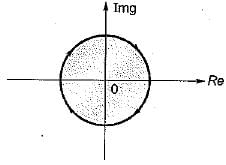
The system is
A unity feedback control system has a forward path transfer function of 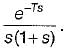 Its phase value will be zero at a frequency of ω1.
Its phase value will be zero at a frequency of ω1.
Which one of the following equation should be satisfied for it?
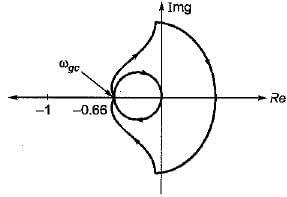
What is the approximate value of gain margin in the Nyquist diagram shown below?
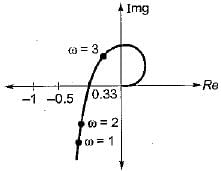
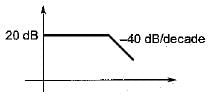
The magnitude-frequency response of a control system is given in figure below:
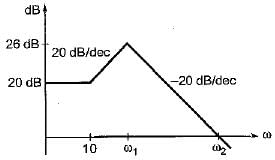
The values of ω1 and ω2 are respectively
Match List-I with List-Il and select the correct answer using the codes given below the lists:
List-I
A. Resonant peak
B. Damped natural frequency (rad/s)
C. Resonant frequency (rad/s)
D. Peak overshoot
List - II
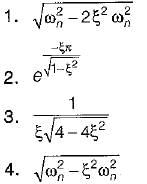
Codes:
A B C D
(a) 3 4 1 2
(b) 3 1 4 2
(c) 2 4 1 3
(d) 2 1 4 3
A second order underdamped system has a damping ratio of 0.8. It is subjected to a sinusoidal input of unit amplitude. It has resonant peak of
Band width is the range of frequencies for which system gain is
|
53 videos|74 docs|40 tests
|


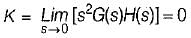

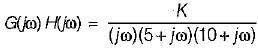
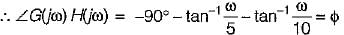
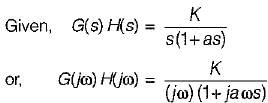
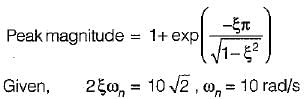
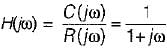
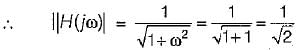
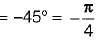
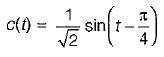
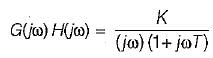
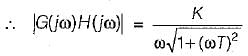
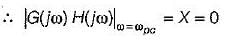
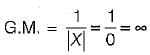
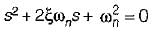

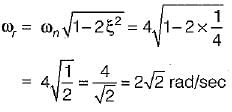
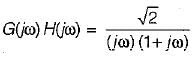
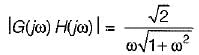
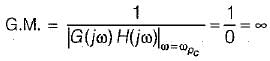
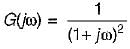
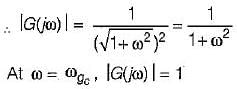
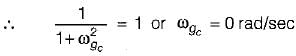
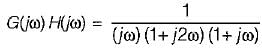
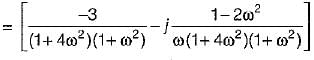
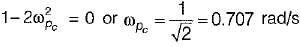 Now,
Now,