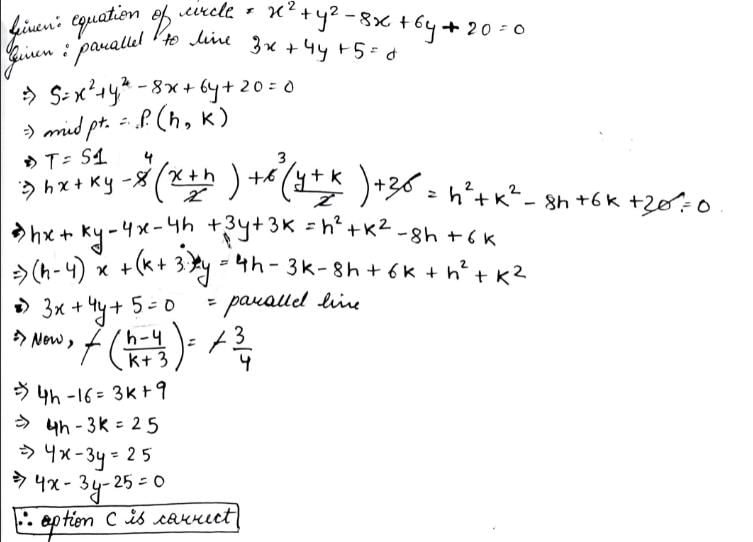Test: Circle- 3 - JEE MCQ

Slopes of tangents through (7, 1) to the circle x² + y² = 25 satisfy the equation

The circle with (4, –1) and touching x-axis is

The y-intercept of the circle x² + y² + 4x + 8y - 5 = 0 is

The centre of the circle passing through origin and making intercepts 8 and –4 on x and y axes respectively is

Equation of circles touching x-axis at the origin and the line 4x – 3y + 24 = 0 are

The equation of the chord of x² + y² - 4x + 6y + 3 = 0 whose mid point is (1, -2) is

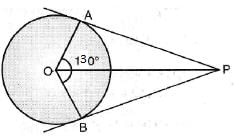
In the given figure, PA and PB are tangents from P to a circle with centre O. If ∠AOB = 130°, then find ∠APB.

If the two circles (x - 1)² + (y - 3)² = r² and x² + y² - 8x + 2y + 8 = 0 intersect in two distinct points, then

If the distance between the centres of two circles of radii 3, 4 is 25 then the length of the transverse common tangent is

The equation of the tangent to the circle x² + y² - 4x + 4y - 2 = 0 and (1, 1) is

Equation of the tangent to the circle x² + y² - 2x + 4y - 4 = 0 which is parallel to the line 3x + 4y -1 = 0 is

The equation to the side BC of ΔABC is x + 5 = 0. If (-3, 2) is the orthocentre and (0, 0) is the circumcentre then radius of the circle is

The chord of contact of (1, 2) with respect to the circle x² + y² - 4x - 6y + 2 = 0 is

The circles x² + y² - 8x + 6y + 21 = 0 , x² + y² + 4x - 10y - 115 = 0 ar

If the circles x² + y² + 2gx + 2fy = 0 x² + y² + 2g' x + 2f ' y = 0 touch each other then

Locus of the point of intersection of tangents to the circle. x² + y² + 2x + 4y - 1 = 0 which include an angle of 60^o is

Number of circles touching all the lines x + y – 1 = 0, x – y – 1 = 0 and y + 1 = 0 are

If the line y = x touches the circle x² + y² + 2gx + 2fy + c = 0 at P where OP = 6√2 then c =

If the tangent at (3, –4) to the circle x² + y² - 4x + 2y - 5 = 0 cuts the circle x² + y² + 16x + 2y + 10 = 0 in A and B then the mid point of AB is

Locus of mid points of chords to the circle x² + y² - 8x + 6y + 20 = 0 which are parallel to the line 3x + 4y + 5 = 0 is

If the circles x² + y² = 2 and x² + y² - 4x - 4y + λ = 0 have exactly three real common tangents then λ =

The locus of midpoints of chords of the circle x² + y² - 2x - 2y - 2 = 0 which make an angle of 120^o at the centre is

If P and Q are the points of intersection of the circles x² + y² + 3x + 7y + 2p - 5 = 0 and x² + y² + 2x + 2y - p² = 0 , then there is a circle passing through P, Q and (1, 1) for

Lengths of common tangents of the circles x² + y² = 6x, x² + y² + 2x = 0 are

The centre of the circle passing through the points (0, 0), (1, 0) and touching the circle x² + y² = 9 is

The line 2x – y + 1 = 0 is tangent to the circle at the point (2, 5) and the centre of the circles lies on x – 2y = 4. The radius of the circle is

Circle with centre (0, 4) and passing through the projection of (2, 4) on x-axis is