Test: Linear Inequalities- Case Based Type Questions - Commerce MCQ
10 Questions MCQ Test Mathematics (Maths) Class 11 - Test: Linear Inequalities- Case Based Type Questions
A beaker contains 640 litres of 8% solution of Boric acid. This is to be diluted by adding a 2% Boric acid solution to it.
Based on the above data, answer the following question.
Q. If x litres of 2% Boric acid solution is added to the beaker, the amount of acid becomes ........... litres.

A beaker contains 640 litres of 8% solution of Boric acid. This is to be diluted by adding a 2% Boric acid solution to it.
Based on the above data, answer the following question.
Q. If the resulting mixture is to be more than 4% but less than 6% boric acid, then the range of x is ..........

A beaker contains 640 litres of 8% solution of Boric acid. This is to be diluted by adding a 2% Boric acid solution to it.
Based on the above data, answer the following question.
Q. The initial quantity of water in the beaker is ........... litres.

A beaker contains 640 litres of 8% solution of Boric acid. This is to be diluted by adding a 2% Boric acid solution to it.
Based on the above data, answer the following question.
Q. The amount of water after the mixing is .......... litres.
A beaker contains 640 litres of 8% solution of Boric acid. This is to be diluted by adding a 2% Boric acid solution to it.
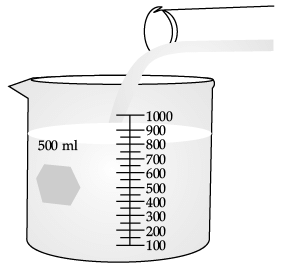
Based on the above data, answer the following question.
Q. The initial amount of acid in the beaker is ........... litres.
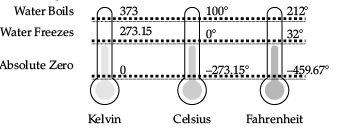
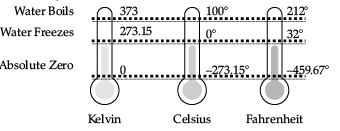
Kelvin(K), degree Celsius(°C) and degree Fahrenheit(°F) are three units of temperature. The conversion formula for them is as follows:
F = 9 / 5C + 32 and K = C + 273.15
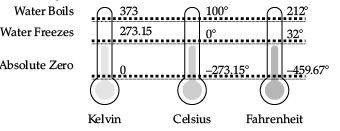
Based on the above data, answer the following question.
Q. When F = 68°F, C = ..............
Kelvin(K), degree Celsius(°C) and degree Fahrenheit(°F) are three units of temperature. The conversion formula for them is as follows:
F = 9 / 5C + 32 and K = C + 273.15
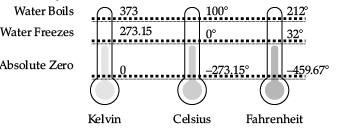
Based on the above data, answer the following question.
Q. For part (iv), the range of temperature in Kelvin(K) is ................
Kelvin(K), degree Celsius(°C) and degree Fahrenheit(°F) are three units of temperature. The conversion formula for them is as follows:
F = 9 / 5C + 32 and K = C + 273.15
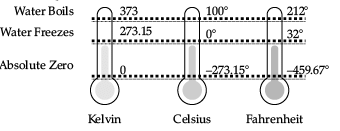
Based on the above data, answer the following question.
Q. When C = 120° F = .................
Kelvin(K), degree Celsius(°C) and degree Fahrenheit(°F) are three units of temperature. The conversion formula for them is as follows:
F = 9 / 5C + 32 and K = C + 273.15
Based on the above data, answer the following question.
Q. A solution is kept between 68°F and 77°F. What is the range in degree Celsius(C)?
Kelvin(K), degree Celsius(°C) and degree Fahrenheit(°F) are three units of temperature. The conversion formula for them is as follows:
F = 9 / 5C + 32 and K = C + 273.15
Based on the above data, answer the following question.
Q. The formula for C in terms of F is C = ...............
|
74 videos|239 docs|91 tests
|




















