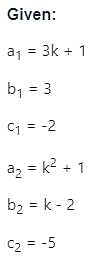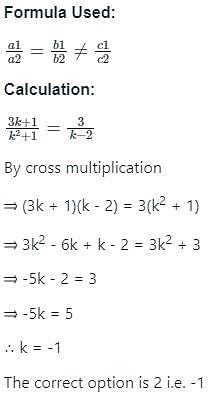All Courses
Get the App Signup / Login
Sign in Open App
Mechanical Engineering Exam  >  Mechanical Engineering Tests  >  GATE Mechanical (ME) Mock Test Series 2025  >  Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Mechanical Engineering MCQ

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Mechanical Engineering MCQ


Test Description

30 Questions MCQ Test GATE Mechanical (ME) Mock Test Series 2025 - Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 for Mechanical Engineering 2024 is part of GATE Mechanical (ME) Mock Test Series 2025 preparation. The Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 questions and answers have been prepared according to the Mechanical Engineering exam syllabus.The Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 MCQs are made for Mechanical Engineering 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 below.
Solutions of Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 questions in English are available as part of our GATE Mechanical (ME) Mock Test Series 2025 for Mechanical Engineering & Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 solutions in Hindi for GATE Mechanical (ME) Mock Test Series 2025 course. Download more important topics, notes, lectures and mock test series for Mechanical Engineering Exam by signing up for free. Attempt Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 | 30 questions in 90 minutes | Mock test for Mechanical Engineering preparation | Free important questions MCQ to study GATE Mechanical (ME) Mock Test Series 2025 for Mechanical Engineering Exam | Download free PDF with solutions
Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 1
Save

Consider the system of equations given below: 
x + y =  2
2x + 2y = 5
This system has

Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 1

(b) This can be written as AX = B Where A

Angemented matrix 

rank(A) ≠ rank(). The system is inconsistant .So system has no solution.

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 2
Save

For what value of a, if any, will the following system of equations in x, y and z have a solution?  
2x + 3y = 4
x+y+z = 4
x + 2y - z = a

Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 2

(b)


If a = 0 then rank (A) = rank() = 2. Therefore the system is consistant
∴ The system has soln .

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 3
Save

Solution for the system defined by the set of equations
4y + 3z = 8;
2x – z = 2
and 3x + 2y =5 is 

Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 3

Ans.(d)
Consider the matrix A =   ,Now det (A) = 0
So byCramer's Rule the system has no solution

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 4
Save

For what values of α and β the following simultaneous equations have an infinite numberof solutions? 
x + y + z = 5; x + 3y + 3z = 9; x + 2y + αz = β
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 4

(d)

 =


For infinite solution of the system
α − 2 = 0 and β − 7 = 0
⇒ α = 2 and β = 7.

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 5
Save

Let A be a 3 × 3 matrix with rank 2. Then AX = 0 has 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 5

(b)
We know , rank (A) + Solution space X(A) = no. of unknowns.
⇒2 + X(A) = 3 . [Solution space X(A)= No. of linearly independent vectors]
⇒ X(A) =1.

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 6
Save

A is a 3 x 4 real matrix and A x = b is an inconsistent system of equations. The highest possible rank of A is  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 6

(b). Highest possible rank of A= 2 ,as Ax = b is an inconsistent system.

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 7
Save

Consider the matrices X (4 × 3), Y (4 × 3) and P (2 × 3). The order or P (XTY)–1PT] T will be 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 7

 

The correct option is A (2 × 2)
Given, X(4×3)′ Y(4×3)′ P(2×3)

None of the given matrices is square, hence (AB)−1 = B−1A−1 does not hold for the above given matrices.

Now, Order of XTY i,e.

XT(3×4)Y(4×3) = (3×3)

⇒ Order of (XTY)−1=(3×3)

Order of P(XTY)−1=(2×3)

Order of P(XTY)−1PT=(2×2)

⇒ Order [P(XTY)−1PT]=(2×2)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 8
Save

Given matrix [A] =  the rank of the matrix is 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 8

(c)


∴Rank(A) = 2

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 9
Save

The Laplace transform of  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 9

Ans. (b)False
Laplace transform of  

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 10
Save

For what value of k, the system linear equation has no solution

(3k + 1)x + 3y - 2 = 0

(k2 + 1)x + (k - 2)y - 5 = 0
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 10

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 11
Save

If L defines the Laplace Transform of a function, L [sin (at)] will be equal to  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 11

Ans. (b)


⇒ 

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 12
Save

The Inverse Laplace transform of   is         
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 12

Ans. (c)



Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 13
Save

Laplace transform for the function f(x) = cosh (ax) is         
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 13

Ans. (b)
It is a standard result that
L (cosh at) =  

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 14
Save

If F(s) is the Laplace transform of function f (t), then Laplace transform of    
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 14

Ans. (a)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 15
Save

Laplace transform of the function sin ωt            
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 15

Ans. (b)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 16
Save

Laplace transform of (a + bt)2 where ‘a’ and ‘b’ are constants is given by:      
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 16

Ans.(c)   


Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 17
Save

A delayed unit step function is defined as Its Laplace transform is
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 17

Ans. (d)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 18
Save

The Laplace transform of the function sin2 2t is  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 18

Ans.(a)


 

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 19
Save

 Find the rank of the matrix 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 19

To find the rank of a matrix of order n , first , complete its determinant ( in the case of a square matrix). if it is not 0 , then its rank = n . if it is 0. then see weather there is any non-zero minor of order n-1. if such minor exists, then the rank of the matrix = n-1

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 20
Save

The running integrator, given by             
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 20

Ans. (b)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 21
Save

The state transition matrix for the system  X- = AX with initial state X(0) is  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 21

Correct option is C. 
Laplace inverse of [(sI−A)−1]
eAt = L−1[sI−A]−1

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 22
Save

The Fourier transform of x(t) = e–at u(–t), where u(t) is the unit step function 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 22

Ans. (d)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 23
Save

The fundamental period of the discrete-time signal is 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 23

Ans. (b)

or  

or   

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 24
Save

u(t) represents the unit step function. The Laplace transform of u(t – ζ) is   
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 24

Ans. (c)
f(t) = u(t – ζ)
L{f(t)} = L{u(t – ζ)}

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 25
Save

The fundamental period of x(t) = 2 sin πt + 3 sin 3πt, with t expressed in seconds, is 
Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 26
Save

If the Fourier transform of x[n] is X(ejω), then the Fourier transform of (–1)n x[n] is 
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 26

Ans. (c)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 27
Save

Given f(t) and g(t) as shown below:

g (t) can be expressed as                      
Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 28
Save

Given f(t) and g(t) as shown below:

The Laplace transform of g(t) is                  
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 28

Ans. (c)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 29
Save

The Laplace transform of g(t) is     
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 29

Ans. (c)

Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 30
Save

Let Y(s) be the Laplace transformation of the function y (t), then final value of the function is   
Detailed Solution for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 - Question 30

Ans. (c)

29 docs|220 tests
Join Course
Information about Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 Page
In this test you can find the Exam questions for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Systems of Linear Equations, Matrix Algebra & Transform Theory- 2, EduRev gives you an ample number of Online tests for practice

Top Courses for Mechanical Engineering

Download as PDF

Top Courses for Mechanical Engineering

Important Questions for Systems of Linear Equations, Matrix Algebra & Transform Theory- 2

Find all the important questions for Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 at EduRev.Get fully prepared for Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 resources.

Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 MCQs with Answers

Prepare for the Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 within the Mechanical Engineering exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 GATE Mechanical (ME) Mock Test Series 2025

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace Systems of Linear Equations, Matrix Algebra & Transform Theory- 2 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup