Important Questions (1 mark): Work & Energy - Class 9 MCQ
25 Questions MCQ Test - Important Questions (1 mark): Work & Energy
When a light and a heavy body have equal K.E, then which one has a greater momentum?
Kilowatt hour (kWh) represents the unit of
When speed of a motor car increases six times, then kinetic energy increases by
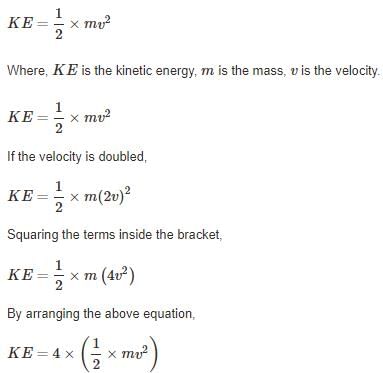
When speed of the moving object is doubled its
When time taken to complete a given amount of work increases, then
When the force applied and the displacement of the body are inclined at 90° with each other, then work done is
The KE. of a body in increased most by doubling its
If a force F is applied on a body and it move with velocity v, then power will be :-
Potential energy and kinetic energy are types of
Work done in moving a 50 kg block through a horizontal distance of 10 m by applying a force of 100N which makes an angle of 60° with the horizontal is
An elevator is designed to lift a load of 1000 kg through 6 floors of a building averaging 3·5 m per floor in 6 sec. Power of the elevator, neglecting other losses, will be
The work done by a body is directly proportional to :
Work done is said to be positive when a force causes displacement:
The work done on an object does not depends on the
A stone is tied to a string and whirled in a circular path. The work done by the stone is :
A rocket rises up in the air due to the force generated by the fuel. The work done by the:
One joule work is said to be done when a force of one newton acts through a distance of: