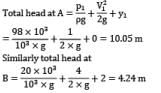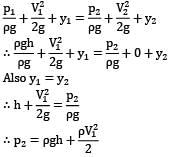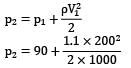Test: Fluid Dynamics Level - 1 - Mechanical Engineering MCQ
10 Questions MCQ Test Fluid Mechanics for Mechanical Engineering - Test: Fluid Dynamics Level - 1
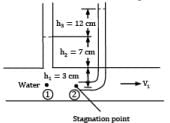
A piezometer and a Pitot tube are tapped into a horizontal water pipe, as shown in figure. The velocity of water at the center of the pipe is

Both free vortex and forced vortex can be expressed mathematically in terms of tangential velocity V at the corresponding radius r. Choose the correct combination.
Free vortex Forced vortex
At point A in a pipeline carrying water, the diameter is 1 m, the pressure 98 kPa and the velocity 1m/s. At point B, 2 m higher than A, the diameter is 0.5 m and the pressure 20 kPa.
The direction of flow would be [
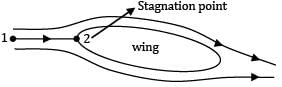
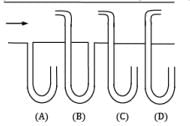
Which configuration measures the stagnation pressure?
The pressure at a point in the fluid is 4.9 N/cm2. Find height when the fluid under consideration is in oil of specific gravity of 0.85.
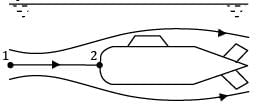
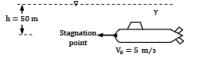
A submarine moves through seawater (SG = 1.03) at a depth of 50 m with velocity V0 = 5.0 m/s as shown in Fig. Determine the pressure at the stagnation point on the front of the submarine (in kPa).
A light plane flies at 720 km/hr in standard air at an altitude of 1000 m. Determine the absolute stagnation pressure at the leading edge of the wing. Take, pair = 9.0 × 104 N/m2, ρ = 1.1 kg/m3.
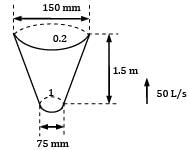
A vertical water pipe, 1.5 m long, diverges from 75 mm diameter at the bottom to 150 mm diameter at the top and the rate of flow is 50 L/s upwards. If the pressure at the bottom end is 150 kN/m2 , the pressure at the top will be nearly
(!) 195.2 kN⁄m2
An open tank contains water upto a depth of 350 cm and above it an oil of specific gravity 0.65 for a depth of 2.5 m. Find the pressure intensity at the extreme bottom of the tank.
Statement (I): There exists a positive pressure difference between the inlet and throat of a venturi meter.
Statement (II): The coefficient of discharge of a venturi meter accounts for the nonuniformity of flow at both inlet and throat.
|
56 videos|106 docs|75 tests
|