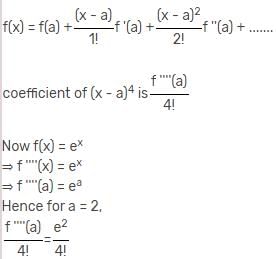Test: Fourier Series, Numerical Methods & Complex Variables- 1 - Mechanical Engineering MCQ

The function x(t) is shown in the figure. Even and odd parts of a unit-step function u(t) are respectively,  

In the Taylor series expansion of e^x about x = 2, the coefficient of (x - 2)⁴ is 

The Fourier series expansion of a symmetric and even function, f(x) where 

 Will be 

The Fourier series expansion    of the periodic signal shown below will contain the following nonzero terms  

The Fourier series for the function f(x)=sin²x is  

X(t) is a real valued function of a real variable with period T. Its trigonometric Fourier Series expansion contains no terms of frequency ω = 2π (2k ) /T ; k = 1, 2,.... Also, no sine terms are present. Then x(t) satisfies the equation 

 The residue of the function f(z) 

The residues of a complex function  ​ at its poles are   ​

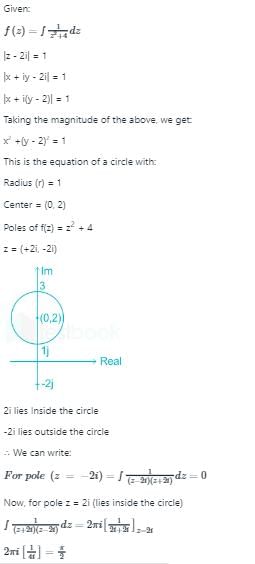
The value of the contour integral    in positive sense is  

The integral    evaluated around the unit circle on the complex plane for   

An analytic function of a complex variable z = x + iy is expressed as f(z) = u (x, y) + i v(x, y) where i =√−1 . If u = xy, the expression for v should be 

The analytic function     has singularities at   

The value of the integral    (where C is a closed curve given by |z| = 1) is 

 Roots of the algebraic equation x³ +x² +x+ 1 = 0 are 

The algebraic equation    

F (s ) = s⁵ − 3s⁴+ 5s³− 7s² + 4s + 20 is given F ( s ) = 0 has