Test: Index Numbers- 4 - CA Foundation MCQ

From the following data

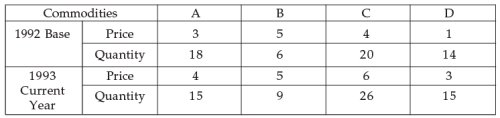
Q. The Passche price Index number is : 

From the following data

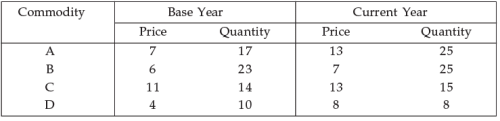
Q. The Marshall Edge worth Index number is : 

The circular Test is an extension of

Circular test, an index constructed for the year ‘x’ on the base year ‘y’ and for the year ‘y’ on the base year ‘z’ should yield the same result as an index constructed for ‘x’ on base year ‘z’ i.e. I_0,1 x I_1,2 x I_2,0 equal is :

In 1976 the average price of a commodity was 20% more than that in 1975 but 20% less than that in 1974 and more over it was 50% more than that in 1977. The price relatives using 1975 as base year (1975 price relative = 100) then the reduce date is :

Time Reversal Test is represented by symbolically is :

The prices of a commodity in the years 1975 and 1980 were 25 and 30 respectively, taking 1975 as base year the price relative is :

From the following data

Q. (Base 1992 = 100) for the year 1993–97. The construction of chain index is :

During a certain period the cost of living index number goes up from 110 to 200 and the salary of a worker is also raised from Rs. 325 to Rs. 500. The worker does not get really gain. Then the real wages decreased by :

Net monthly salary of an employee was Rs. 3000 in 1980. The consumer price index number in 1985 is 250 with 1980 as base year. If the has to be rightly compensated. Then 7th dearness allowances to be paid to the employee is :

Net Monthly income of an employee was Rs. 800 in 1980. The consumer price Index number was 160 in 1980. It is rises to 200 in 1984. If he has to be rightly compensated. The additional dearness allowance to be paid to the employee is :

When the cost of Tobacco was increased by 50%, a certain hardened smoker, who maintained his formal scale of consumption, said that the rise had increased his cost of living by 5%. Before the change in price, the percentage of his cost of living was due to buying Tobacco is

If the price index for the year, say 1960 be 110.3 and the price index for the year, say 1950 be 98.4. Then the purchasing power of money (Rupees) of 1950 will be of 1960 is

When index number is calculated for several variables, it is called: 

The consumer price Index for April 1985 was 125. The food price index was 120 and other items index was 135. The percentage of the total weight of the index is

The total value of retained imports into India in 1960 was Rs. 71.5 million per month. The corresponding total for 1967 was Rs. 87.6 million per month. The index of volume of retained imports in 1967 composed with 1960 (= 100) was 62.0. The price index for retained inputs for 1967 our 1960 as base is

During the certain period the C.L.I. gives up from 110 to 200 and the Salary of a worker is also raised from 330 to 500, then the real terms is

From the following data

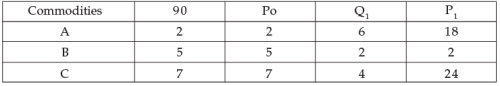
Q. Then the fisher’s quantity index number is

From the following data

Q. Then index numbers from G. M. Method is :

Using the following data

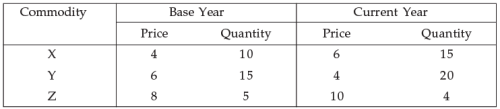
Q. The Paasche’s formula for index is :

Group index number is represented by

 The quantity Index number using Fisher formula satisfies:

For constructing consumer price Index is used:

 If the 1970 index with base 1965 is 200 and 1965 index with base 1960 is 150, the index 1970 on base 1960 will be:

 With regard to Laspeyre and Paasche price index numbers, it is maintained that If the Prices of all the goods change in the same ratio, the two indices will be equal for them the weighting system is irrelevant; or if the quantities of all the goods change in the same ratio, they will be equal, for them the two weighting systems are the same relatively. Then the above statements satisfy.

In 1996 the average price of a commodity was 20% more than in 1995 but 20% less than in 1994; and more over it was 50% more than in 1997 to price relatives using 1995 as base (1995 price relative 100) Reduce the data is:

The value index is equal to: