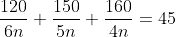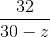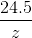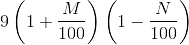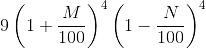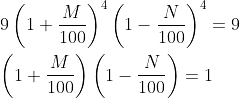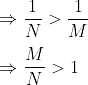Test Level 2: Ratio & Proportion - 2 - CAT MCQ
20 Questions MCQ Test Level-wise Tests for CAT - Test Level 2: Ratio & Proportion - 2
On her birthday, Ritu distributes 124 chocolates in her class. She gives 4 chocolates to each girl and 3 chocolates to each boy. If she had given 2 chocolates to each boy and girl, she would have saved 52 chocolates. The ratio of number of girls to boys in Ritu's class is:
Glass A is filled with 2/3rd of wine and the remaining portion is filled with water. Similarly, glass B of same size is filled with 4/7th of wine and remaining with water. The contents of the two glasses are poured in a tumbler and mixed. The ratio of water to wine in the tumbler is:
Of all the students in a certain dormitory, half are first-year students and the rest are second-year students. If 4/5 of the first-year students have not declared a major and the fraction of second-year students who have declared a major is 3 times the fraction of the first-year students who have declared a major, then what fraction of all the students in the dormitory are second-year students who have not declared a major?
Directions: In a paper of CAT there are 3 sections, each contains 25 questions and each question carries 4 marks. Wrong answer carries 1 mark negative. There is no negative or positive marking for not attempted question. A student attempted the questions from section A, B and C in the ratio 6 : 5 : 4. The number of correct answers are in the ratio 4 : 3 : 2, respectively. The student got 96 marks in section A.
Q. How many marks will the student get in section C?
The cost of a diamond varies directly as the square of its weight. Once, this diamond broke into four pieces with weights in the ratio 1 : 2 : 3 : 4. When the pieces were sold, the merchant got Rs. 70,000 less. Find the original price of the diamond.
A sum of Rs. 735 was divided among A, B and C. If each of them had received Rs. 25 less, then their shares would have been in the ratio 1 : 3 : 2. What is the money received by C?
$430 is divided among 45 persons consisting of men, women and children. The sum of shares of men, women and children is in the ratio 12 : 15 : 16, but the individual shares of a man, a woman and a child are in the ratio 6 : 5 : 4. Find the share of each man, woman and child, respectively.
P and Q are two different mouth fresheners, prepared by mixing Supari and Saunf in the ratios 9 : 4 and 9 : 17, respectively. If equal quantities of the P and Q mouth fresheners are mixed to form a third kind of mouth freshener R, find the ratio of Saunf and Supari in R.
Eight bananas and six apples cost a certain amount. If the cost of a banana increases by 10% and the cost of an apple decreases by 10%, then eight bananas and six apples will cost the same amount. What is the ratio of the cost of a banana to that of an apple?
The number of goals scored by a team in a football match varies directly as the square of the number of international level players in the team. If the difference in goals scored when four and six international players participate is five goals, then how many goals will be scored when eight international level players participate?
Jagan and Rex had a total of 30 cars in their showrooms. Both sold their cars at different prices, but earned the same amount of money. If Jagan had sold his cars at Rex's price, he would have earned Rs. 32 lakh. If Rex had sold his cars at Jagan's price, he would have earned Rs. 24.5 lakh. At what price did Rex sell each of his cars?
The force of attraction between two bodies of mass m1 and m2 varies directly as the product of their masses and inversely as the square of the distance between them. If the masses of the bodies and the distance between them are doubled, then the force of attraction will
The Rice Tent Company sells tents in two different sizes, large and small. Last year, the company sold 200 tents, out of which one-quarter were large. The sales of the large tents produced one-third of the company`s income. What was the ratio of the price of a large tent to the price of a small tent last year?
A pet merchant started his business at the end of 2008 by buying nine score pets of different kinds. Every year he added M% of the pets at the beginning and sold N% of the pets at the end, where both M and N are more than zero. The merchant had nine score pets at the end of the year 2012, after making the sales for that year. Which of the following can be the possible value of M : N?
Robin and Robert have some money in the ratio of 4 : 3. Robin gives Rs.p to Robert. Now, they have equal amounts of money. But Robert spends Rs.q out of the total money he has. Now again, the ratio is 4 : 3. Find the ratio p : q.
In an examination, the number of students who passed and those who failed were in the ratio 5 : 2. Had 25 more appeared and 5 less passed, the ratio of passed to failed would have been 3 : 2. Find the total number of students who appeared in the examination.
If the work done by p men in (p + 2) days is to the work done by (p + 4) men in (p – 1) days is in the ratio 1 : 1, then the value of p is
Rahim covers a distance of 48 km performed by train, bike and car in that order, the distance covered by the three ways in that order are in the ratio of 8 : 1 : 3 and charges per kilometer in that order are in the ratio of 8 : 1 : 4. If the train charges being 24 ₹ per kilometer, the total cost of the journey is
Concentrations of three type of milks X, Y and Z are 10%, 20% and 30%, respectively. They are mixed in the ratio 2 : 3 : P resulting in a 23% concentration solution. Find P.
In a co-educational school there are 15 more girls than boys. If the number of girls is increased by 10% and the number of boys is also increased by 16%, there would be 9 more girls than boys. What is the number of students in the school?
|
5 docs|272 tests
|