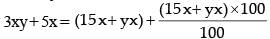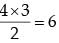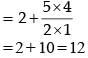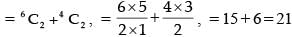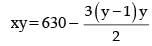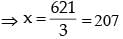Test: Permutation, Combination & Probability - 2 (2017-2010) - UPSC MCQ
15 Questions MCQ Test - Test: Permutation, Combination & Probability - 2 (2017-2010)
A bag contains 20 balls. 8 balls are green, 7 are white and 5 are red. What is the minimum number of balls that must be picked up from the bag blindfolded (without replacing any of it) to be assured of picking at least one ball of each colour?
[2017]
Four-digit numbers are to be formed using the digits 1, 2, 3 and 4; and none of these four digits are repeated in any manner. Further,
1. 2 and 3 are not to immediately follow each other
2. 1 is not to be immediately followed by 3
3. 4 is not to appear at the last place
4. 1 is not to appear at the first place
How many different numbers can be formed?
[2016]
1. 2 and 3 are not to immediately follow each other
2. 1 is not to be immediately followed by 3
3. 4 is not to appear at the last place
4. 1 is not to appear at the first place
How many different numbers can be formed?
| 1 Crore+ students have signed up on EduRev. Have you? Download the App |
A person ordered 5 pairs of black socks and some pairs of brown socks. The price of a black pair was thrice that of a brown pair. While preparing the bill, the bill clerk interchanged the number of black and brown pairs by mistake which increased the bill by 100%. What was the number of pairs of brown socks in the original order?
[2015]
A student has to opt for 2 subjects out of 5 subjects for a course, namely, Commerce, Economics, Statistics, Mathematics I and Mathematics II. Mathematics II can be offered only if Mathematics I is also opted. The number of different combinations of two subjects which can be opted is
[2015]
A selection is to be made for one post of Principal and two posts of Vice-Principal. Amongst the six candidates called for the interview, only two are eligible for the post of Principal while they all are eligible for the post of Vice-Principal. The number of possible combinations of selectees is
[2015]
In a box of marbles, there are three less white marbles than the red ones and five more white marbles than the green ones. If there are a total of 10 white marbles, how many marbles are there in the box?
[2015]
In a society it is customary for friends of the same sex to hug and for friends of opposite sex to shake hands when they meet. A group of friends met in a party and there were 24 handshakes. Which one among the following numbers indicates the possible number of hugs?
[2015]
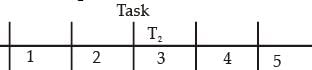
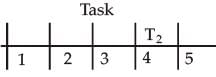
There are 5 tasks and 5 persons. Task-l cannot be assigned to either person-l or person-2. Task-2 must be assigned to either person-3 or person-4. Every person is to be assigned one task. In how many ways can the assignment be done?
[2015]
Twelve people form a club. By picking lots, one of them will host a dinner for all once in a month. The number of dinners a particular member has to host in one year is
[2015]
A group of 630 children is seated in rows for a group photo session. Each row contains three less children than the row in front of it. Which one of the following number of rows is not possible?
[2014]
There are 100 students in a particular class. 60% students play cricket, 30% student play football and 10% students play both the games. What is the number of students who play neither cricket nor football?
[2011]
In how many ways can four children be made to stand in a line such that two of them, A and B are always together?
[2010]
When ten persons shake hands with one another, in how many ways is it possible?
[2010]
A person X has four notes of Rupee 1, 2, 5 and 10 denomination. The number of different sums of money she can form from them is
[2010]
A question paper had ten questions. Each question could only be answered as True (T) or False (F). Each candidate answered all the questions. Yet, no two candidates wrote the answers in an identical sequence. How many different sequences of answers are possible?
[2010]