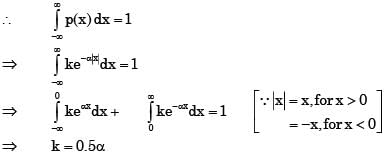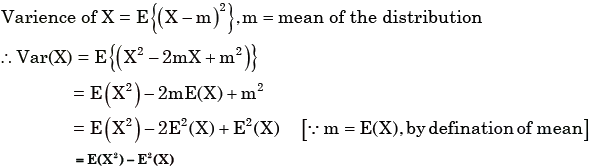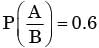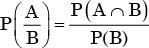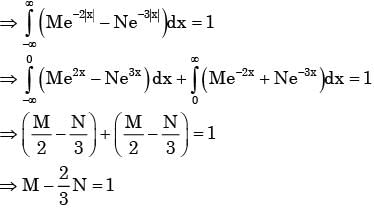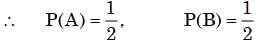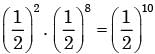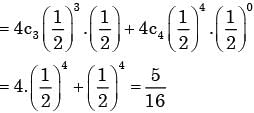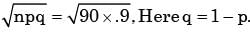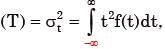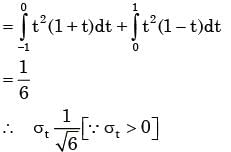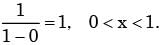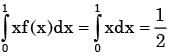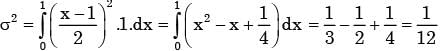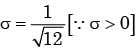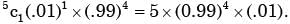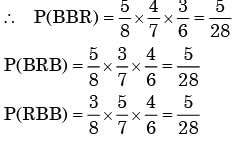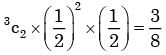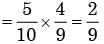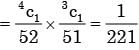Test: Probability Part:- 1 - Mathematics MCQ
20 Questions MCQ Test Additional Topics for IIT JAM Mathematics - Test: Probability Part:- 1
A fair dice is rolled twice. The probability that an odd number will follow an even number is
A probability density function is of the form
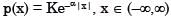
The value of K is
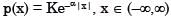
The value of K is
Three companies X, Y and Z supply computers to a university. The percentage of computers supplied by them and the probability of those being defective are tabulated below
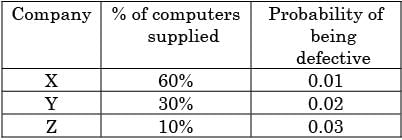
Given that a computer is defective, the probability that it was supplied by Y is

Given that a computer is defective, the probability that it was supplied by Y is
If E denotes expectation, the variance of a random variable X is given by
An examination consists of two papers, Paper 1 and Paper 2. The probability of failing in Paper 1 is 0.3 and that in Paper 2 is 0.2. Given that a student has failed in Paper 2, the probability of failing in Paper 1 is 0.6. The probability of a student failing in both the papers is
Px(x) = M exp(–2|x|) – N exp(–3 |x|) is the probability density function for the real random variable X, over the entire x axis. M and N are both positive real numbers. The equation relating M and N is
A fair coin is tossed 10 times. What is the probability that ONLY the first two tosses will yield heads?
A fair coin is tossed independently four times. The probability of the event "the number of time heads shown up is more than the number of times tails shown up" is
In a manufacturing plant, the probability of making a defective bolt is 0.1. The mean and standard deviation of defective bolts in a total of 900 bolts are respectively
Consider the continuous random variable with probability density function
f(t) = 1 + t for -1 ≤ t ≤ 0
= 1 - t for 0 ≤ t ≤ 1
The standard deviation of the random variables is
The standard deviation of a uniformly distributed random variable between 0 and 1 is
The probability that two friends share the same birth-month is
The probability of a defective piece produced in a manufacturing process is 0.01. The probability that out of 5 successive pieces, only one is defective, is
A box contains 5 block balls and 3 red balls. A total of three balls are picked from the box one after another, without replacing them back. The probability of getting two black balls and one red ball is
An unbiased coin is tossed three times. The probability that the head turns up in exactly two cases is
Two dice are thrown. What is the probability that is the sum of the numbers on the two dice is eight?
Manish has to travel from A to D changing buses at stops B and C enroute. The maximum waiting time at either stop can be 8 minutes each, but any time of waiting up to 8 minutes is equally likely at both places. He can afford up to 13 minutes of total waiting time if he is to arrive at D on time. What is the probability that Manish will arrive late at D?
Arrivals at a telephone booth are considered to be poison, with an average time of 10 minutes between successive arrivals. The length of a phone call is distributes exponentially with mean 3 minutes. The probability that an arrival does not have to wait before service is
A box contains 5 black and 5 red balls. Two balls are randomly picked one after another from the box, without replacement. The probability for both balls being red is
From a pack of regular from a playing cards, two cards are drawn at random. What is the probability that both cards will be Kings, if first card in NOT replaced
|
2 videos|44 docs|4 tests
|