Test: Transformers - EmSAT Achieve MCQ
10 Questions MCQ Test Physics for EmSAT Achieve - Test: Transformers
Energy lost due to hysteresis during each cycle in the core of a transformer working at 50 Hz is 6 J. What will be the power loss due to hysteresis?
For a 20 - kVA transformer, the iron and copper losses at full load are 300 W and 250 W, respectively. What is the transformer efficiency under full load at unity power factor?
Under load conditions, the efficiency of two identical transformers can be measured by:
A transformer has positive voltage regulation when its load power factor is:
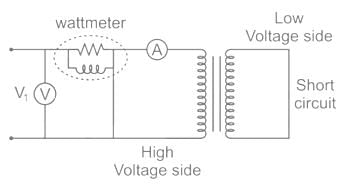
Which is to be short circuited on performing short circuit test on a transformer?
Generally the no-load losses of an electrical machine is represented in its equivalent circuit by a
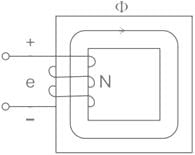
A single-phase 111-V, 50-Hz supply is connected to a coil with 200 turns of a coil-core assembly as shown in the given figure. Find the magnitude of maximum flux in the core.
In order to minimize the inrush current in a single-phase transformer, the supply switch should be closed at the instant when:
|
212 videos|286 docs|193 tests
|