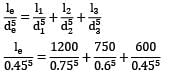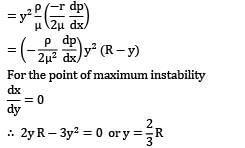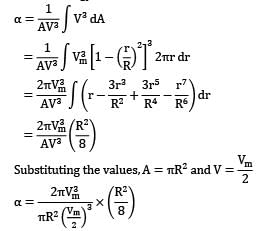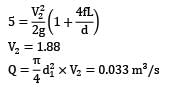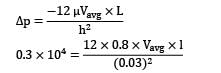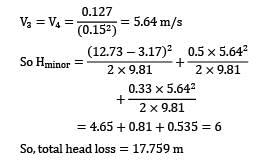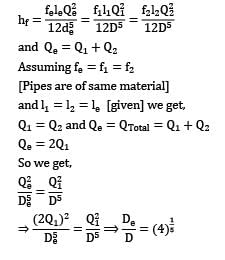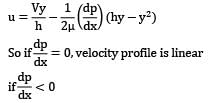Test: Viscous Flow of Incompressible Fluids, Flow Losses Level - 2 - Mechanical Engineering MCQ
20 Questions MCQ Test - Test: Viscous Flow of Incompressible Fluids, Flow Losses Level - 2
A piping system consists of three pipes arranged in series; the lengths of the pipes are 1200 m, 750 m and 600 m and diameters 750 mm, 600 mm and 450 mm respectively.
Transform the system to an equivalent 450 mm diameter pipe
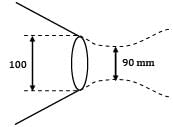
A fluid jet is discharging from a 100 mm nozzle and the vena contracta formed has a diameter of 90 mm. If the coefficient of velocity is 0.95, then the coefficient of discharge for the nozzle is
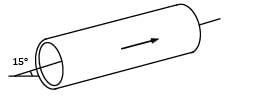
Oil at 20°C (ρ = 888 kg⁄m3 and μ = 0.800 kg ⁄ ms) is flowing steadily through a 5-cm-diameter 40-m-long pipe as shown in the below figure. The pressure at the pipe inlet and outlet are measured to be 745 and 97 kPa, respectively. Determine the flow rate of oil through the pipe (in m3/s) assuming the pipe is inclined 15° upward.

Consider the diagram below.
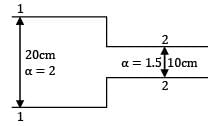
ρ = 1000 kg/m3 = constant
If pressure at 1 = 150 kPa and pressure at 2 = 100 kPa, the loss coefficient = 0.5 and discharge = 0.01 m3 /s. Calculate the major head loss, correct upto 2 decimal places (in m)
Where does the point of maximum instability exist for laminar flow through a pipe of radius R?
The value of kinetic energy correction factor for laminar flow through a circular pipe is approximately equal to
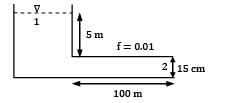
Water is discharged from a tank maintained at a constant head of 5 m above the exit of a straight pipe 100 m long and 15 cm in diameter. If the friction coefficient for the pipe is 0.01, the rate of flow will be nearly
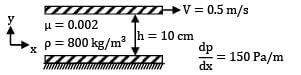
An oil of viscosity 8 poise flows between two parallel fixed plates, which are kept at a distance of 30 mm apart. If the drop of pressure for a length of 1 m is 0.3 × 104 N⁄m2 and width of the plates is 500 mm, the rate of oil flow between the plates will be
With reference to section ① and ② consider the following statements, neglecting all losses.
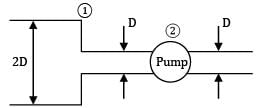
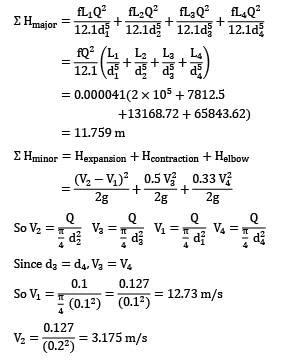
Considering all the major and minor losses in the pipe network, the total head loss is (Assume Q and Darcy friction factor, f is a constant) __________ m.
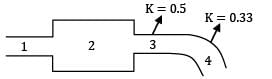
1: Length = 200 cm, diameter = 10 cm
2: Length = 2.5 m, diameter = 20 cm
3: Length = 1 m, diameter = 15 cm Loss coefficient = 0.5
4: Mean length = 500 cm, diameter = 15 cm Loss coefficient = 0.33
Q = 0.1 m3 /s, f = 0.05. Assume flow is laminar everywhere.
Two reservoirs connected by two pipelines in parallel of the same diameter D and length l. It is proposed to replace the two pipelines by a single pipeline of the same length l, without affecting the total discharge and loss of head due to friction. The diameter of the equivalent pipe De in terms of the diameter of the existing pipeline, De / D is
Consider fully developed laminar flow in a circular pipe of a fixed length
1. The friction factor is inversely proportional to Reynolds number
2. The pressure drop in the pipe is proportional to the average velocity of the flow in the pipe
3. The friction factor is higher for a rough pipe as compared to a smooth pipe
4. The pressure drop in the pipe is proportional to the square of average of flow in the pipe
What of the above statements are correct?
The space between two plates (20cm*20cm*1cm), 1 cm apart, is filled with a liquid of viscosity 1 Poise. The upper plate is dragged to the right with a force of 5N keeping the lower plate stationary.
What will be the velocity in m/s of flow at a point 0.5 cm below the lower surface of the upper plate if linear velocity profile is assumed for the flow?
Consider the case of minor head loss due to sudden contraction
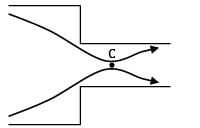
(here C is the point of vena contracta)
Which of the following is correct?
A 0.20 m diameter pipe 20 km long transports oil at a flow rate of 0.01 m3⁄s. Calculate power required to maintain flow if dynamic viscosity and density of oil is 0.08 Pas and 900 kg⁄m3 respectively.
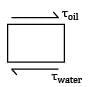
Consider flow of oil and water through a channel; the boundary conditions at the interface are
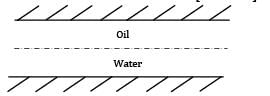
Consider the image below.
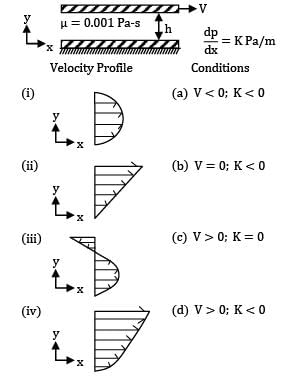
Codes: (i) (ii) (iii) (iv)
Assertion (A): The power transmitted through a pipe is maximum when the loss of head due to friction is equal to one-third of total head at the inlet.
Reason (R): Velocity is maximum when the friction loss is one-third of the total head at the inlet.
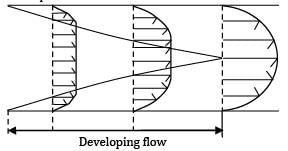
Which one of the following is incorrect for the developing region of the pipe flow?
For viscous, incompressible flow between two flat plates as shown, the velocity at y = h / 2 is