All Courses
Get the App Signup / Login
Sign in Open App
CAT Exam  >  CAT Tests  >  Test: XAT Quant 2016 - CAT MCQ

Test: XAT Quant 2016 - CAT MCQ


Test Description

29 Questions MCQ Test - Test: XAT Quant 2016

Test: XAT Quant 2016 for CAT 2024 is part of CAT preparation. The Test: XAT Quant 2016 questions and answers have been prepared according to the CAT exam syllabus.The Test: XAT Quant 2016 MCQs are made for CAT 2024 Exam. Find important definitions, questions, notes, meanings, examples, exercises, MCQs and online tests for Test: XAT Quant 2016 below.
Solutions of Test: XAT Quant 2016 questions in English are available as part of our course for CAT & Test: XAT Quant 2016 solutions in Hindi for CAT course. Download more important topics, notes, lectures and mock test series for CAT Exam by signing up for free. Attempt Test: XAT Quant 2016 | 29 questions in 45 minutes | Mock test for CAT preparation | Free important questions MCQ to study for CAT Exam | Download free PDF with solutions
Test: XAT Quant 2016 - Question 1
Save

Consider the set of numbers {1, 3, 32, 33... 3100}. The ratio of the last number and the sum of the remaining numbers is closest to:

Detailed Solution for Test: XAT Quant 2016 - Question 1
Sum of the remaining numbers would be a GP series whosea = 1, n = 100 and r = 3.

Thus, ratio would be (3100/ [(1 x 3100 - 1)/3-1]) = 2

We are assuming (for all practical purposes) 1 x 3100 – 1 = 3100

Test: XAT Quant 2016 - Question 2
Save

Anita, Biplove, Cheryl, Danish, Emily and Feroze compared their marks among themselves.Anita scored the highest marks, Biplove scored more than Danish. Cheryl scored more than at least two others and Emily had not scored the lowest.

Statement I: Exactly two members scored less than Cheryl.

Statement II: Emily and Feroze scored the same marks.

Which of the following statements would be sufficient to identify the one with the lowestmarks?

Detailed Solution for Test: XAT Quant 2016 - Question 2
From the main part we get

A is highest.

B > D.

E is not at 6th position.

C can have positions 2, 3 or 4.

Hence, 6th position holder can be D or F.

From Statement I: C is at 4th position.

Using Statement I we cannot confirm 6th position holder. It can be D or F.

Form statement II: E = F. As E is not in 6th position, F is also not in 6th position.

Then, D is 6th position holder.

Option B is the correct answer.

1 Crore+ students have signed up on EduRev. Have you? Download the App
Test: XAT Quant 2016 - Question 3
Save

Rani bought more apples than oranges. She sells apples at Rs. 23 apiece and makes 15%profit. She sells oranges at Rs. 10 apiece and marks 25% profit. If she gets Rs. 653 afterselling all the apples and oranges, find her profit percentage.

Detailed Solution for Test: XAT Quant 2016 - Question 3
Assume Rani sells X apples and Y oranges, where X > Y

Then, 23X + 10Y = 653

Then try X = 1, 11, 21 etc. (to get last digit = 3)

We find X = 21 and Y = 17 is the only solution where X > Y

Cost of an Apple = 23/1.15 and cost of an Orange = 10/1.25

Overall cost = 483/1.15 + 170/1.25 = 556

Then profit = 653 – 556 = 97

Profit percentage = (97/556) x 100 = 17.4%

Test: XAT Quant 2016 - Question 4
Save

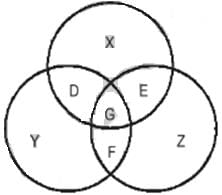
In an amusement park along with the entry pass a visitor gets two of the three availablerides (A, B and C) free. ON a particular day 77 opted for ride A, 55 opted for B and 50 opted for C; 25 visitors opted for both A and C, 22 opted for both A and B, while no visitor opted for both B and C. 40 visitors did not opt for ride A and B, or both. How many visited with the entry pass on that day?
Detailed Solution for Test: XAT Quant 2016 - Question 4

Assume, M = Number of visitors who did not opt for any of A, B or C.

Given,

X + D + E + G = 77

Y + D + G + F = 55

Z + E + G + F = 50

Z + M = 40

E + G = 25

D + G = 22

G + F = 0

Then, G = 0, F = 0.

E = 25

D = 22

X = 30

Y = 33

Z = 25

M = 15

Total number of visitors = X + Y + Z + D + E + F + G + M = 150

Hence, Option E is the correct answer.

Test: XAT Quant 2016 - Question 5
Save

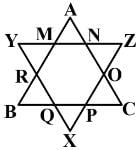
ΔABC and ΔXYZ are equilateral triangles of 54 cm sides. All smaller triangles like ΔANM, ΔOCP, ΔQPX etc. are also equilateral triangles. Find the area of the shape MNOPQRM.
Detailed Solution for Test: XAT Quant 2016 - Question 5

Area of any equilateral triangle = (√3/2) x (side) 4AB = 54 cm. Then, MR = 18 cm. Area of ∆YMR =81√3 sq. cm. and

area of ∆XYZ = 729√3 sq. cm.

Finally, area of shape MNOPQRM

= 729√3 – 3 x 81√3 = 486√3 sq. cm.

Using formula of hexagon, area of shape MNOPQRM

= (3√3/2) x 182 = 486 √3 sq. cm.

Test: XAT Quant 2016 - Question 6
Save

In the figure below, AB = AC = CD. If < />
Detailed Solution for Test: XAT Quant 2016 - Question 6
Given, AB = AC. Then ∠ABC =∠ACB

Also, AC = CD. Then ∠CAB =∠ADC =∠ADB = 20°

From the properties of triangle,

∠ACB =∠CAD +∠ADC = 40°

Now, ∠BAC = 180°−∠ABC −∠ACB

= 180°− 40°− 40°= 100°

Finally, ∠BAD =∠BAC =∠CAD = 100°+ 20°= 120°

Hence, Option D is the correct answer.

Test: XAT Quant 2016 - Question 7
Save

Akhtar plans to cover a rectangular floor of dimensions 9.5 meters and 11.5 meters using tiles. Two types of square shaped tiles are available in the market. A tile with side 1-metercosts Rs. 100 and a tile with side 0.5 meters costs Rs. 30. The tiles can be cut if required.What will be the minimum cost of covering the entire floor with tiles?
Detailed Solution for Test: XAT Quant 2016 - Question 7
We should use 1 meter tiles (cost 100) before 0.5 meter side tiles (cost 4 × 30 = 120).

Area of A = 9 × 11 sq. meters

Area of B = 0.5 × 11 sq. meters

Area of C = 0.5 × 9 sq. meters

Area of D = 0.5 × 0.5 sq. meters

For A we need 99 tiles with 1 meter side

For B and C together we need (11 + 9)/2 tiles with 1 meter side

(we need to break each tile into two equal areas)

For D we need 1 tile with 0.5 meter side

Hence, the total cost = 9900 + 1000 + 30 = 10930

Test: XAT Quant 2016 - Question 8
Save

Pradeep could either walk or drive to office. The time taken to walk to the office is 8 timesthe driving time. One day, his wife took the car making him walk to office. After walking 1 km, he reached a temple when his wife called to say that he can now take the car. Pradeep figure that continuing to walk to the office will take as long as walking back home and then driving to the office. Calculate the distance between the temple and the office.
Detailed Solution for Test: XAT Quant 2016 - Question 8
Suppose the distance between his home and office is x km.

Then, distance between his office and temple is (x – 1) km.

If he walks at a speed of y km/hr. Then his driving speed

= 8y km/hour, as he is 8 times faster in car.

Then,

1/y + x/8y = (x - 1)/y

or, 1+ x/8y = x-1

or, x = 16/7

finally, x – 1 = 9/7

Option C is the correct answer.

Test: XAT Quant 2016 - Question 9
Save

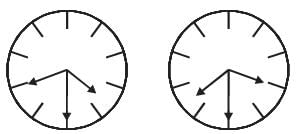
Each day on Planet M is 10 hours, each hour 60 minutes and each minute 40 seconds. Theinhabitants of Planet M use 10-hour analog clock with an hour hand, a minute hand and a second hand. If one such clock shows 3 hours 42 minutes and 20 seconds in a mirror what will be the time in Planet M exactly after 5 minutes?
Detailed Solution for Test: XAT Quant 2016 - Question 9

In the above figure, left side diagram shows mirror image and right side diagram shows actual clock.

Form the drawing, actual time = 6 hours 17 min 20 seconds.

After 5 minutes actual time = 6 hours 22 min 20 seconds.

Option B is the correct answer.

Test: XAT Quant 2016 - Question 10
Save

A square piece of paper is folded three times along its diagonal to get an isosceles trianglewhose equal sides are 10 cm. What is the area of the unfolder original piece of paper?
Detailed Solution for Test: XAT Quant 2016 - Question 10
The smaller side of the isosceles triangle = 10

Then the side of the square = 20 and the area of the square

= 400 sq. cm.

Option A is the correct answer.

Test: XAT Quant 2016 - Question 11
Save

The difference between the area of the circumscribed circle and the area of the inscribedcircle of an equilateral triangle is 2156 sq. cm. What is the area of the equilateral triangle?
Detailed Solution for Test: XAT Quant 2016 - Question 11

In an equilateral triangle, both circles have same centre (point B). Assume, AB is radius of circumscribed circle and BC is the radius of inscribed circle, where BCA is right angle. Then,

BC/AB = sin30° = ½

Let, BC = x and AB = 2x.

Then, AC = x√3

Side of the triangle = 2x√3

Area of the triangle = 12x2√3 /4

Then, 4πx2 – πx2 = 2156 Or x2 = 2156 x 7/66

Finally, area of the triangle = 686√3

Option A is the correct answer.

Test: XAT Quant 2016 - Question 12
Save

A person standing on the ground at point A saw an object at point B on the ground at adistance of 600 meters. The object started flying towards him at an angle of 30° with theground. The person saw the object for the second time at point C flying at 30° angle withhim. At point C, the object changed direction and continued flying upwards. The person sawthe object for the third time when the object was directly above him. The object was flyingat a constant speed of 10 kmph.

Find the angle at which the object was flying after the person saw it for the second time. You may use additional statement(s) if required.

Statement I: After changing direction the object took 3 more minutes than it had taken before.

Statement II: After changing direction the object travelled an additional 200√3 meters.

Which of the following is the correct option?
Detailed Solution for Test: XAT Quant 2016 - Question 12

Given, AB = 600 meters. ∠ CAE =° 60. Assume D is the middle point of AB. Then AD = 300 meters.

As ∠CAB =∠CBA, CA = CB and ∠ACD is right angled triangle.

Also, AD/AC = cos30° Then, AC = 600/√3 = 200√3 = CB

From Statement I:

CE = 500 meters. Assume F is perpendicular on AE.

Then, ∠ACF = 30° and ∠ CAF = 60°. Also, CF = 300 meters.

Then, cos ∠ECF = 300/500 = 3/5. So, we can get the value of

∠ECF, the new angle.

From Statement II: CE = 500 meters = CA. Then,

∠ECF = 30°, which is different from the angle found usingStatement I.

Hence, Option D is the correct answer.

Test: XAT Quant 2016 - Question 13
Save

f is a function for which f (1) = 1 and f(x) = 2x + f(x – 1) for each natural number x ≥ 2. Find f (31)
Detailed Solution for Test: XAT Quant 2016 - Question 13
f (31) = f (30) + 2×31 = f (29) + 2(31 + 30) = f (28) + 2(31 + 30 + 29) = ... = f (2) + 2(31 + 30 + 29 + ... + 3) = 2(31 + 30 + 29 + 3 + 2) +1

= 2 × 32 × 31/2 – 1 = 991

Test: XAT Quant 2016 - Question 14
Save

Two numbers in the base system B are 2061B and 601B. The sum of these two numbers indecimal system is 432. Find the value of 1010B in decimal system.
Detailed Solution for Test: XAT Quant 2016 - Question 14
In decimal system the values are

2061B = 2B3 + 6B + 1 and 601B = 6B2 + 1

Then, 2B3 + 6B2 + 6B + 2 = 432

Or, (B + 1)3 = 216 = 63

Hence, B = 5

Then, 1010B = B3 + B = 53 + 5 = 130

Option C is the correct answer.

Test: XAT Quant 2016 - Question 15
Save

A water tank has M inlet pipes and N outlet pipes. An inlet pipe can fill the tank in 8 hourswhile an outlet pipe can empty the full tank in 12 hours. If all pipes are left open simultaneously, it takes 6 hours to fill the empty tank. What is the relationship between M and N?
Detailed Solution for Test: XAT Quant 2016 - Question 15
M inlet pipes can fill the Mth/8 part of the tank in 1 hour and N outlet pipes can empty Nth/12 part of the tank in 1 hour. If all pipes are left open, in 1 hour the tank will be filled = M/8 – N/12 = 1/6 (the tank will take 6 hours to fill).

Then, 6M – 4N = 8

M = (8 + 4N)/6

There will be infinite number of solutions (N = 1, 4, 7, 10, 13, 16 ...) but none of the above ratios are correct. For example, above equation is solvable when M = 2, N = 1 or M = 4, N = 4. But the ratios 2 : 1 (i.e. M = 2, N = 1) or 1 : 1 (M = 4, N = 4) are not correct because the solution of the above equation will not be an integer for values like M = 4, N = 2 or M = 6, N = 6.

Therefore, Option E is the correct answer.

Test: XAT Quant 2016 - Question 16
Save

Company ABC starts an educational program in collaboration with Institute XYZ. As per theagreement, ABC and XYZ will share profit in 60: 40 ratio. The initial investment of Rs. 100,000 on infrastructure is borne entirely by ABC whereas the running cost of Rs. 400 per student is borne by XYZ. If each student pays Rs. 200 for the program find the minimumnumber of students required to make the program profitable, assuming ABC wants to recover its investment in the very first year and the program has no seat limits.
Detailed Solution for Test: XAT Quant 2016 - Question 16
For program to be profitable both companies must recover costs before they can start making profits.

Since, ABC wants to recoup investment in the first year and there is no limit of number of students profits can only be shared after both companies can reach a situation of minimum profits (zero profit), which would be: 400x + 100000 = 2000x,

Left hand side represents total costs and right hand side represents revenues. Let x be minimum number of students required to reach a situation of minimum profits (in this case 0).

For the entire program, the MINIMUM students required for minimum profit is = 100000/ (2000 - 400) = 62.5 = 63.

Test: XAT Quant 2016 - Question 17
Save

For two positive integers a and b, if (a + b) (a + b) is divisible by 500, then the least possiblevalue of a × b is:
Detailed Solution for Test: XAT Quant 2016 - Question 17
500 can be written as 5 × 5 × 5 × 2 × 2. As the number is divisible by 500, (a + b) should be divisible by 10.

Then, least possible value of (a + b) = 10

Hence, least possible value of a×b = 9×1 = 9 (when a = 9 and b = 1)

Option B is the correct answer.

Test: XAT Quant 2016 - Question 18
Save

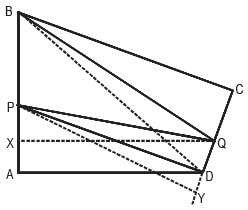
Study the figure below and answer the question:

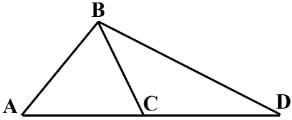
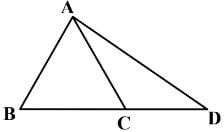
Four persons walk from Point A to Point D following different routes. The one following ABCD takes 70 minutes. Another person takes 45 minutes following ABD. The third person takes 30 minutes following route ACD. The last person takes 65 minutes following route ABCD. IF all were to walk at the same speed, how long will it take to go from point B to point C?
Detailed Solution for Test: XAT Quant 2016 - Question 18
AB + BC + CD = 70

AB + BD = 45

AC + CD = 30

AC + CB + BD = 65

Then, (AB + BC + CD) + (AC + CB + BD) = 70 +65 = 135

or, (AB + BD) + (AC + CD) + 2BC = 135

Finally, BC = 30

Option C is the correct answer.

Test: XAT Quant 2016 - Question 19
Save

a, b, c are integers, |a| ≠ |b| ≠|c| and –10 ≤ a, b, c ≤ 10. What will be the maximum possible value of [abc – (a + b + c)]?
Detailed Solution for Test: XAT Quant 2016 - Question 19
For maximum value, two of a, b, and c should be negative (all three negative will make abc negative). Hence the maximum will occur when a = –10, b = –9, and c = 8.

Then, abc = 731

Option C is the correct answer.

Test: XAT Quant 2016 - Question 20
Save

ABCD is a quadrilateral such that AD = 9 cm, BC = 13 cm and ⎿DAB = ⎿BCD = 90°. P and Qare two points on AB and CD respectively, such that DQ: BP = 1: 2 and DQ is an integer. How many values can DQ take, for which the maximum possible area of the quadrilateralPBQD is 150 sq.cm?
Detailed Solution for Test: XAT Quant 2016 - Question 20

Let’s assume DQ = x, CQ =y, AP = z. Thus, BP = 2x

Area of PBQD = Total area of the quadrilateral ABCD – (Area of triangle APD + Area of triangle BCQ)

Total area of the quadrilateral ABCD = Area of triangle ABD + Area of triangle BCD

Thus, Area of PBQD

[1/2 x (2x + z) x 9 + ½ x 13 x (x+y)] – [1/2 x 9 x z + ½ x 13 x y]

= 31x/2

According to the question, 31x/2 ≤ 150 ≤ or, x 9.68

Therefore, x can take 9 integer values. Option D is the correct answer.

Test: XAT Quant 2016 - Question 21
Save

If a, b and c are 3 consecutive integers between –10 to +10 (both inclusive), how manyinteger values are possible for the expression (a3 + b3 + c3 + 3abc)/ (a+b+c) 2?
Detailed Solution for Test: XAT Quant 2016 - Question 21
a3 + b3 + c3 – 3abc = (a + b + c)1/2 {(a – b)2 + (b – c)2 + (c –a)2}

For three consecutive integers, (x – 1), x and (x + 1), {(a – b)2 + (b – c)2 + (c – a)2} = 6, (a + b + c) = 3x and abc = x3 – x.

Then, (a3 + b3 + c3 + 3abc)/ (a+b+c) 2 = (a + b + c) 3 - 3abc + 6abc/ (a+b+c) 2

= (9x + 6x3 – 6x) / 9x2 = 1/3x + 2x/3

For the expression integer value is possible only if x = 1 and x = –1.

Possible values of a, b, c are (0, 1, 2) and (–2, –1, 0).

Hence, Option C is the correct answer.

Test: XAT Quant 2016 - Question 22
Save

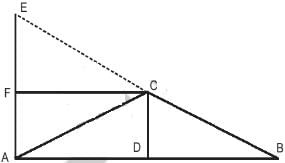
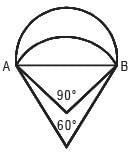
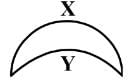
In the figure below, two circular curves create 60° and 90° angles with their respective centres. If the length of the bottom curve Y is 10π, find the length of the other curve.
Detailed Solution for Test: XAT Quant 2016 - Question 22

Here, curve X creates 90° and curve Y creates 60°.

Take, radius of curve X = R1 and radius of curve Y = R2

For curve Y, 2πR2= 10π× 6.

Then, R2 = 30 = line AB.

Then, R1 = 15√2 Finally, length of curve X = 2πR2/ 4 = 15π / √2

Option A is the correct answer.

Test: XAT Quant 2016 - Question 23
Save

Study the graph below and answer the questions that follow:

This graph depicts the last eight years’ annual salaries (in Rs. lacs.) offered to student during campus placement. Every year 100 students go through placement process. However, at least one of them fails to get placed. The salaries of all unplaced students are marked zero and represented in the graph.

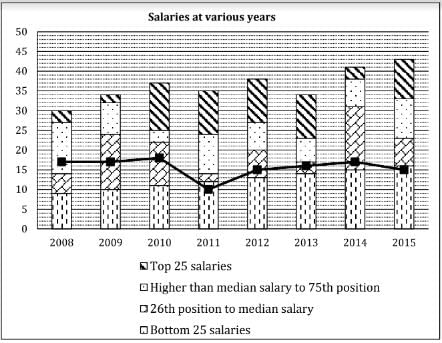
The bold line in the graph presents Mean salaries at various years.

Q. In which year were a maximum number of students offered salaries between Rs. 20 to Rs. 30 lacs(both inclusive)?
Detailed Solution for Test: XAT Quant 2016 - Question 23
At least 25% of the students definitely got salaries between 20-30 lacs. in years 2008, 2010 and 2012. However, the graphs do not tell the actual salaries. Hence, no comment can be made on the number of students within a particular salary range.

Option E is the correct answer.

Test: XAT Quant 2016 - Question 24
Save

Study the graph below and answer the questions that follow:

This graph depicts the last eight years’ annual salaries (in Rs. lacs.) offered to student during campus placement. Every year 100 students go through placement process. However, at least one of them fails to get placed. The salaries of all unplaced students are marked zero and represented in the graph.

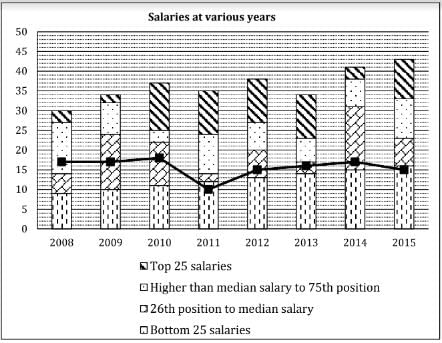
The bold line in the graph presents Mean salaries at various years.

Q. Identify the years in which the annual median salary is higher by at least 60% than the average salary of the preceding year?
Detailed Solution for Test: XAT Quant 2016 - Question 24
It is clear from the above table that in 2012 and 2014, the median of existing year is higher than 1.6 times mean of previous years. Hence the correct answer is: 2012 and 2014.

Option B is the correct answer.

Test: XAT Quant 2016 - Question 25
Save

Study the graph below and answer the questions that follow:

This graph depicts the last eight years’ annual salaries (in Rs. lacs.) offered to student during campus placement. Every year 100 students go through placement process. However, at least one of them fails to get placed. The salaries of all unplaced students are marked zero and represented in the graph.

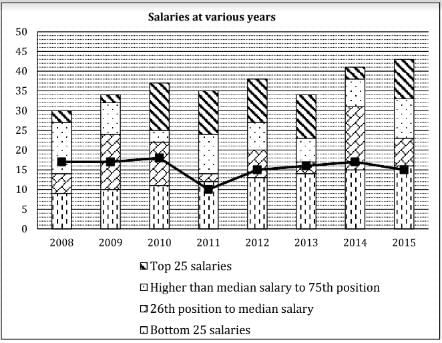
The bold line in the graph presents Mean salaries at various years.

Q. Identify the number of years in which the difference between the average salaries of the top 25% and the bottom 25% is more than Rs. 20 lacs:
Detailed Solution for Test: XAT Quant 2016 - Question 25
The question was withdrawn because of insufficient data.

Test: XAT Quant 2016 - Question 26
Save

Study the graph below and answer the questions that follow:

This graph depicts the last eight years’ annual salaries (in Rs. lacs.) offered to student during campus placement. Every year 100 students go through placement process. However, at least one of them fails to get placed. The salaries of all unplaced students are marked zero and represented in the graph.

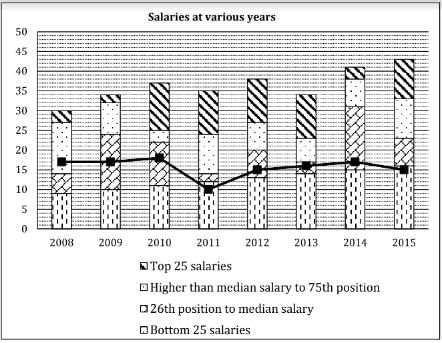
The bold line in the graph presents Mean salaries at various years.

Q. If the average salary is computed excluding students with no offers, in how many years will the new average salary be greater than the existing median salary? Refer the table below for number of students without offers.
Detailed Solution for Test: XAT Quant 2016 - Question 26
The answer can be found based on the table below

New Average Salary = (Old Average Salary) x 100/(100 - students without jobs)

Based on the additional information, the new average would be higher than the graph average of all the years. During two years old averages were already higher than median (2008 and 2013). With 20 students taken out, the new average salary for 2010 would jump to 23.4, which is clearly higher than median salary of 22. Similarly, the value of 2013 would jump further by at least one hence making new average definitely higher than existing median. In 2008, even the existing mean is visibly higher than median. Hence, the three 3 years are: (2008, 2010, and 2013).Option A is the correct answer.

Test: XAT Quant 2016 - Question 27
Save

Study the data given in the table below and answer the question that follow:

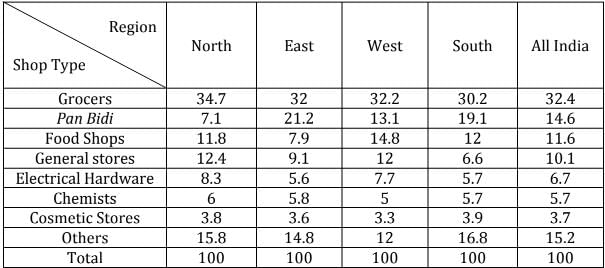
All figures are in percentage

Based on survey of ‘shop types’ Kamath categorized Indian states into four geographical regions as shown in the table above. His boss felt that the categorization was inadequate since important labels were missing. Kamath argued that no further labels are required to interpret the data.

Q. A consultant observing the data made the following two inferences:

Inference I: The number of Grocers per-thousand-population is the highest in North India.

Inference II: The number of Cosmetic per-thousand-population is the highest in South India.

Which of following options is DEFINITELY correct?
Detailed Solution for Test: XAT Quant 2016 - Question 27
Since no data is given on population as well as distribution of states into four zones, it is not possible to calculate per thousand numbers.

Test: XAT Quant 2016 - Question 28
Save

Study the data given in the table below and answer the question that follow:

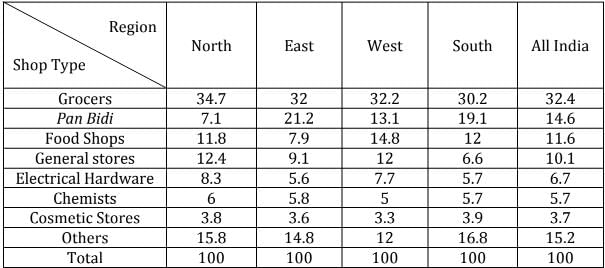
All figures are in percentage

Based on survey of ‘shop types’ Kamath categorized Indian states into four geographical regions as shown in the table above. His boss felt that the categorization was inadequate since important labels were missing. Kamath argued that no further labels are required to interpret the data.

Q. The average size of Food Shops in East India was twice that of Food Shops in West India.Which of the following cannot be inferred from the above data?
Detailed Solution for Test: XAT Quant 2016 - Question 28
Option A and B can be one of the possible conclusions (there are many others) as average size of food shops in East India is higher.

Option D also is a possible conclusion as percentage given is 14.8, which can be converted into 740 (14.8 × 50) or a similar multiple of 14.8

Similarly Option E can also be a possible conclusion since 240 is a multiple of 12 (12 × 20 = 240).

To conclude option C, we require additional data about number of customers and hence cannot be concluded on the basis of data above.

Test: XAT Quant 2016 - Question 29
Save

Study the data given in the table below and answer the question that follow:

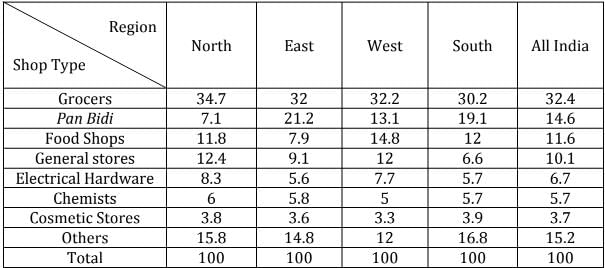
All figures are in percentage

Based on survey of ‘shop types’ Kamath categorized Indian states into four geographical regions as shown in the table above. His boss felt that the categorization was inadequate since important labels were missing. Kamath argued that no further labels are required to interpret the data.

Bala collected the same data five years after Kamath, using the same categorization. His data is presented below:

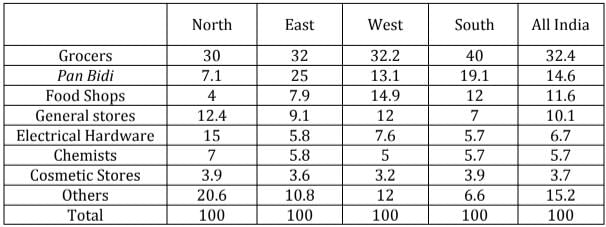Which of the following statements can DEFINITELY be concluded?
Detailed Solution for Test: XAT Quant 2016 - Question 29
Option A cannot be concluded as there is no mention of gross number in the two time periods.

Using a similar rationale, Option B, C and D cannot be concluded.

Option E can be concluded as population for Pan Bidi shops and Grocers is the same. These are percentages of the same base (total number of shops in East India)

Information about Test: XAT Quant 2016 Page
In this test you can find the Exam questions for Test: XAT Quant 2016 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: XAT Quant 2016, EduRev gives you an ample number of Online tests for practice

Top Courses for CAT

Download as PDF

Top Courses for CAT

Important Questions for XAT Quant 2016

Find all the important questions for XAT Quant 2016 at EduRev.Get fully prepared for XAT Quant 2016 with EduRev's comprehensive question bank and test resources. Our platform offers a diverse range of question papers covering various topics within the XAT Quant 2016 syllabus. Whether you need to review specific subjects or assess your overall readiness, EduRev has you covered. The questions are designed to challenge you and help you gain confidence in tackling the actual exam. Maximize your chances of success by utilizing EduRev's extensive collection of XAT Quant 2016 resources.

XAT Quant 2016 MCQs with Answers

Prepare for the XAT Quant 2016 within the CAT exam with comprehensive MCQs and answers at EduRev. Our platform offers a wide range of practice papers, question papers, and mock tests to familiarize you with the exam pattern and syllabus. Access the best books, study materials, and notes curated by toppers to enhance your preparation. Stay updated with the exam date and receive expert preparation tips and paper analysis. Visit EduRev's official website today and access a wealth of videos and coaching resources to excel in your exam.

Online Tests for XAT Quant 2016

Practice with a wide array of question papers that follow the exam pattern and syllabus. Our platform offers a user-friendly interface, allowing you to track your progress and identify areas for improvement. Access detailed solutions and explanations for each test to enhance your understanding of concepts. With EduRev's Online Tests, you can build confidence, boost your performance, and ace XAT Quant 2016 with ease. Join thousands of successful students who have benefited from our trusted online resources.
© EduRev
Education Revolution
Follow Us
Signup to see your scores go up within 7 days!
Access 1000+ FREE Docs, Videos and Tests
Takes less than 10 seconds to signup