Explore Exams
Login Signup
Sign in Open App
CBSE Class 9  >  Class 9 Test  >  Mathematics (Maths)   >  Test: Lines And Angles- 2 - Class 9 MCQ

Lines And Angles- 2 - Free MCQ Practice Test with solutions, Class 9 Maths


MCQ Practice Test & Solutions: Test: Lines And Angles- 2 (15 Questions)

You can prepare effectively for Class 9 Mathematics (Maths) Class 9 with this dedicated MCQ Practice Test (available with solutions) on the important topic of "Test: Lines And Angles- 2". These 15 questions have been designed by the experts with the latest curriculum of Class 9 2026, to help you master the concept.

Test Highlights:

  • - Format: Multiple Choice Questions (MCQ)
  • - Duration: 25 minutes
  • - Number of Questions: 15

Sign up on EduRev for free to attempt this test and track your preparation progress.

Test: Lines And Angles- 2 - Question 1
Save

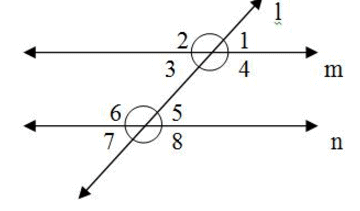
In the adjoining figure, if m ║ n, then ∠4 + ∠7 is equal to –


 

 

Detailed Solution: Question 1

As lines m and n are parallel and line l is the transversal line

∠4 = ∠8 [ corresponding angles are equal]
∠7 + ∠8 = 180 [replacinig ∠8 by ∠4 ]
∠7 + ∠4 = 180

Test: Lines And Angles- 2 - Question 2
Save

If two angles are supplementary and the larger is 200 less then three times the smaller, then the angles are :-

Detailed Solution: Question 2

supplementary = 180 degrees
larger = 3x - 20
smaller = x 
3x - 20 + x = 180
4x - 20 = 180
4x = 180 + 20
4x = 200
4x/4 = 200/4
x = 50 
smaller = 50 degrees
larger = 3(50) - 20 = 150 - 20 = 130 degrees

Clear all your doubts with EduRev
Test: Lines And Angles- 2 - Question 3
Save

Two planes intersect each other to form a :

Detailed Solution: Question 3

Two non-parallel planes in space form a line upon intersection, as long as each plane is unique. Three non-parallel planes intersect at a point. The best and simplest example is to fold a piece of paper. Since the folded paper now represents two distinct planes, the crease itself is the intersection point, forming a line.

Test: Lines And Angles- 2 - Question 4
Save

In a right-angled triangle where angle A = 90° and AB = AC. What are the values of angle B?

Detailed Solution: Question 4

∵ In ∆ABC,
AB = AC
∴ ∠B = ∠C    ...(1)
| Angles opposite to equal sides of a triangle are equal
In ∆ABC,
∠A + ∠B + ∠C = 180°
| Sum of all the angles of a triangle is 180°
⇒ 90° + ∠B + ∠C = 180°
| ∵ ∠A = 90° (given)
⇒    ∠B + ∠C = 90°    ...(2)
From (1) and (2), we get
∠B = ∠C = 45°.

Test: Lines And Angles- 2 - Question 5
Save

An exterior angle of a triangle is 800 and the interior opposite angles are in the ratio 1 : 3. Measure of each interior opposite angle is :

Detailed Solution: Question 5

Let the interior angles be x and 3x 

We know that exterior angle of triangle is equal to sum of interior opposite angles.

⇒ x+3x=80∘

⇒ 4x=80∘

⇒ x=20∘

So the angles are 

x=20∘

3x=3×20∘

= 60∘

Test: Lines And Angles- 2 - Question 6
Save

In figure, AB and CD are parallel to each other. The value of x is :

Detailed Solution: Question 6

Step-by-Step Explanation:

  1. Draw Parallel Line: Draw a line EF parallel to AB and CD through the point E.

  2. Identify Angles:

    • ∠BXE = 120° is given.
    • ∠XED = 140° is also given.
    • Let ∠EFX be the angle formed by the line EF and the transversal EX.

  3. Corresponding Angles: Since EF || AB and AB || CD, the angles formed by the transversal EX with these parallel lines are corresponding angles. Therefore:

    • ∠BXE = ∠FEX = 120° (corresponding angles).
    • ∠XED = ∠EFX = 140° (corresponding angles).

  4. Sum of Angles Around Point E: The angles around point E add up to 360°. We have:
    ∠FEX + ∠XED + ∠EFX + x = 360°Substituting the values:
    120° + 140° + x = 360°

  5. Calculate x: Solve for x:
    260° + x = 360°
    x = 360° − 260°
    x = 100°
    Thus, by introducing the parallel line EF and using the properties of corresponding angles, we conclude that the value of x is 100°.

Test: Lines And Angles- 2 - Question 7
Save

In the adjoining figure, m ║ n. If ∠a : ∠b = 2 : 3, then the measure of ∠h is –

Detailed Solution: Question 7

A+b =180(linear pair)

2x+3x=180

5x=180

x=180/5

x=36

a=2x=2*36=72

b=3x=3*36=108

b=d (vertical opposite angles are equal)

d=f (alternative interior angles are equal)

f=h (vertically opposite angles are equal)

So, h= 108

Test: Lines And Angles- 2 - Question 8
Save

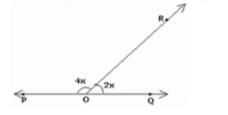
In the figure, POQ is a line, ∠POR = 4x and ∠QOR = 2x. Find the value of x.

Detailed Solution: Question 8

∠POR + ∠QOR = 180° (Linear pair axiom)
But:
∠POR = 4x
∠QOR = 2x
Therefore:
∴ 4x + 2x = 180°
6x = 180°
x = 30°
Thus, the value of x = 30°.

Test: Lines And Angles- 2 - Question 9
Save

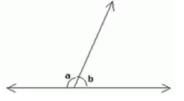
In the figure, a is greater than b by one third of a right angle. Find the values of a and b.

Detailed Solution: Question 9

Given that a > b by one third of a right angle:
a = b + (1/3) × 90° → a = b + 30°
But a + b = 180° (Linear pair axiom):
b + 30° + b = 180°
2b + 30° = 180°
2b = 180° - 30°
2b = 150°
Thus:
b = 75°
a = 180° - 75° = 105°
Therefore, the measure of a is 105° and b is 75°.

Test: Lines And Angles- 2 - Question 10
Save

In the figure, if ∠AOC + ∠BOD = 70°, find ∠COD
​​​​​​​

Detailed Solution: Question 10

∠AOC + ∠BOD = 70° (Given) ................... (i)
∠AOC + ∠COD + ∠BOD = 180° (Linear pair axiom)
∴ ∠COD = 180° - (∠AOC + ∠BOD)
= 180° - 70° [From (i)]
= 110°

∴ The measure of ∠COD = 110°.

Test: Lines And Angles- 2 - Question 11
Save

In two interior angles on the same side of a transversal intersecting two parallel lines are in the ratio 5 : 4, then the smaller of the two angles is :

Detailed Solution: Question 11

Let the two interior angles on the same side of the transversal be 5x and 4x. The sum of these angles is 180°, as they are co-interior angles.

5x + 4x = 180°
9x = 180°
x = 180° / 9 = 20°

The smaller angle is 4x = 4 × 20 = 80°.

Test: Lines And Angles- 2 - Question 12
Save

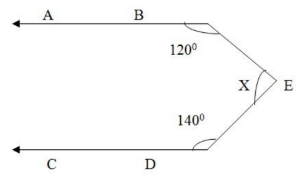
In the adjoining figure, AB ║ CD and AB ║ EF. The value of x is :-

Detailed Solution: Question 12

Given, AB ║ CD and AB ║ EF

so CD || EF

which means ∠ECD + ∠CEF = 1800 (corresponding angles)

∠ECD = 180 - 150 = 300

since AB || CD so 

∠ABC= ∠BCD (alternate interior angles)

∠ABC = 30 + ∠ECD = 30 + 30 = 600

Test: Lines And Angles- 2 - Question 13
Save

Sum of all angles around a main point equals to

Detailed Solution: Question 13

The sum of all angles around a main point equals 360 degree , as it represents a full rotation around the point.

Final Answer: A: 360°.

Test: Lines And Angles- 2 - Question 14
Save

What is the supplement of 105°      

Detailed Solution: Question 14

The supplement of an angle is calculated as:
Supplement = 180° − Given angle

For 105°:
Supplement = 180° − 105° = 75°

Test: Lines And Angles- 2 - Question 15
Save

Find the angle if six times of its complement 12° less than twice of its supplement?

Detailed Solution: Question 15

Let the angle be x. The complement of x is 90° - x, and the supplement of x is 180° - x.

From the condition, "Six times its complement is 12° less than twice its supplement," we have:

6(90 - x) = 2(180 - x) - 12

Expanding both sides:
540 - 6x = 360 - 2x - 12

Simplify:
540 - 6x = 348 - 2x

Rearranging terms:
540 - 348 = 6x - 2x
192 = 4x

Solving for x:
x = 192 / 4 = 48°

The angle is 48°.

40 videos|468 docs|55 tests
Join Course
Information about Test: Lines And Angles- 2 Page
In this test you can find the Exam questions for Test: Lines And Angles- 2 solved & explained in the simplest way possible. Besides giving Questions and answers for Test: Lines And Angles- 2, EduRev gives you an ample number of Online tests for practice
40 videos|468 docs|55 tests
Join Course
Download as PDF

About this Class 9 Practice Test

This test contains 15 MCQs on Lines And Angles- 2 with detailed solutions for each question. It is part of the Mathematics (Maths) Class 9 course on EduRev, designed to align with the current Class 9 syllabus. Each solution explains not just the correct answer but also gives you an understanding of the topic — not just to attempt the question but also help you prepare for the exam with practice.

Explore more Class 9 Study Resources

If you found this Lines And Angles- 2 MCQ Test helpful, you can further enhance your Class 9 preparation with in-depth courses on EduRev, for all the subjects and topics available in Mathematics (Maths) Class 9 course. The course offers:
  • Video Lectures: covering all the topics of Mathematics (Maths) Class 9
  • Notes for Revision: offering revision notes, important questions, summaries, flashcards, cheatsheets & more for Class 9 preparation
  • MCQ Test: variety of questions as per the pattern of Class 9 exam
Signup to see your scores go up
within 7 days!
Takes less than 10 seconds to signup