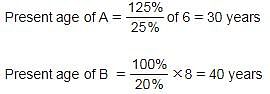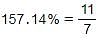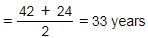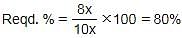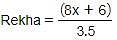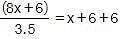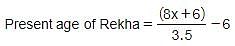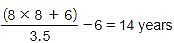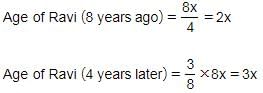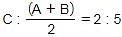MCQ: Problem Based on Ages - 3 - SSC CGL MCQ
15 Questions MCQ Test - MCQ: Problem Based on Ages - 3
8 years ago, the ratio of the age of P to that of Q was 6 : 7 which is equal to the ratio of the present age of A to that of B. 6 years later, A will be 1.2 times as old P and B will be 13 years older than Q. What is the present age (in years) of Q?
In the last 6 years, the age of A increased by 25% and in the next 8 years age of B will increase by 20%. 15 years from now, the ratio of the age of A to that of B, will be the same as the ratio of the age of C to that of D. If the difference between the age of C and D is 6 years, what is the present age (in years) of D?
The difference between the square of the present age (in years) of a mother and her daughter is 875. 5 years later, the ratio of their ages will be 7 : 2. What is the present age (in years) of the mother?
The average age of a family of four members (father, mother, boy and girl) is 28 years and when it becomes 32 years the girl will be 10 years old. When the girl was born the father was four times as old as the boy. If the father is 2 years older than the wife, what is the present age (in years) of the boy?
The age gap between Amit and Kiran is 4 years and that between Kiran and Rashi is also 4 years. The product of the age of Amit and Rashi is 240 years. If all of them have different ages, which of the following can be the ratio of the age of Amit to that of Kiran?
The average ages of A and B is 157.14% of the average age of C and D. 10 years ago, the ages of A, B, C and D were ‘X + 12’ years, ‘X + 16’ years, ‘X + 2’ years and ‘X − 6’ years, respectively. What is the present average age (in years) of A and D?
A family has six members and 12 years ago their average age was 50 years. 4 years ago, they married their only daughter and this year they married their only son so this year family again has six members including daughter-in-law and excluding the daughter such that the sum of the age of all family members is 382 years. What is the age difference between the daughter and the daughter-in-law?
Firdauz, Ali, Sarfaraz, Sameer and Faiz are five brothers, youngest to eldest in the same order. The age gap between the five brothers is equal such that the ratio of the age of the youngest to the eldest brother is 5 : 9. Ali will be ‘z’ years old when the ratio of the age of Firdauz to the age of Faiz becomes 9 : 13. Present age of Sameer is what percent of ‘z’?
Rohan is 8 times as old as Jatin and 6 years later he will be 3.5 times as old as Rekha. Rekha celebrated her 8th birthday 1 year after Jatin celebrated his first birthday. What is the present age (in years) of Rekha?
The ratio of the age of A to that of B ‘x’ years ago was 2 : 3 and this ratio will be 7 : 8 after ‘3x’ years. Presently B is 17/13 times as old as A. 2 years after A was born, B was five times as old as A. What is the value of ‘x’?
The sum of the ages of Chetan and Ravi is equal to the age of their grandmother. 8 years ago, Ravi was 1/4 times as old as his grandmother’s present age and 4 years later he will be 3/8 times as old as her present age. What will be the ratio of the age of Ravi to that of Chetan after 8 years?
Sneha and Garima met at a Coffee shop where Garima told Sneha, “10 years ago I was 2/3rd of your age and 14 years later you will be 7/6th of my age.” If both of them completed their high school at the age of 16, what was the age (in years) of Garima when Sneha completed her high school?
The average age of Naira, Ada and Sehar is 35 years. When Ada becomes as old as the present age of Naira, Naira’s age will be 11/6 times the present age of Sehar. 12 years ago Sehar was as old as the present age of Ada. What is the age (in years) of the oldest among them?
15 years from now A will be thrice as old as B was 5 years ago. C is 2/5 times as old as the present average age of A and B. If the present average age of A, B and C is 20 years, what was the age (in years) of A when C was born?
A couple got married when both of them were 20 years old. After ‘x’ years a son was born and ‘y’ years later they had twin daughters. Presently the total age of the family is 116 years and the youngest member is 10 years old. If the ratio of the age of the mother and the son is 5 : 2, what is the present age (in years) of the son?