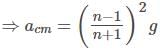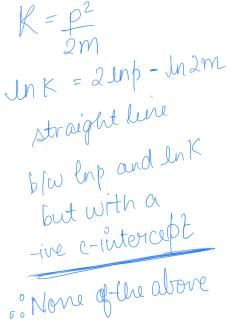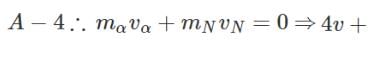Test: Centre of Mass - 3 - NEET MCQ
30 Questions MCQ Test Topic-wise MCQ Tests for NEET - Test: Centre of Mass - 3
Two billiard balls undergo a head-on collision. Ball 1 is twice as heavy as ball 2. Initially, ball 1 moves with a speed v towards ball 2 which is at rest. Immediately after the collision, ball 1 travels at a speed of v/ 3 in the same direction. What type of collision has occured ?
A ball of 0.1kg strikes a wall at right angle with a speed of 6 m/s and rebounds along its original path at 4 m/s. The change in momentum in Newton- sec is-
Before a rubber ball bounces off from the floor the ball is in contact with the floor for a fraction of second. Which of the following statements are correct-
A metal ball hits a wall and does not rebound whereas a rubber ball of the same mass on hitting the wall the same velocity rebounds back. It can be concluded that -
An object of mass 5 kg and speed 10 ms-1 explodes into two pieces of equal mass. One piece comes to rest. The kinetic energy added to the system during the explosion is-
A ball is dropped from height 5m. The time after which ball stops rebounding if coefficient of restitution between ball and ground e = 1/2, is
A ball is dropped from a height h. As is bounces off the floor, its speed is 80 percent of what it was just before it hit the floor. The ball will then rise to a height of most nearly
Two billiard balls undergo a head-on collision. Ball 1 is twice as heavy as ball 2. Initially, ball 1 moves with a speed v towards ball 2 which is at rest. Immediately after the collision, ball 1 travels at a speed of v/ 3 in the same direction. What type of collision has occured ?
A truck moving on horizontal road east with velocity 20ms-1 collides elastically with a light ball moving with velocity 25 ms-1 along west. The velocity of the ball just after collision
Two balls A and B having masses 1 kg and 2 kg, moving with speeds 21 m/s and 4 m/s respectively in opposite direction, collide head on. After collision A moves with a speed of 1 m/s in the same direction, then the coefficient of restitution is
A ball hits the floor and rebounds after an inelastic collision . In this case-
A ball strikes a smooth horizontal ground at an angle of 45° with the vertical. What cannot be the possible angle of its velocity with the vertical after the collision. (Assume e <=1).
A boy hits a baseball with a bat and imparts an impulse J to the ball. The boy hits the ball again with the same force, except that the ball and the bat are in contact for twice the amount of time as in the first hit. The new impulse equals.
A system of N particles is free from any external forces
Which of the following is true for the magnitude of the total momentum of the system ?
An isolated rail car of mass M is moving along a straight, frictionless track at an initial speed v0 . The car is passing under a bridge when a crate filled with N bowling balls, each of mass m, is dropped from the bridge into the bed of the rail car. The crate splits open and the bowling balls bounce around inside the rail car, but none of them fall out.
Is the momentum of the rail car + bowling balls system conserved in this collision ?
Two balls are thrown in air. The acceleration of the centre of mass of the two balls while in air (neglect air resistance)
A man of mass M stands at one end of a plank of length L which lies at rest on a frictionless surface. The man walks to other end of the plank. If the mass of the plank is , then the distance that the man moves relative to ground is :
A uniform square plate ABCD has a mass of 10kg. If two point masses of 3 kg each are placed at the corners C and D as shown in the adjoining figure, then the centre of mass shifts to the point which is lie on-
A particle of mass 3m is projected from the ground at some angle with horizontal. The horizontal range is R. At the highest point of its path it breaks into two pieces m and 2m. The smaller mass comes to rest and larger mass finally falls at a distance x from the point of projection where x is equal to
Two particles having mass ratio n : 1 are interconnected by a light inextensible string that passes over a smooth pulley. If the system is released, then the acceleration of the centre of mass of the system is :
Which of the following graphs represents the graphical relation between momentum (p) and kinetic energy (K) for a body in motion ?
A bomb initially at rest explodes by it self into three equal mass fragments. The velocities of two fragments are ( 3 + 2
) m/s and (_
_ 4
) m/s. The velocity of the third fragment is (in m/s)-
A stone of mass m1 moving with a uniform speed v suddenly explodes on its own into two fragments. If the fragment of mass m2 is at rest, the speed of the other fragment is-
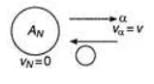
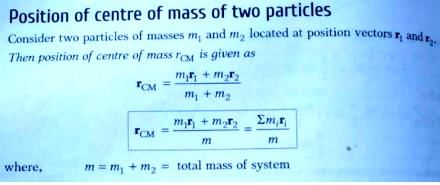
A nucleus of mass number A originally at rest emits a-particle with speed v. The recoil speed of daughter nucleus is :
A super-ball is to bounce elastically back and forth between two rigid walls at a distance d from each other. Neglecting gravity and assuming the velocity of super-ball to be v0 horizontally, the average force being exerted by the super-ball on each wall is :
A force exerts an impulse I on a particle changing its speed from u to 2u. The applied force and the initial velocity are oppositely directed along the same line. The work done by the force is
|
9 docs|1259 tests
|





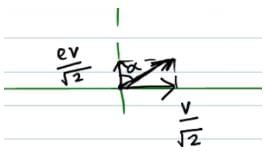
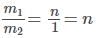
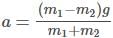
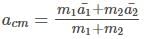
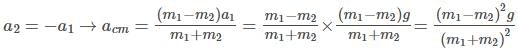
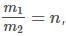 diving by m2 and simplifying
diving by m2 and simplifying