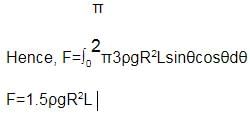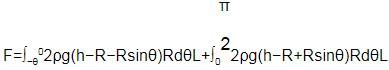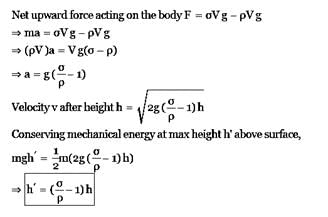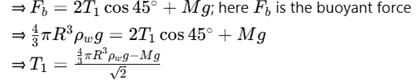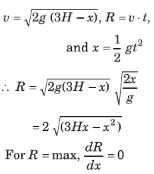Test: Fluid Mechanics - 2 - NEET MCQ
25 Questions MCQ Test - Test: Fluid Mechanics - 2
Some liquid is filled in a cylindrical vessel of radius R. Let F1 be the force applied by the liquid on the bottom of the cylinder. Now the same liquid is poured into a vessel of uniform square cross-section of side R. Let F2 be the force applied by the liquid on the bottom of this new vessel. Then :
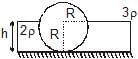
In the figure shown, the heavy cylinder (radius R) resting on a smooth surface separates two liquids of densities 2r and 3r. The height `h' for the equilibrium of cylinder must be
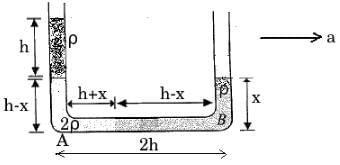
The vertical limbs of a U shaped tube are filled with a liquid of density r upto a height h on each side. The horizontal portion of the U tube having length 2h contains a liquid of density 2r. The U tube is moved horizontally with an accelerator g/2 parallel to the horizontal arm. The difference in heights in liquid levels in the two vertical limbs, at steady state will be
An open cubical tank was initially fully filled with water. When the tank was accelerated on a horizontal plane along one of its side it was found that one third of volume of water spilled out. The acceleration was
A cone of radius R and height H, is hanging inside a liquid of density r by means of a string as shown in the figure. The force, due to the liquid acting on the slant surface of the cone is (neglect atmospheric pressure)
A heavy hollow cone of radius R and height h is placed on a horizontal table surface, with its flat base on the table. The whole volume inside the cone is filled with water of density r. The circular rim of the cone's base has a watertight seal with the table's surface and the top apex of the cone has a small hole. Neglecting atmospheric pressure find the total upward force exerted by water on the cone is
A slender homogeneous rod of length 2L floats partly immersed in water, being supported by a string fastened to one of its ends, as shown. The specific gravity of the rod is 0.75. The length of rod that extends out of water is
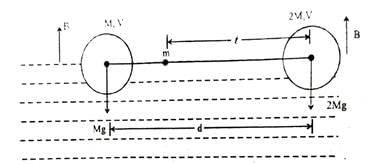
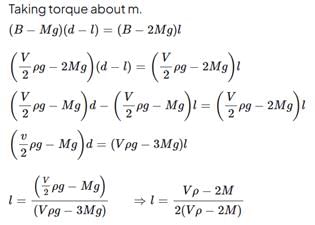
A dumbbell is placed in water of density r. It is observed that by attaching a mass m to the rod, the dumbbell floats with the rod horizontal on the surface of water and each sphere exactly half submerged as shown in the figure. The volume of the mass m is negligible. The value of length l is
A container of large surface area is filled with liquid of density r. A cubical block of side edge a and mass M is floating in it with four-fifth of its volume submerged. If a coin of mass m is placed gently on the top surface of the block is just submerged. M is
A cork of density 0.5 gcm-3 floats on a calm swimming pool. The fraction of the cork's volume which is under water is
Two cyllinders of same cross-section and length L but made of two material of densities d1and d2 are cemented together to form a cylinder of length 2L. The combination floats in a liquid of density d with a length L/2 above the surface of the liquid. If d1> d2 then :
A small wooden ball of density ρ is immersed in water of density 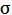 to depth h and then released. The height h' above the surface of water up to which the ball will jump out of water is
to depth h and then released. The height h' above the surface of water up to which the ball will jump out of water is
A hollow sphere of mass M and radius r is immersed in a tank of water (density rw). The sphere would float if it were set free. The sphere is tied to the bottom of the tank by two wires which makes angle 45º with the horizontal as shown in the figure. The tension T1 in the wire is :
A body having volume V and density r is attached to the bottom of a container as shown. Density of the liquid is d(>r). Container has a constant upward acceleration a. Tension in the string is
A hollow cone floats with its axis vertical upto one-third of its height in a liquid of relative density 0.8 and with its vertex submerged. When another liquid of relative density r is filled in it upto one-third of its height, the cone floats upto half its vertical height. The height of the cone is 0.10 m and radius of the circular base is 0.05 m. The specific gravity r is given by
There is a metal cube inside a block of ice which is floating on the surface of water. The ice melts completely and metal falls in the water. Water level in the
container
A uniform solid cylinder of density 0.8 g/cm3 floats in equilibrium in a combination of two non-mixing liquid A and B with its axis vertical. The densities of liquid A and B are 0.7 g/cm3 and 1.2 gm/cm3. The height of liquid A is hA = 1.2 cm and the length of the part of cylinder immersed in liquid B is hB = 0.8 cm. Then the length of the cylinder in air is
A cylindrical tank of height 1 m and cross section area A = 4000 cm2 is initially empty when it is kept under a tap of cross sectional area 1 cm2. Water starts flowing from the tap at t = 0, with a speed = 2 m/s. There is a small hole in the base of the tank of cross-sectional area 0.5 cm2. The variation of height of water in tank (in meters) with time t is best depicted by
A cubical box of wine has a small spout located in one of the bottom corners. When the box is full and placed on a level surface, opening the spout results in a flow of wine with a initial speed of v0 (see figure). When the box is half empty, someone tilts it at 45º so that the spout is at the lowest point (see figure). When the spout is opened the wine will flow out with a speed of
A vertical cylindrical container of base area A and upper cross-section area A1 making an angle 30° with the horizontal is placed in an open rainy field as shown near another cylindrical container having same base area A. The ratio of rates of collection of water in the two containers will be.
large tank is filled with water to a height H. A small hole is made at the base of the tank. It takes T1 time to decrease the height of water to H/h, (h > 1) and it takes T2 time to take out the rest of water. If T1 = T2, then the value of h is :
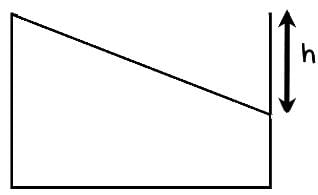
Water flows through a frictionless duct with a cross-section varying as shown in figure. Pressure p at points along the axis is represented by
A cylindrical vessel filled with water upto the height H becomes empty in time t0 due to a small hole at the bottom of the vessel. If water is filled to a height 4H it will flow out in time
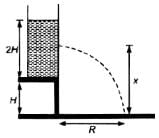
A tank is filled up to a height 2H with a liquid and is placedon a platform of height H from the ground. The distance x from the ground where a small hole is punched to get the maximum range R is :
A large tank is filled with water (density = 103 kg/m3). A small hole is made at a depth 10m below water surface. The range of water issuing out of the hole is Ron ground. What extra pressure must be applied on the water surface so that the range becomes 2R (take 1 atm = 105 Pa and g = 10 m/s2) :